Important Solved Questions for CAT: Number Systems | Quantitative Aptitude (Quant) PDF Download
Q1: For a 4-digit number, the sum of its digits in the thousands, hundreds and tens places is 14, the sum of its digits in the hundreds, tens and units places is 15, and the tens place digit is 4 more than the units place digit. Then the highest possible 4-digit number satisfying the above conditions is
Sol: Given the 4 digit number:
Considering the number in thousands digit is a number in the hundredth digit is b, number in tens digit is c, number in the units digit is d.
Let the number be abcd.
Given that a + b + c = 14. (1)
b + c + d = 15. (2)
c = d + 4. (3).
In order to find the maximum number which satisfies the condition, we need to have abcd such that a is maximum which is the digit in thousands place in order to maximize the value of the number. b, c, and d are less than 9 each as they are single-digit numbers.
Substituting (3) in (2) we have b+d+4+d = 15, b+2*d = 11. (4)
Subtracting (2) and (1) : (2) – (1) = d = a+1. (5)
Since c cannot be greater than 9 considering c to be the maximum value 9 the value of d is 5.
If d = 5, using d = a+1, a = 4.
Hence the maximum value of a = 4 when c = 9, d = 5.
Substituting b+2*d = 11. b = 1.
The highest four-digit number satisfying the condition is 4195
Q2: For all possible integers n satisfying 2.25 ≤ 2 + 2n+2 ≤ 202, then the number of integer values of 3 + 3n+1 is:
Sol: 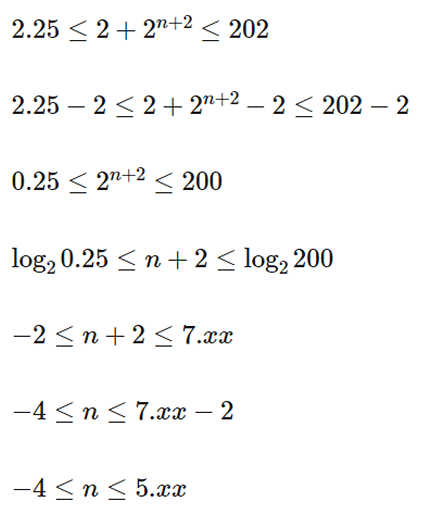
Possible integers = -4, -3, -2, -1, 0, 1, 2, 3, 4, 5
If we see the second expression that is provided, i.e
3 + 3n +1, it can be implied that n should be at least -1 for this expression to be an integer.
So, n = -1, 0, 1, 2, 3, 4, 5.
Hence, there are a total of 7 values.
Q3: How many 4-digit numbers, each greater than 1000 and each having all four digits distinct, are there with 7 coming before 3?
Sol: Here there are two cases possible
Case 1: When 7 is at the left extreme
In that case 3 can occupy any of the three remaining places and the remaining two places can be taken by (0,1,2,4,5,6,8,9)
So total ways 3(8)(7)= 168
Case 2: When 7 is not at the extremes
Here there are 3 cases possible. And the remaining two places can be filled in 7(7) ways.(Remember 0 can’t come on the extreme left)
Hence in total 3(7)(7)=147 ways
Total ways 168+147=315 ways
Q4: How many pairs(a, b) of positive integers are there such that a ≤ b and ab = 42017?
(a) 2018
(b) 2019
(c) 2017
(d) 2020
Ans: (a)
Sol: ab = 42017 = 24034
The total number of factors = 4035.
out of these 4035 factors, we can choose two numbers a, b such that a < b in [4035/2] = 2017.
And since the given number is a perfect square we have one set of two equal factors.
∴ many pairs(a, b) of positive integers are there such that a ≤ b and ab = 42017 = 2018.
Q5: How many of the integers 1, 2, … , 120, are divisible by none of 2, 5 and 7?
(a) 42
(b) 41
(c) 40
(d) 43
Ans: (b)
Sol: The number of multiples of 2 between 1 and 120 = 60
The number of multiples of 5 between 1 and 120 which are not multiples of 2 = 12
The number of multiples of 7 between 1 and 120 which are not multiples of 2 and 5 = 7
Hence, number of the integers 1, 2, … , 120, are divisible by none of 2, 5 and 7 = 120 – 60 – 12 – 7 = 41
Q6: Let N, x and y be positive integers such that N = x + y, 2 < x < 10 and 14 < y < 23. If N > 25, then how many distinct values are possible for N?
Sol: Possible values of x = 3,4,5,6,7,8,9
When x = 3, there is no possible value of y
When x = 4, the possible values of y = 22
When x = 5, the possible values of y=21,22
When x = 6, the possible values of y = 20.21,22
When x = 7, the possible values of y = 19,20,21,22
When x = 8, the possible values of y=18,19,20,21,22
When x = 9, the possible values of y=17,18,19,20,21,22
The unique values of N = 26,27,28,29,30,31
Q7: How many integers in the set {100, 101, 102, …, 999} have at least one digit repeated?
Sol: Total number of numbers from 100 to 999 = 900
The number of three digits numbers with unique digits:
_ _ _
The hundredth’s place can be filled in 9 ways ( Number 0 cannot be selected)
Ten’s place can be filled in 9 ways
One’s place can be filled in 8 ways
Total number of numbers = 9*9*8 = 648
Number of integers in the set {100, 101, 102, …, 999} have at least one digit repeated = 900 – 648 = 252
Q8: Let m and n be natural numbers such that n is even and 0.2 < m/20, n/m, n/11 < 0.5. Then m - 2n equals.
(a) 3
(b) 1
(c) 2
(d) 4
Ans: (b)
Sol: 0.2 < n/11 < 0.5
⇒ 2.2 < n < 5.5
Since n is an even natural number, the value of n = 4
0.2 < m/20 < 0.5 ⇒ 4 < m > 10 . Possible values of m = 5,6,7,8,9
Since 0.2 < n/m < 0.5, the only possible value of m is 9
Hence m-2n = 9-8 = 1
Q9: If a, b and c are positive integers such that ab = 432, bc = 96 and c < 9, then the smallest possible value of a + b + c is
(a) 49
(b) 56
c) 59
(d) 46
Ans: (d)
Sol: Since c < 9, we can have the following viable combinations for b x c 96 (given our objective is to minimize the sum):
48 x 2; 32 × 3; 24 × 4; 16 × 6; 12×8
Similarly, we can factorize a × b = 432 into its factors. On close observation, we notice that 18 × 24 and 24 x 4 corresponding to a × b and b x c respectively together render us with the least value of the sum of a + b + c = 18 + 24 + 4 = 46
Hence, Option D is the correct answer.
Q10: The mean of all 4-digit even natural numbers of the form ‘aabb’, where a > 0 , is
(a) 4466
(b) 5050
(c) 4864
(d) 5544
Ans: (d)
Sol: The four digit even numbers will be of form:
1100, 1122, 1144 … 1188, 2200, 2222, 2244 … 9900, 9922, 9944, 9966, 9988
Their sum ‘S’ will be (1100 + 1100 + 22 + 1100 + 44 + 1100 + 66 + 1100 + 88) + (2200 + 2200 + 22 + 2200 + 44 +…)….+(9900 + 9900 + 22 + 9900 + 44 + 9900 + 66 + 9900 + 88)
=> S=1100*5 + (22 + 44 + 66 + 88) + 2200*5 + (22 + 44 + 66 + 88)….+ 9900*5 + (22 + 44 + 66 + 88)
=> S=5*1100(1 + 2 + 3 +…9) + 9(22 + 44 + 66 + 88)
=>S=5*1100*9*10/2 + 9*11*20
Total number of numbers are 9*5 = 45
∴ Mean will be S/45 = 5*1100 + 44 = 5544.
|
191 videos|131 docs|110 tests
|
FAQs on Important Solved Questions for CAT: Number Systems - Quantitative Aptitude (Quant)
| 1. What are the different types of number systems covered in CAT exams? |  |
| 2. How can I convert a decimal number to a fraction in the number system? |  |
| 3. How to find the least common multiple (LCM) of two or more numbers in the number system? |  |
| 4. How can I convert a fraction to a decimal in the number system? |  |
| 5. How do I solve complex number problems in the number system for CAT exams? |  |


















