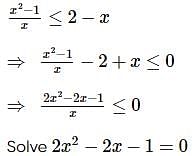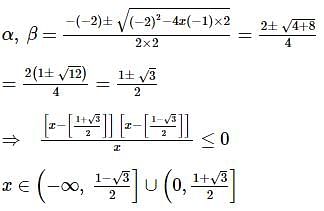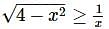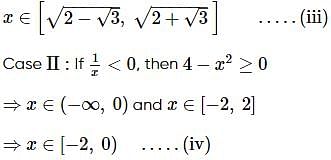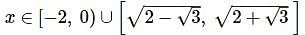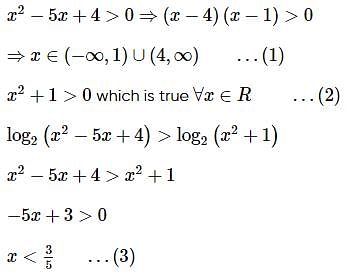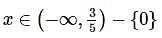BITSAT Mathematics Test - 3 - JEE MCQ
30 Questions MCQ Test BITSAT Mock Tests Series & Past Year Papers 2025 - BITSAT Mathematics Test - 3
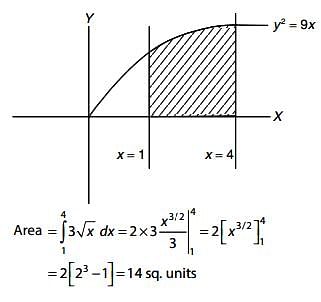
The area bounded by the curve y2 = 9x and the lines x = 1, x = 4 and y = 0 in the first quadrant is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If this scalar triple product of three non-zoro vectors is zero, then the vectors are
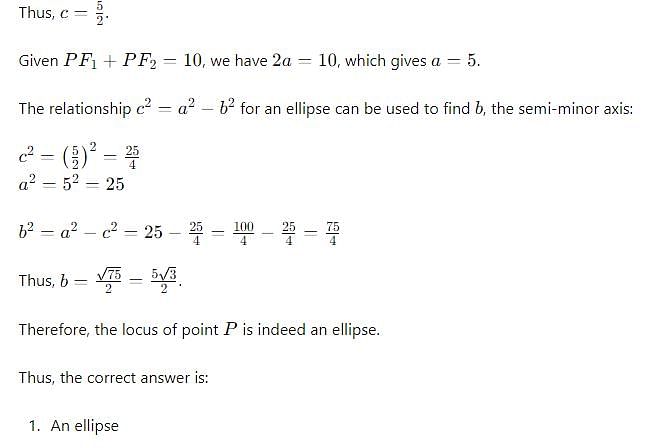
If F1 ≡ (0, 0), F2 ≡ (3, 4) and I PF1I + IPF2l = 10, then locus of p is
The area of the parallelogram, whose digonal are given by the vectors,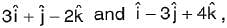 is
is
The area of triangle formed by the lines y = x, y = 2x and y = 3x + 4 is
Which of the following lines is a normal to the circle ( x - 1)2 + ( y - 2 )2 = 10?
Which of the following terms of the expansion of the following expression is independent of x?
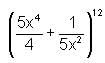
Let P, Q and R be the interior angles of a triangle PQR. What is the value of 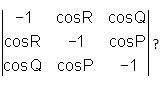
Which of the following equations is satisfied by the given function?
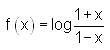
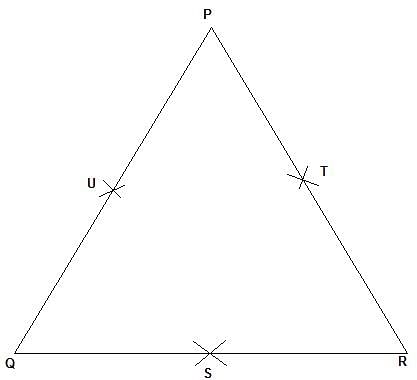
The mid-points of sides QR, RP and PQ of a triangle PQR are (p, 0, 0), (0, q, 0) and (0, 0, r). What is the value of 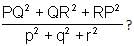
Directions: Consider the given lines.
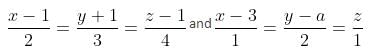
If L1 and L2 intersect at any point, then what is the value of a?
If f (x) = log5 + log (x3 - 3), where x [-1, 1], then find the value of c by using Rolle's theorem.
A tangent of a curve intercepts the y-axis at a point P, which is perpendicular to the tangent through another point (3, 1) on the curve. The differential equation of this curve is
A manager draws two pens from his drawer randomly and one by one. The drawer has three blue and three red pens. What is the probability that both of them are of different colours?
If α and β are the roots of the equation (log2x)2+4(log2x)−1=0 then the value of logβ α+logα β equals
Set of all real values of x satisfying the in equation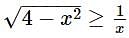 is
is
Set of all real values of x satisfying the in equation 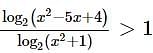 is
is
If a > 0, then the expression ax2 + bx + c is positive for all values of 'x' provided
|
2 videos|17 docs|85 tests
|
|
2 videos|17 docs|85 tests
|


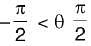
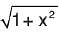
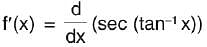
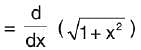
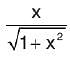
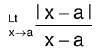 for x>a
for x>a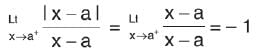

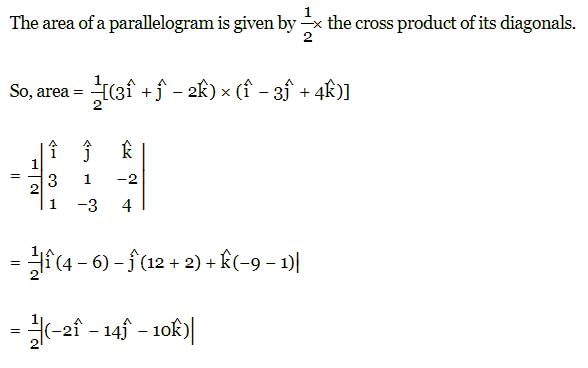
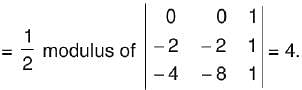
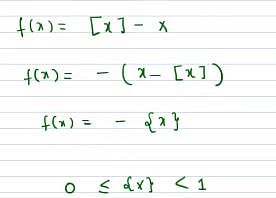
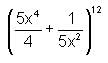
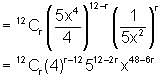
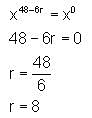
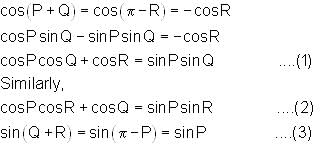
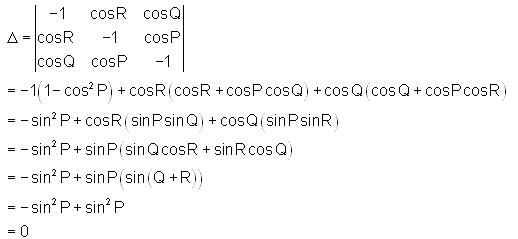
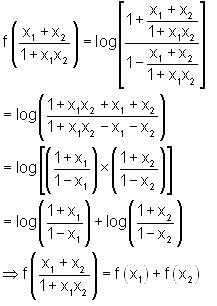
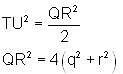
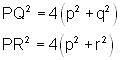
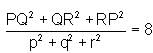
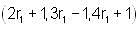 and on L2 is
and on L2 is 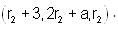
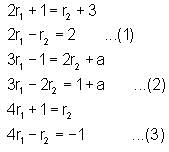
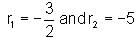
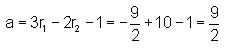
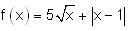 is continuous at
is continuous at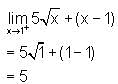
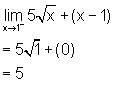
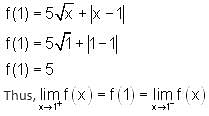
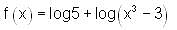
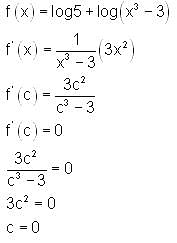
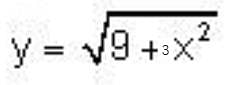 and
and 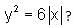
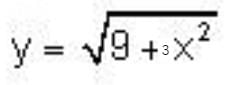
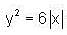
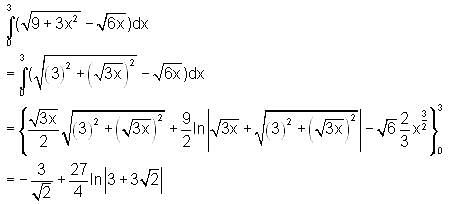
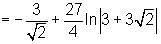
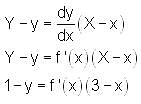
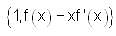
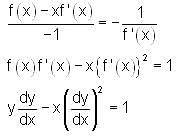
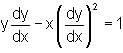
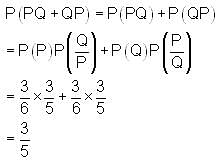
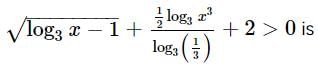
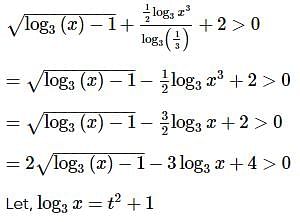
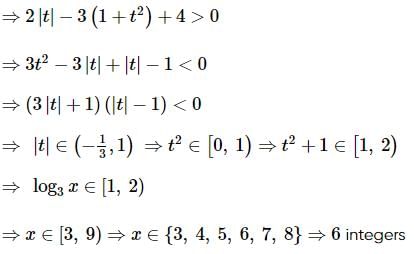
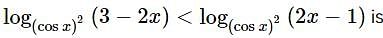
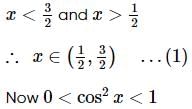
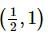
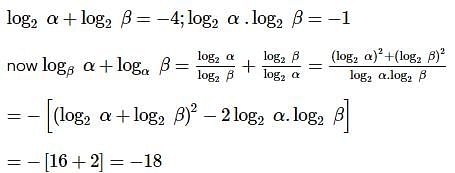
 then relation between a and b will be
then relation between a and b will be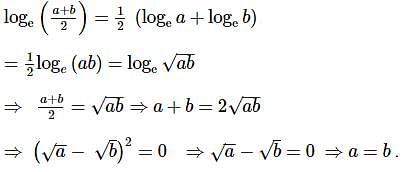
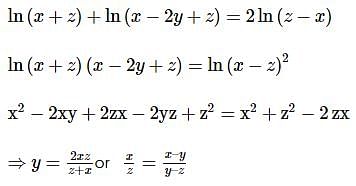
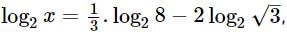 then x is equal to
then x is equal to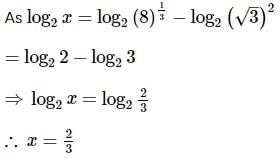
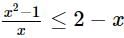 is
is