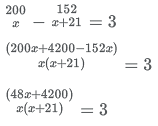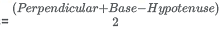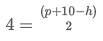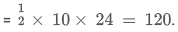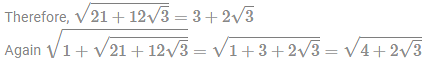CAT Mock Test - 16 (November 18) - CAT MCQ
30 Questions MCQ Test Daily Test for CAT Preparation - CAT Mock Test - 16 (November 18)
Directions: The passage below is accompanied by a set of questions. Choose the best answer to each question.
A season of mists and mellow fruitfulness it may be. But autumn is also the fall, and there is little fruitfulness to be had from leafless plants. This does not matter in nature. The winter dieback has a reason: the cost of maintaining the leaves, and the risk of storm and frost damage, exceed the photosynthetic benefits to be from the pallid winter sunshine. But the interests of vegetables and the interests of their growers do not always coincide. The latter would like the former to flourish all year around.
Although artificial light can fool plants into thinking that autumn has not actually arrived, this is expensive in electricity. A new technique developed by Richard Amasino and Susheng Gan, of the University of Wisconsin-Madison, may fool plants on the cheap. They have devised a way to persuade their charges to keep their leaves even when the external signals are warning that winter is nigh. As a bonus, the method also seems to keep plants green and fresh for longer after they have been cut.
It is done by meddling with the genes. When a plant prepares to drop its leaves, it first evacuates as much protein from them as possible. Annual plants recycle this protein into their seeds; perennials transport it into storage in the trunk or stem. In nature, the biochemical changes that carry out this mobilization are governed by a genetic promoter: a DNA switch that starts up the genes that code for the enzymes required for the autumnal changes to take place.
Dr. Amasino and Mr. Gan have isolated this promoter and attached it to a new genetic partner. The gene they chose is for the enzyme that produces cytokinin, a hormone that invigorates many plant tissues, including the leaves. They have inserted their new gene promoter combination into tobacco and arabidopsis (a weed much favored by plant geneticists as a workhorse for experiments).
The idea was that, when autumnal changes switched on the natural promoter to start the process of leaf senescence, they would also switch on the synthetic promoter for the cytokinin gene, nullifying the message to close down the leaf. Cytokinin would be produced until it had managed to keep the leaves green and healthy. At that point it would shut itself off. In this way the system would be self regulating; the plant would never produce enough hormone to have side-effects beyond the leaves.
It worked. Leaves from transgenic plants stayed green for the whole of a 20 weeks trial period, while control leaves gradually turned yellow. Even when picked, leaves from transgenic plants stayed green for around 40 days. Dr. Amasino and Mr. Gan see their discovery's first use as being to protect leaf vegetables such as lettuces and cabbages and to prolong their shelf life. But the new genetic combination could eventually be applied more widely. The added growth permitted by extra weeks or months of photosynthesis could boost yields of grain, and of flowers, from crops containing the hybrid gene. The transgenic tobacco plants turned out to weight 50% more seed, than normal plants. Fruitful indeed.
Q. Each of the following is true about the Amasino-Gan technique EXCEPT:
Although artificial light can fool plants into thinking that autumn has not actually arrived, this is expensive in electricity. A new technique developed by Richard Amasino and Susheng Gan, of the University of Wisconsin-Madison, may fool plants on the cheap. They have devised a way to persuade their charges to keep their leaves even when the external signals are warning that winter is nigh. As a bonus, the method also seems to keep plants green and fresh for longer after they have been cut.
It is done by meddling with the genes. When a plant prepares to drop its leaves, it first evacuates as much protein from them as possible. Annual plants recycle this protein into their seeds; perennials transport it into storage in the trunk or stem. In nature, the biochemical changes that carry out this mobilization are governed by a genetic promoter: a DNA switch that starts up the genes that code for the enzymes required for the autumnal changes to take place.
Dr. Amasino and Mr. Gan have isolated this promoter and attached it to a new genetic partner. The gene they chose is for the enzyme that produces cytokinin, a hormone that invigorates many plant tissues, including the leaves. They have inserted their new gene promoter combination into tobacco and arabidopsis (a weed much favored by plant geneticists as a workhorse for experiments).
The idea was that, when autumnal changes switched on the natural promoter to start the process of leaf senescence, they would also switch on the synthetic promoter for the cytokinin gene, nullifying the message to close down the leaf. Cytokinin would be produced until it had managed to keep the leaves green and healthy. At that point it would shut itself off. In this way the system would be self regulating; the plant would never produce enough hormone to have side-effects beyond the leaves.
It worked. Leaves from transgenic plants stayed green for the whole of a 20 weeks trial period, while control leaves gradually turned yellow. Even when picked, leaves from transgenic plants stayed green for around 40 days. Dr. Amasino and Mr. Gan see their discovery's first use as being to protect leaf vegetables such as lettuces and cabbages and to prolong their shelf life. But the new genetic combination could eventually be applied more widely. The added growth permitted by extra weeks or months of photosynthesis could boost yields of grain, and of flowers, from crops containing the hybrid gene. The transgenic tobacco plants turned out to weight 50% more seed, than normal plants. Fruitful indeed.
Directions: Read the following passage and answer the questions:
The target audience is likely to switch channels in an attempt to whittle away the time while waiting for their favorite program to resume. Even if the ad does reach the desired target audience, the message conveyed comes and departs mostly unnoticed and many people would have trouble recalling an ad that they had seen just two minutes ago. Companies however continue to spend billions of dollars every year in an effort to attract buyers.
Instead of paying massive endorsement fees to celebrities to promote a brand and spending a pretty penny on conceptualizing and creating ads, would it not make more sense for the behemoth corporations to save that money and pass on a large share back to the consumers as loyalty benefits? A satisfied user is often the best publicity and companies can simply divide the amount of money they are going to spend on publicity of a product by the expected number of units they are going to sell in that year and share the average expected per unit savings with the consumers.
Even if the companies decide to keep a substantial portion of money saved from advertising for themselves and directly pass on the rest to consumers as an across the board price cutback, it can help the consumers to buy more for less in these times of tight liquidity.
Companies keep spending so much money on advertising because they realize that the subconscious mind is working even when the conscious mind tunes out the message. Once the subconscious learns something, that information gets stored in the vast labyrinths of the neural passages that are present in our brains and like a burr beneath the saddle of a horse, the message will keep irritating our subconscious, egging us on to buy the proffered product or service. Also, with the customer being constantly bombarded by inducements for buying, a vacillating mind might just get seduced into pulling out the credit card.
Another reason could be that the advertisers and advertising agencies are relying on a collective message that they send out to teeming humanity of equating material possessions with happiness. Another startling input could be that the promoter knows that only ten percent of advertising converts into a sale and therefore the buyers end up paying for the message that the remaining 90% did not heed. In this cat and mouse game of one trying to tempt another into buying, perhaps it is the advertising agency that has the last laugh – their product is selling even if the advertiser’s is not and the fall guy for the entire con game is the consumer who is paying for it.
Q. What according to the passage is not the reason why companies spend so much on advertising?
Instead of paying massive endorsement fees to celebrities to promote a brand and spending a pretty penny on conceptualizing and creating ads, would it not make more sense for the behemoth corporations to save that money and pass on a large share back to the consumers as loyalty benefits? A satisfied user is often the best publicity and companies can simply divide the amount of money they are going to spend on publicity of a product by the expected number of units they are going to sell in that year and share the average expected per unit savings with the consumers.
Even if the companies decide to keep a substantial portion of money saved from advertising for themselves and directly pass on the rest to consumers as an across the board price cutback, it can help the consumers to buy more for less in these times of tight liquidity.
Companies keep spending so much money on advertising because they realize that the subconscious mind is working even when the conscious mind tunes out the message. Once the subconscious learns something, that information gets stored in the vast labyrinths of the neural passages that are present in our brains and like a burr beneath the saddle of a horse, the message will keep irritating our subconscious, egging us on to buy the proffered product or service. Also, with the customer being constantly bombarded by inducements for buying, a vacillating mind might just get seduced into pulling out the credit card.
Another reason could be that the advertisers and advertising agencies are relying on a collective message that they send out to teeming humanity of equating material possessions with happiness. Another startling input could be that the promoter knows that only ten percent of advertising converts into a sale and therefore the buyers end up paying for the message that the remaining 90% did not heed. In this cat and mouse game of one trying to tempt another into buying, perhaps it is the advertising agency that has the last laugh – their product is selling even if the advertiser’s is not and the fall guy for the entire con game is the consumer who is paying for it.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Directions: Read the following passage and answer the questions:
But the reason that mathematicians are not intuitive is that they do not see what is before them, and that, accustomed to the exact and plain principles of mathematics, and not reasoning till they have well inspected and arranged their principles, they are lost in matters of intuition where the principles do not allow of such arrangement.
They are scarcely seen; they are felt rather than seen; there is the greatest difficulty in making them felt by those who do not of themselves perceive them. These principles are so fine and so numerous that a very delicate and very clear sense is needed to perceive them, and to judge rightly and justly when they are perceived, without for the most part being able to demonstrate them in order as in mathematics; because the principles are not known to us in the same way, and because it would be an endless matter to undertake it.
We must see the matter at once, at one glance, and not by a process of reasoning, at least to a certain degree. And thus it is rare that mathematicians are intuitive, and that men of intuition are mathematicians, because mathematicians wish to treat matters of intuition mathematically, which is not the way to proceed in this kind of reasoning. Not that the mind does not do so, but it does it tacitly, naturally, and without technical rules.
Intuitive minds, on the contrary, being thus accustomed to judge at a single glance, are so astonished when they are presented with propositions of which they understand nothing, and the way to which is through definitions and axioms so sterile, and which they are not accustomed to see thus in detail, that they are repelled and disheartened.
Q. The tone of the author is
They are scarcely seen; they are felt rather than seen; there is the greatest difficulty in making them felt by those who do not of themselves perceive them. These principles are so fine and so numerous that a very delicate and very clear sense is needed to perceive them, and to judge rightly and justly when they are perceived, without for the most part being able to demonstrate them in order as in mathematics; because the principles are not known to us in the same way, and because it would be an endless matter to undertake it.
We must see the matter at once, at one glance, and not by a process of reasoning, at least to a certain degree. And thus it is rare that mathematicians are intuitive, and that men of intuition are mathematicians, because mathematicians wish to treat matters of intuition mathematically, which is not the way to proceed in this kind of reasoning. Not that the mind does not do so, but it does it tacitly, naturally, and without technical rules.
Intuitive minds, on the contrary, being thus accustomed to judge at a single glance, are so astonished when they are presented with propositions of which they understand nothing, and the way to which is through definitions and axioms so sterile, and which they are not accustomed to see thus in detail, that they are repelled and disheartened.
Directions: The passage below is followed by a question based on its content. Answer the question on the basis of what is stated or implied in the passage.
Today, the import duty on a complete machine is 35% for all practical purposes, whereas the import duty on raw materials and components ranges from 40% - 85%. The story does not end here. After paying such high import duties on components, once a machine is made, it suffers excise duty from 5% - 10% (including on the customs duty already paid). At the time of sale, the machine tools suffer further taxation, i.e., Central Sales Tax or State Sales Taxes which range from 4% - 16%. This much from the tax angle. Another factor which pushes the cost of manufacture of machine tools is in advanced countries.
The production of machine tools in India being not of the same scale as it is in other countries, the price which India's machine tool builders have to pay for components is more or less based on pattern of high pricing applicable to the prices of spares. The above represents only a few of the extraneous reasons for the high cost of India machines.
The machine tool industry in India has an enviable record of very quick technology absorption, assimilation and development. Machine tools play a key role in the expansion of industrial production since nearly all products are manufactured by machines of some sort; the machine tool is not an end product per se, but it is the means of manufacturing the end product. There are a number of success stories about how machine tool builders were of help at the most critical times. It will be a pity, in fact a tragedy, if we allow this industry to die and disappear from the scene.
It is to be noted that India is at least 6000 km away from any dependable source of supply of machine tools. The Government of India has always given a great deal of importance to the development of small scale and medium scale industries. This industry has also performed pretty well. Today, they are in need of help from India's machine tool industry to enable them to produce quality components at reduced costs. Is it anybody's case that the needs of this fragile sector (which needs tender care) will be met from 6000 km away?
Then, what is it that the industry requests from the Government? It wants a level playing field. In fact all of us must have a deep introspection and recognize the fact that the machine tool industry has a very special place in the country from the point of strategic and vital interests of the nation. Most important, it requests for the Government's consideration and understanding. It is therefore high time that the government gives the due attention to this industry which has a good potential.
Q. None of the following statements can be inferred from the passage EXCEPT:
Directions: The passage below is followed by a question based on its content. Answer the question on the basis of what is stated or implied in the passage.
Today, the import duty on a complete machine is 35% for all practical purposes, whereas the import duty on raw materials and components ranges from 40% - 85%. The story does not end here. After paying such high import duties on components, once a machine is made, it suffers excise duty from 5% - 10% (including on the customs duty already paid). At the time of sale, the machine tools suffer further taxation, i.e., Central Sales Tax or State Sales Taxes which range from 4% - 16%. This much from the tax angle. Another factor which pushes the cost of manufacture of machine tools is in advanced countries.
The production of machine tools in India being not of the same scale as it is in other countries, the price which India's machine tool builders have to pay for components is more or less based on pattern of high pricing applicable to the prices of spares. The above represents only a few of the extraneous reasons for the high cost of India machines.
The machine tool industry in India has an enviable record of very quick technology absorption, assimilation and development. Machine tools play a key role in the expansion of industrial production since nearly all products are manufactured by machines of some sort; the machine tool is not an end product per se, but it is the means of manufacturing the end product. There are a number of success stories about how machine tool builders were of help at the most critical times. It will be a pity, in fact a tragedy, if we allow this industry to die and disappear from the scene.
It is to be noted that India is at least 6000 km away from any dependable source of supply of machine tools. The Government of India has always given a great deal of importance to the development of small scale and medium scale industries. This industry has also performed pretty well. Today, they are in need of help from India's machine tool industry to enable them to produce quality components at reduced costs. Is it anybody's case that the needs of this fragile sector (which needs tender care) will be met from 6000 km away?
Then, what is it that the industry requests from the Government? It wants a level playing field. In fact all of us must have a deep introspection and recognize the fact that the machine tool industry has a very special place in the country from the point of strategic and vital interests of the nation. Most important, it requests for the Government's consideration and understanding. It is therefore high time that the government gives the due attention to this industry which has a good potential.
Q. When the author states that the machine tool industry 'wants a level playing field' in the passage, he implies that it
Directions: Read the following passage and answer the question that follows.
The Communist Party of China was founded in 1921 and Mao was one of the 13 founder members. In 1945, he was elected Chairman of the Communist Party of China and that position he held until his death in 1976. Many have called Mao not only ruthless but also crude. He had no hesitation publicly to exhort his close comrades to "break the wind." However, the man of power was also a man of poetry. He could resort, by turn, to inspiration, eloquence, persuasion, intimidation and liquidation. He could act, almost simultaneously, as an imperious Emperor as well as "a lone monk walking in the world with a leaky umbrella."
Although he was considered to be infallible, he made many mistakes of a serious nature. The Hundred Flowers not only failed to bloom but turned into poisonous weeds. The Great Leap Forward turned out to be a total failure. His two handpicked successors proved to be Vipers under his pillow. Whatever the compulsion that forced him to launch the Great Proletarian Cultural Revolution, the convulsions caused by it haunted China for long. India cannot forgive him for her humiliation at his hands in 1962.
Mao believed that to some extent the personality cult was indispensable. He is said to have observed, "Khrushchev had fallen because he had no cult of personality at all." On one occasion, Mao is said to have observed that the "four greats" – Great Teacher, Great Leader, Great Supreme Commander and Great Helmsman – thrust on him were a "nuisance". He was confident that sooner or later three of them will disappear and only the Teacher will remain.
While adapting Marxist theories to the Chinese environment, Mao was an innovator. He was firmly of the view that the Marxist principles had to be adjusted to the circumstances prevailing in China and could not be used in an abstract manner. To talk of Marxism without taking into consideration the Chinese peculiarities, was an empty abstraction. To quote him, "Consequently, the significance of Marxism that is to say, making certain that in all of its manifestations it is imbued with Chinese peculiarities, becomes a problem that must be understood and solved by the whole party without delay. We must put an end to writing eight legged essays on foreign models. We must discard our dogmatism and replace it by a new and vital Chinese style and manner pleasing to the eye and to the ear of the Chinese common people." Mao put Marxism in Chinese dress. He used anecdotes from the ancient writings from Chinese history and the language of the peasants to make his political points with telling force.
Mao's great contribution to the Chinese Revolution was his reliance on the peasants as a revolutionary force. When the uprisings in the cities failed, Mao realised that China did not possess an urban proletariat in the Marxist sense and hence he had to depend upon the peasants for a revolution in the country. Mao's confidence in peasant initiative and resourcefulness became a cause of tension between him and the Politburo's pragmatists after liberation.
Q. Which one of the following best describes Mao's views regarding Marxism?
Directions: The passage given below is followed by four alternative summaries. Choose the option that best captures the essence of the passage.
The school-to-prison pipeline, a "partnership'' between juvenile courts and the school system, "developed through a punitive and harmful framework to the detriment of many vulnerable children and adolescents,'' is a phenomenon of the late twentieth century. However, juvenile courts and the school system have been historically linked through their focus on controlling young people perceived as troublesome. As early as the 1800s and as late as the 1970s, corporal punishment, such as beatings with a ruler, was used by many schools as a normalized method of dealing with students "acting out.'' The popularity of corporal punishment was diminished as school populations grew during the 1970s, forcing schools to turn to suspensions and expulsions to keep disruptive students away from classrooms.
Directions: There is a sentence that is missing in the paragraph below. Look at the paragraph and decide in which blank (option 1, 2, 3, or 4) the following sentence would best fit.
Sentence: But any obligation to be kind to animals merely concerned animals; the obligation of kind treatment was owed only to other humans.
Paragraph: Before the 19th century, at least in the West, animals were largely excluded from the moral and legal community. ___(1)___. They were considered to be things. This is contrasted with Eastern thinking, which generally accorded at least some moral value to animals that accounted for the vegetarianism that remains prevalent in the Jain, Hindu and most Buddhist traditions. ___(2)___. The Western view was that we could have moral and legal obligations that concerned animals but were not owed to them. ___(3)___. To the extent that the cruel treatment of animals was thought to present a moral problem, it was only because it made us more likely to be cruel to other humans. ___(4)___. This was the view of Immanuel Kant, Thomas Aquinas and others. We had a legal obligation not to harm our neighbour's property - whether that property was a cow or a cart. But that was an obligation owed to our neighbour as a property owner, not to the cow or the cart.
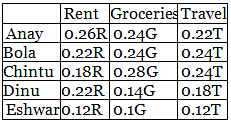
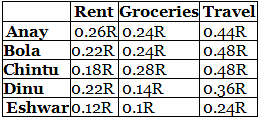
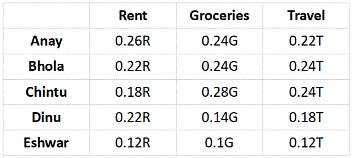
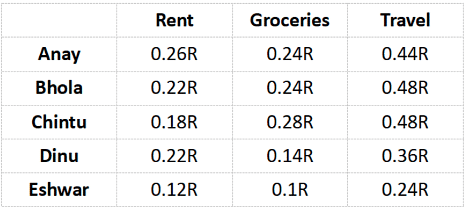
5 friends Anay, Bhola, Chintu, Dinu and Eshwar spend their entire salary on Rent, Groceries and Travel. The following graph shows the amount of money spent by each of the 5 friends on a particular expense as a percentage of the total amount of money spent by all of the 5 friends on a particular expense. For example, if the amount spend by all the friends on Rent is ‘R’ then the amount spent by Anay on rent is 0.26R. The total amount of money spent by exactly 3 friends is equal.
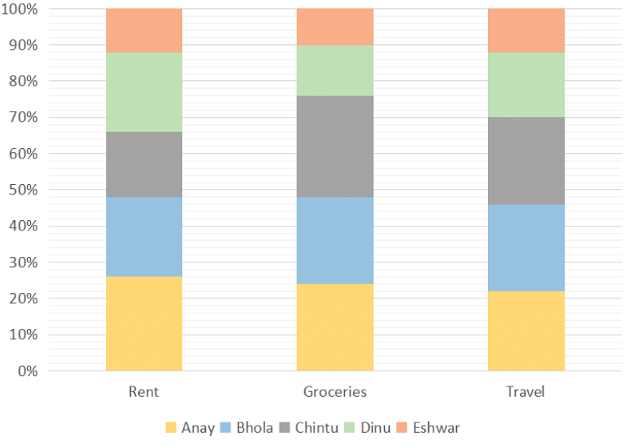
Q. What is the ratio of the amount spent by Chintu on Rent and the amount spent by Eshwar on Travelling?
5 friends Anay, Bhola, Chintu, Dinu and Eshwar spend their entire salary on Rent, Groceries and Travel. The following graph shows the amount of money spent by each of the 5 friends on a particular expense as a percentage of the total amount of money spent by all of the 5 friends on a particular expense. For example, if the amount spend by all the friends on Rent is ‘R’ then the amount spent by Anay on rent is 0.26R. The total amount of money spent by exactly 3 friends is equal.
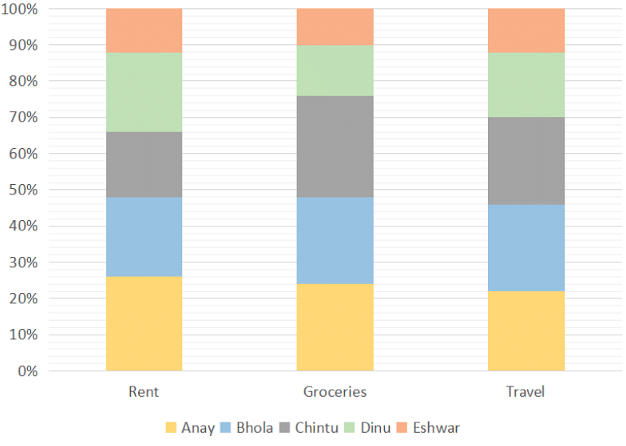
Q.If the amount spent on Groceries is 90000 then what is the amount spent by Anay on Travelling?
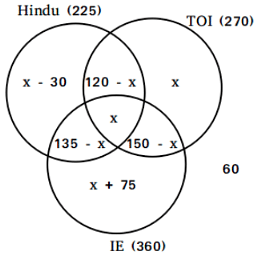
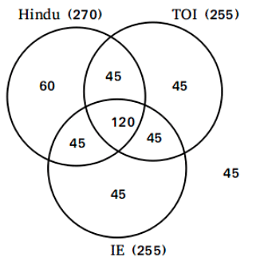
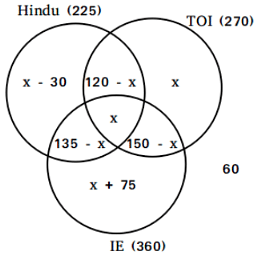
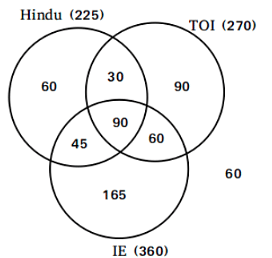
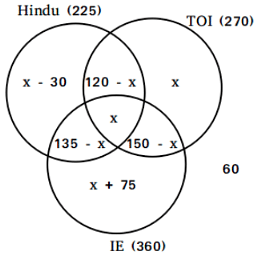
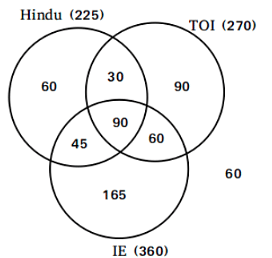
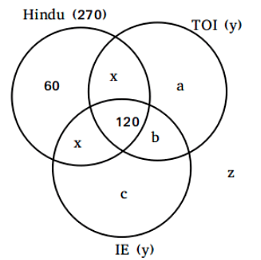
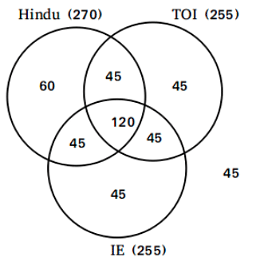
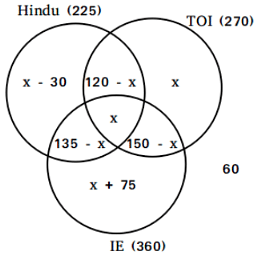
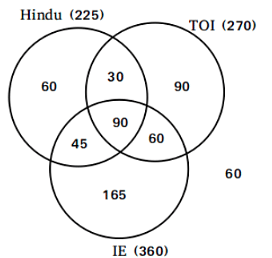
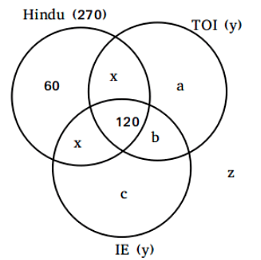
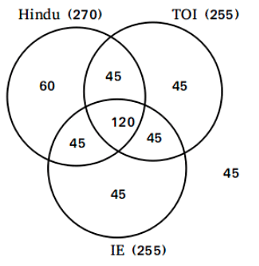
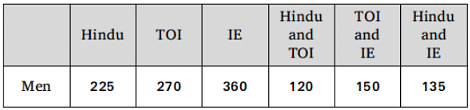
A survey was conducted among 1050 members of a locality. There were asked about the newspaper they read among Hindu, TOI and IE. Some of the results of the survey have been tabulated as under.
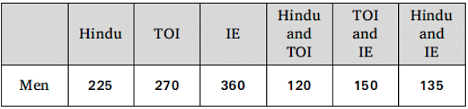
It is also known that:
I) The number of women who read only Hindu and IE is equal to the number of women who read only Hindi and TOI.
II) 20% of the people surveyed read all the three newspapers. The number of people who read exactly two newspaper is thrice the number of men who read all the three newspapers.
III) The number of women who read only Hindu is equal to the number of men who read only TOI and IE. The number of women surveyed is 150 less than the number of men surveyed.
IV) An equal number of women read TOI and IE. 495 people read Hindu and 435 people do not read IE. 10% of men read none of the newspapers.
How many women do not read any newspaper?
A survey was conducted among 1050 members of a locality. There were asked about the newspaper they read among Hindu, TOI and IE. Some of the results of the survey have been tabulated as under.
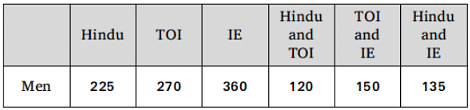
It is also known that:
I) The number of women who read only Hindu and IE is equal to the number of women who read only Hindi and TOI.
II) 20% of the people surveyed read all the three newspapers. The number of people who read exactly two newspaper is thrice the number of men who read all the three newspapers.
III) The number of women who read only Hindu is equal to the number of men who read only TOI and IE. The number of women surveyed is 150 less than the number of men surveyed.
IV) An equal number of women read TOI and IE. 495 people read Hindu and 435 people do not read IE. 10% of men read none of the newspapers.
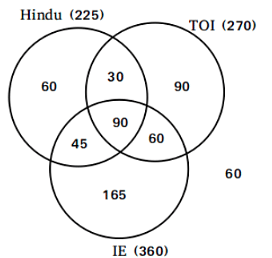
How many women read TOI and IE both?
A survey was conducted among 1050 members of a locality. There were asked about the newspaper they read among Hindu, TOI and IE. Some of the results of the survey have been tabulated as under.
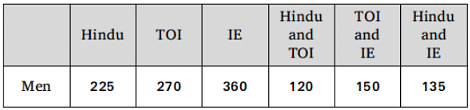
It is also known that:
I) The number of women who read only Hindu and IE is equal to the number of women who read only Hindi and TOI.
II) 20% of the people surveyed read all the three newspapers. The number of people who read exactly two newspaper is thrice the number of men who read all the three newspapers.
III) The number of women who read only Hindu is equal to the number of men who read only TOI and IE. The number of women surveyed is 150 less than the number of men surveyed.
IV) An equal number of women read TOI and IE. 495 people read Hindu and 435 people do not read IE. 10% of men read none of the newspapers.
How many people read Hindu and TOI both?
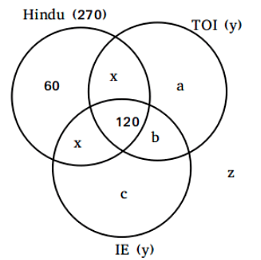
A survey was conducted among 1050 members of a locality. There were asked about the newspaper they read among Hindu, TOI and IE. Some of the results of the survey have been tabulated as under.
It is also known that:
I) The number of women who read only Hindu and IE is equal to the number of women who read only Hindi and TOI.
II) 20% of the people surveyed read all the three newspapers. The number of people who read exactly two newspaper is thrice the number of men who read all the three newspapers.
III) The number of women who read only Hindu is equal to the number of men who read only TOI and IE. The number of women surveyed is 150 less than the number of men surveyed.
IV) An equal number of women read TOI and IE. 495 people read Hindu and 435 people do not read IE. 10% of men read none of the newspapers.
How many people read exactly one newspaper?
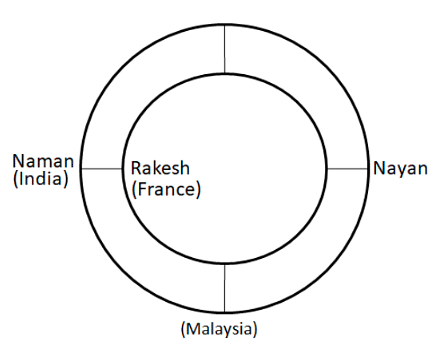
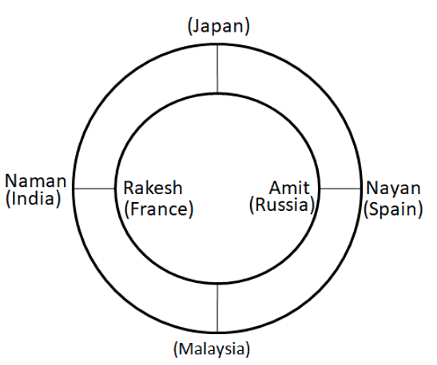
Eight delegates namely - Amit, Aman, Rajesh, Rakesh, Hitesh, Himesh, Naman and Nayan are seated in two concentric circles, with four person around each circle in such a manner that each member of inner circle sits exactly opposite to one of the members of the outer circle. Each of the delegates represents a different nation namely India, China, Japan, Russia, Nepal, Malaysia, France and Spain not necessarily in the same order. Further it is given that:
Amit, who is from Russia, faces the immediate neighbour of the person who is from Japan.
Naman is sitting second to left of Nayan and the delegates from Nepal and India are seated in different circles.
Rakesh is not from either China or Nepal and faces Naman, who is neither from Malaysia nor from Spain.
The delegate from France faces the delegate from India.
The members sitting in the outer circle are Hitesh, Himesh, Naman and Nayan and all of them are facing towards the centre.
The delegate who is from Malaysia is sitting immediate left of Nayan. Himesh, who is not from Japan, is not facing Amit.
The delegates from Malaysia and Spain are immediate neighbours and one of them is facing Aman, who is from China.
The delegates who are from France and Russia are seared in the same circle but they are not immediate neighbours.
The members sitting in the inner circle are Amit, Aman, Rajesh and Rakesh and all of them are facing away from the centre.
Who is facing the Malaysian delegate?
Directions: Read the following information and answer the question the follows:
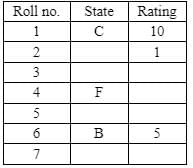
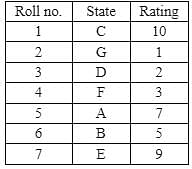
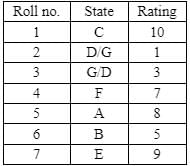
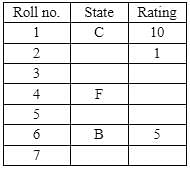
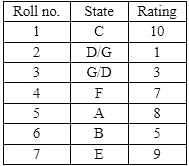
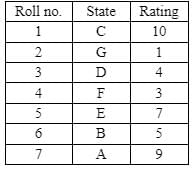
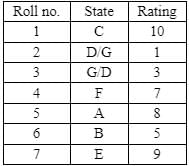
A faculty member of a renowned JEE coaching institute was tasked with assessing seven students from different states – named A, B, C, D, E, F, and G - for potential inclusion in their special batch. To maintain impartiality, each student was given a distinct roll number, ranging from 1 to 7. The faculty member evaluated these students on a 10-point scale based on their interview performances, with '10' being the highest score and '1' the lowest. Each student received a unique rating.
Additional known details are as follows:
(i) The student assigned roll number 4 is from state F.
(ii) The student from state C received the highest score, which is an even number.
(iii) The student with roll number 1 scored twice as much as the student with roll number 6.
(iv) Only two students received even-numbered scores. The student from state A scored 5 points more than the student with roll number 3.
(v) The student with roll number 2 received the lowest score but is not from state A.
(vi) The student with roll number 7 scored higher than the student with roll number 5 but lower than the student with roll number 1.
(vii) The student from state F scored higher than the student from state G but lower than the student from state E. None of these three students received an even-numbered score.
(viii) The student assigned roll number 6 is from state B.
Q. What is the maximum possible sum of the ratings got by all the students?
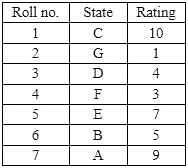
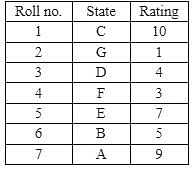
Directions: Read the following information and answer the question the follows:
A faculty member of a renowned JEE coaching institute was tasked with assessing seven students from different states – named A, B, C, D, E, F, and G - for potential inclusion in their special batch. To maintain impartiality, each student was given a distinct roll number, ranging from 1 to 7. The faculty member evaluated these students on a 10-point scale based on their interview performances, with '10' being the highest score and '1' the lowest. Each student received a unique rating.
Additional known details are as follows:
(i) The student assigned roll number 4 is from state F.
(ii) The student from state C received the highest score, which is an even number.
(iii) The student with roll number 1 scored twice as much as the student with roll number 6.
(iv) Only two students received even-numbered scores. The student from state A scored 5 points more than the student with roll number 3.
(v) The student with roll number 2 received the lowest score but is not from state A.
(vi) The student with roll number 7 scored higher than the student with roll number 5 but lower than the student with roll number 1.
(vii) The student from state F scored higher than the student from state G but lower than the student from state E. None of these three students received an even-numbered score.
(viii) The student assigned roll number 6 is from state B.
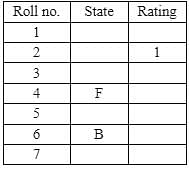
Q. If the rating of the student from state D is 2, then what is the absolute difference between the ratings of the students who belong to state G and state A?
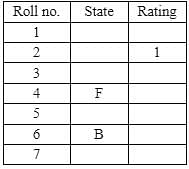
Directions: Read the following information and answer the question the follows:
A faculty member of a renowned JEE coaching institute was tasked with assessing seven students from different states – named A, B, C, D, E, F, and G - for potential inclusion in their special batch. To maintain impartiality, each student was given a distinct roll number, ranging from 1 to 7. The faculty member evaluated these students on a 10-point scale based on their interview performances, with '10' being the highest score and '1' the lowest. Each student received a unique rating.
Additional known details are as follows:
(i) The student assigned roll number 4 is from state F.
(ii) The student from state C received the highest score, which is an even number.
(iii) The student with roll number 1 scored twice as much as the student with roll number 6.
(iv) Only two students received even-numbered scores. The student from state A scored 5 points more than the student with roll number 3.
(v) The student with roll number 2 received the lowest score but is not from state A.
(vi) The student with roll number 7 scored higher than the student with roll number 5 but lower than the student with roll number 1.
(vii) The student from state F scored higher than the student from state G but lower than the student from state E. None of these three students received an even-numbered score.
(viii) The student assigned roll number 6 is from state B.
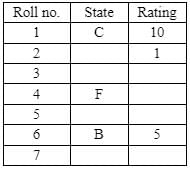
Q. If the rating of the student from state G is 3, then the sum of the ratings of the those who got even numbered ratings is
Directions: Read the following information and answer the question the follows:
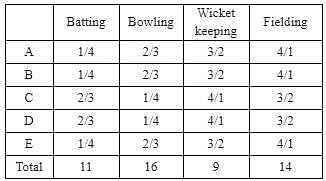
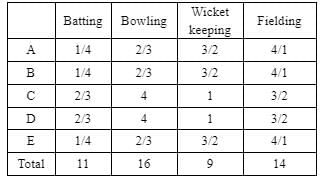
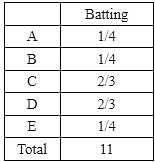
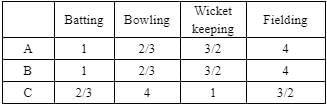
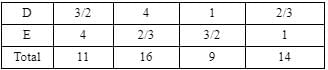
In a local park a cricket match was going on between two teams, these players didn’t know that 5 national team selectors were speculating Ram (one of the players playing with them). The names of selectors are A, B, C, D and E. Ram was graded by these 5 selectors on the following parameters: Batting, Bowling, wicket keeping and fielding from 1(lowest) to 4(highest). While grading Ram on four attributes, no selector gave the same grade to two attributes.
The graph below shows the average grade of each of the attributes.
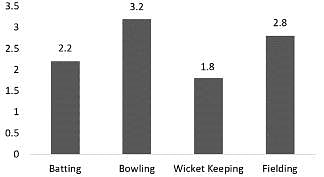
The following table shows the attribute which was not given grade 1 or 4 by the selectors.

Q. Which of the following could be an accurate list of grades given by A, If A and B both gave same grade in Batting?
If all the permutations of the letters of the word 'AGAIN' be arranged as in a dictionary, then the rank of the word 'NAAIG' shall be
The average of 7 natural numbers is 23.142857. If one number which is 7, is removed, it is observed that the other numbers are in the ratio 1:3:4:5:7:11, then find the difference between the largest and the second largest number.
How many 4 digit numbers, having all distinct digits exist, such that the digits are all in ascending order?
Suppose hospital A admitted 21 less Covid infected patients than hospital B, and all eventually recovered. The sum of recovery days for patients in hospitals A and B were 200 and 152, respectively. If the average recovery days for patients admitted in hospital A was 3 more than the average in hospital B then the number admitted in hospital A was
In how many ways 3 girls and 9 boys can be seated in two bus, each having numbered seats, 3 in the front and 4 at the back, if the 3 girls sit together in a back row on adjacent seats?
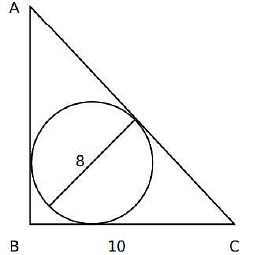
A circle of diameter 8 inches is inscribed in a triangle ABC. where ∠ABC = 90° if BC = 10 inches then the area of the triangle in square inches is
Three boxes A, B, C each contain 4 white balls and 5 black balls. All the balls are identical except for the colour. A ball is shifted from box A to box B and then a ball is shifted from box B to box C and finally a ball is shifted from box C to box A. The probability that each of the boxes will contain again 4 white balls and 5 black balls is
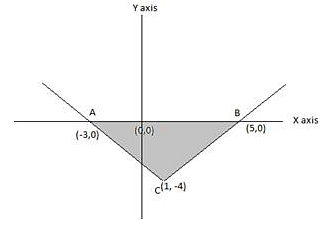
Find the area of region common to y = |x-1| - 4 and between the axis?
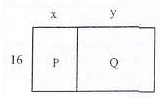
In a rectangle, length of the smaller side is 16 cm. A line parallel to the smaller side is drawn such that the rectangle is divided into two rectangles P and Q such that one of the rectangles is similar to the original rectangle. How many distinct pairs of rectangles P and Q are possible? {given that the sides of both the rectangles P and Q are integral values}
In the figure given below AG and BG are diameters of two circles with radii 2 cm and 6 cm respectively. G is the only point of contact of the two circles. BC is a tangent of length 12 cm to the bigger circle and BF is perpendicular to the line AC. Find the length of CF.
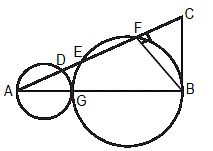
|
152 docs|327 tests
|