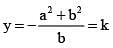NDA Mock Test: Mathematics - 6 - NDA MCQ
30 Questions MCQ Test NDA (National Defence Academy) Mock Test Series 2024 - NDA Mock Test: Mathematics - 6
Two sets, A and B, are as under:
A = {(a, b) ∈ R × R : |a − 5| < 1 and |b − 5| < 1};
B = {a, b) ∈ R × R : 4 (a − 6)2 + 9(b−5) 2 ≤ 36}. Then,
A = {(a, b) ∈ R × R : |a − 5| < 1 and |b − 5| < 1};
B = {a, b) ∈ R × R : 4 (a − 6)2 + 9(b−5) 2 ≤ 36}. Then,
If A = {2, 3, 4, 8, 10}, B = {3, 4, 5, 10, 12}, C = {4, 5, 6, 12, 14} then (A ∩ B) ∪ (A ∩ C) is equal to:
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If f(x) = x – x2, then f(a + 1) – f(a – 1) , a ∈ R is :
The minor Mij of an element aij of a determinant is defined as the value of the determinant obtained after deleting the
If z is a complex number such that 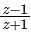 is purely imaginary, then what is |z| equal to ?
is purely imaginary, then what is |z| equal to ?
Let Tn denote the number of triangles which can be formed using the vertices of a regular polygon of n sides. If Tn + 1 - Tn = 21, then n equals (2001S)
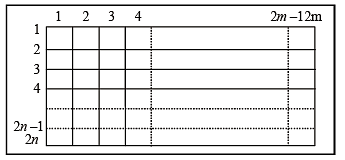
A rectangle with sides of length (2m – 1) an d (2n – 1) units is divided into squares of unit length by drawing parallel lines as shown in the diagram, then the number of rectangles possible with odd side length s is (2005S)
In how many ways, a party of 5 men and 5 women be seated at a circular table, so that no two women are adjacent?
In how many ways can 4 red, 3 yellow and 2 green chairs be arranged in a row if the chairs of the same colour are indistinguishable?
If the coefficients of (r +1)th term and (r + 3)th term in the expansion of (1+x)2n be equal then
If in the expansion of(1+x)43, the coefficients of (2r+1)th and (r+2)th terms are equal, then r is equal to
Let Tr be the rth term of an A.P. whose first term is a and common difference is d. If for some positive integers m, n, m ≠ n, Tm = 1/n and Tn = 1/m, then a - d equals-
[AIEEE- 2004]
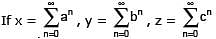 where a, b, c are in A.P. and I a I < 1, l b I < 1, I c I < 1 then x, y, z are in -
where a, b, c are in A.P. and I a I < 1, l b I < 1, I c I < 1 then x, y, z are in -
[AIEEE- 2005]
The point on y-axis equidistant from the points (3,2) and (-1,3) is
For a line whose equation is √3x + y = 8, the length of the perpendicular from the origin is
IQ of a person is given by the formula where MA is mental age and CA is the chronological age. If
for a group of 12 years old children, find the range of their mental age.
In a game a person wins a TV if in four throws of a dice he get sum greater than 20 .In three throws he got numbers as 5,3,6. What should be his fourth throw so that he wins a TV?
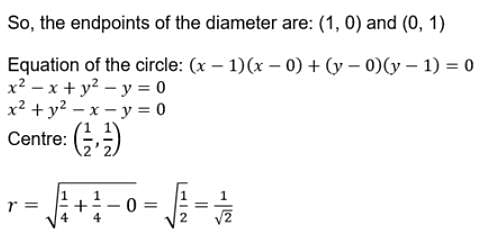
Centre and radius of the circle with segment of the line x + y = 1 cut off by coordinate axes as diameter is
Slopes of tangents through (7, 1) to the circle x2 + y2 = 25 satisfy the equation
Directrix of a parabola is x + y = 2. If it's focus is origin, then latus rectum of the parabola is equal to
Which one of the following equations represents parametrically, parabolic profile ?
If the distance of a point on the ellipse +
= 1 from the centre is 2, then the eccentric angle is
A tangent having slope of - 4/3 to the ellipse +
= 1 intersects the major & minor axes in points A & B respectively. If C is the centre of the ellipse then the area of the triangle ABC is
Let P(a secθ, b tanθ) and Q(a secφ, b tanφ) where θ+φ = π/2, be two points on the hyperbola 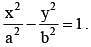 If (h, k) is the point of the intersection of the normals at P and Q, then k is equal to
If (h, k) is the point of the intersection of the normals at P and Q, then k is equal to
Foot of normals drawn from the point p(h,k) to the hyperbola will always lie on the conic
|
2 docs|101 tests
|
|
2 docs|101 tests
|


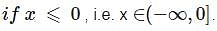
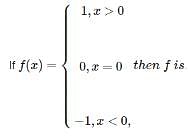
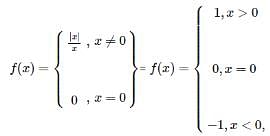
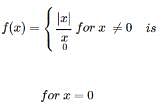
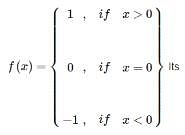
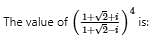

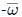
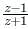 is purely imaginary,
is purely imaginary,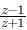 = a + ib, then a = 0
= a + ib, then a = 0
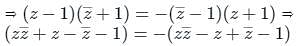

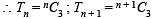
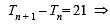
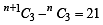
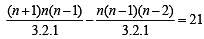
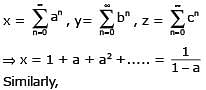
 .........(ii)
.........(ii) are in AP.
are in AP.