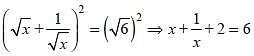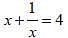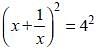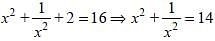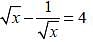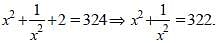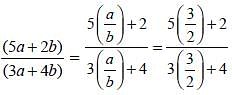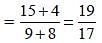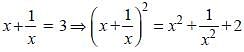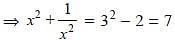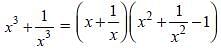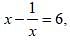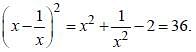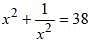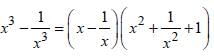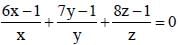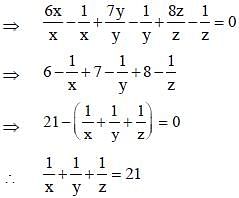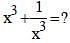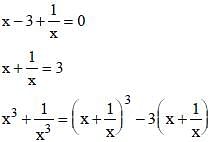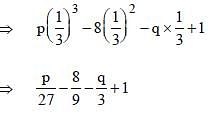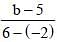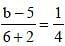SSC CGL Previous Year Questions: Algebra- 1 - SSC CGL MCQ
20 Questions MCQ Test SSC CGL Mathematics Previous Year Paper (Topic-wise) - SSC CGL Previous Year Questions: Algebra- 1
If (5a – 3b) : (4a – 2b) =2 : 3, then a : b is equal to: (SSC CGL-2018)
If a – b = 5 an d ab = 6, then (a3 – b3) is equal to: (SSC CGL-2018)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If a + b + c = 10 and ab + bc + ca = 32 then a3 + b3 + c3 – 3abc is equal to: (SSC CGL-2018)
If  then x2 + (1/x2) is equal to: (SSC CGL-2018)
then x2 + (1/x2) is equal to: (SSC CGL-2018)
If a : b = 3 : 2, then (5a + 2b) : (3a + 4b) is equal to: (SSC CGL-2018)
If a + b + c = 13 and ab + bc + ca = 54, then a3 + b3 + c3 – 3abc is equal to: (SSC CGL-2018)
If x + (1/x)= 3, then x3 + (1/(x3)) is equal to: (SSC CGL-2018)
If a2 + b2 = 169, ab = 60, (a > b), then (a2 – b2) is equal to : (SSC CHSL-2018)
If (2x + 3)3 + (x – 8)3 + (x + 13)3 = (2x + 3) (3x – 24) (x +13), then what is the value of x? (SSC Sub. Ins. 2018 )
If a3 + b3 = 5824 and a + b = 28, then (a – b)2 + ab is equal to: (SSC Sub. Ins. 2018 )
If x – (1/x) = 6, then x3 – (1/x3) is equal to: (SSC Sub. Ins. 2018 )
If 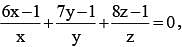 then what is the value of
then what is the value of 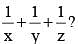 (SSC Sub. Ins. 2017)
(SSC Sub. Ins. 2017)
If x2 – 3x + 1 = 0, then what is the value of 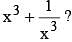 (SSC Sub. Ins. 2017)
(SSC Sub. Ins. 2017)
If a = 2017, b = 2016 and c = 2015, then what is the value of a2 + b2 + c2 – ab – bc – ca? (SSC Sub. Ins. 2017)
If the expression (px3 – 8x2 – qx + 1) is completely divisible by the expression (3x2 – 4x + 1), then what will be the value of p and q respectively? (SSC Sub. Ins. 2017)
If (p2 + q2) / (r2 + s2) = (pq) / (rs), then what is the value of (p – q) (p + q) in terms of r and s? (SSC Sub. Ins. 2017)
If 'a' and 'b' are positive integers such that a2 – b2 = 19, then the value of 'a' is : (SSC MTS 2017)
The line passing through (–2, 5) and (6, b) is perpendicular to the line 20x + 5y = 3. Find b? (SSC CHSL 2017)
If x – y = 6 and xy = 40, then find x2 + y2? (SSC CHSL 2017)
|
21 docs|55 tests
|
|
21 docs|55 tests
|


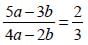
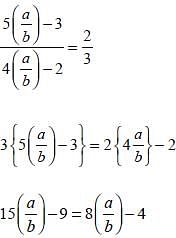
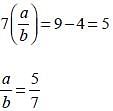
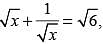 then
then 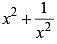 is equal to: (SSC CGL-2018)
is equal to: (SSC CGL-2018)