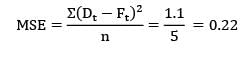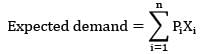Test: Forecasting Level - 2 - Mechanical Engineering MCQ
10 Questions MCQ Test - Test: Forecasting Level - 2
Demand for the month of January is given as 200; February 50; March 150. If a 9 month moving average method is used for forecasting and the forecasted value for the month of January is 100 units, then the forecast for the month of March using Exponential smoothening method is ___________
A hospital has used a 9-month moving average forecasting method to forecast drug and dressing inventory requirements. The actual demand for one item is as shown in the following table. Using previous moving average data, convert to an exponential forecast and find the forecast for the month 33.
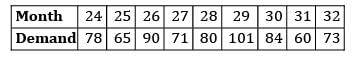

The demand and forecasted values for a new product are given in the table below.
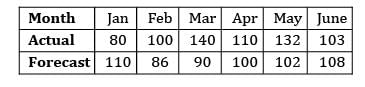
Tracking signal value is

The demand for 7 weeks is as follows 90, 93, 96, 99, 102, 105, 108
‘X’ is the forecasted demand for the 8th week using 3 month moving average method. ‘Y’ is the forecasted demand for the 8th week using 4 month moving average method. ‘Z’ is the forecasted demand for the 8th week using regression analysis. What would be the relation between X, Y, Z?
In a time series forecasting model, the demand for five time periods was 10, 13, 15, 18 and 22. A linear regression fit resulted in an equation F = 6.9 + 2.9 t where ‘F’ is the forecast for ‘t’ period. The mean squared error is ____________
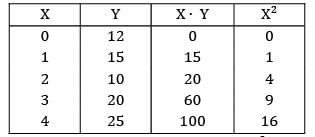
Consider the following data
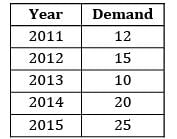
Find the forecast for the year 2016 using least squares method.
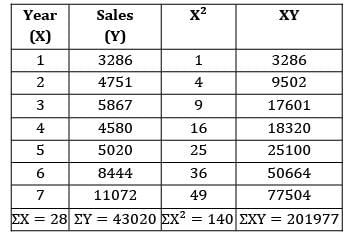
Historical data on the sales of cars for the year 2010 to 2016 is given below Find the forecast for the year 2017 using trend line method.

A company forecasts the future demand with “Regression analysis”. The following data is given n = 8; ΣX = 260; ΣY = 420; ΣXY = 14, 900 ΣX2 = 9750; ΣY2 = 23266
The forecast for 10th period is
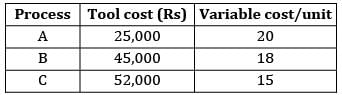
A company is introducing new product.It contains three alternatives to make the product with the following costs.
The forecasted demand estimates are as follows:
The minimum total cost is ____________
A company has forecasted the demand value for the month of June as 150 units. The smoothening constant is given as 0.2. The demand values for the month of June, July and August are constant and is given as 140 units. Determine the mean forecast error


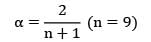
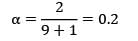

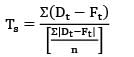
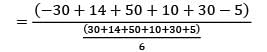
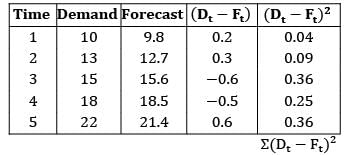 = 1.1
= 1.1