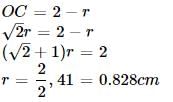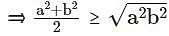Test: Geometry- 2 - CAT MCQ
15 Questions MCQ Test Quantitative Aptitude (Quant) - Test: Geometry- 2
A square is inscribed in a semi circle of radius 10 cm. What is the area of the inscribed square? (Given that the side of the square is along the diameter of the semicircle.)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Two circles of an equal radii are drawn, without any overlap, in a semicircle of radius 2 cm. If these are the largest possible circles that the semicircle can accommodate, what is the radius (in cm) of each of the circles?
PQRS is a Trapezzium, in which PQ is Parralel to RS, and PQ = 3 (RS) . The diagnol of the Trapezzium intersect each other at X, then the ratio of, ar ( ∆ PXQ) : ar ( ∆ RXS) is?
Let ABCDEF be a regular hexagon. What is the ratio of the area of the triangle ACE to that of the hexagon ABCDEF?
A pond 100 m in diameter is surrounded by a circular grass walk-way 2 m wide. How many square metres of grass is the on the walk-way?
The dimensions of a rectangular box are in the ratio of 1:2:4 and the difference between the costs of covering it with the cloth and a sheet at the rate of Rs 20 and Rs 20.5 per sq m respectively is Rs 126. Find the dimensions of the box.
The ratio of the area of a square inscribed in a semicircle to that of the area of a square inscribed in the circle of the same radius is
The ratio of the area of a square to that of the square drawn on the its the diagonal is
What is the area of the triangle in which two of its medians 9 cm and 12 cm long intersect at the right angles?
Four horses are tethered at four comers of a square plot of side 14 m so that the adjacent horses can just reach one another. There is a small circular pond of area 20 m2 at the centre. Find the ungrazed area.
Two sides of a triangle are 4 and 5. Then, for the area of the triangle, which one of the following bounds is the sharpest?
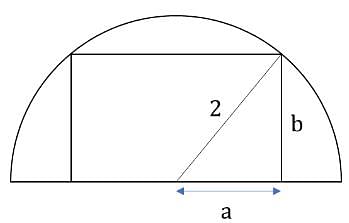
A rectangle with the largest possible area is drawn inside a semicircle of radius 2 cm. Then, the ratio of the lengths of the largest to the smallest side of this rectangle is?
The lengths of all four sides of a quadrilateral are integer valued. If three of its sides are of length 1 cm, 2 cm and 4 cm, then the total number of possible lengths of the fourth side is
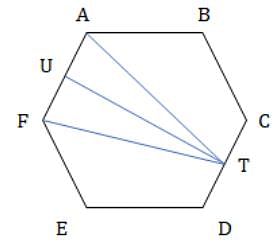
Suppose the length of each side of a regular hexagon ABCDEF is 2 cm. If T is the mid point of CD, then the length of AT, in cm, is
|
191 videos|131 docs|110 tests
|
|
191 videos|131 docs|110 tests
|