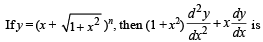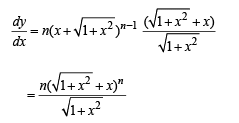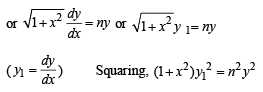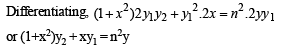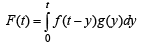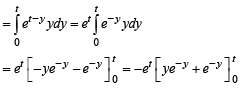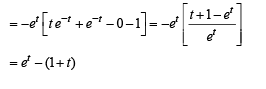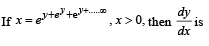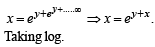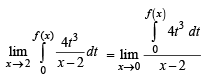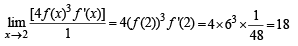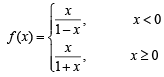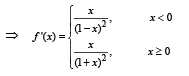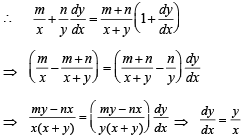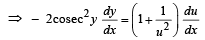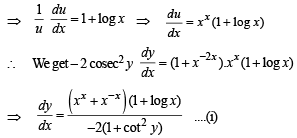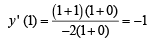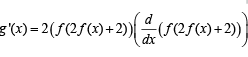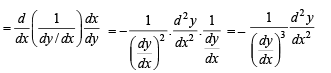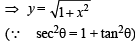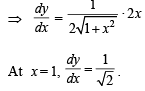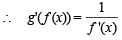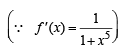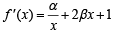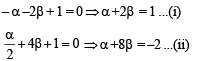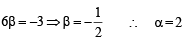Test: JEE Main 35 Year PYQs- Differentiation - Airforce X Y / Indian Navy SSR MCQ
16 Questions MCQ Test Mathematics for Airmen Group X - Test: JEE Main 35 Year PYQs- Differentiation
If f ( y) = ey , g (y) = y; y > 0 and
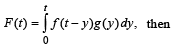
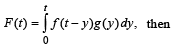
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Let f(x) be a polynomial fun ction of second degree. If f(1) = f (– 1) and a, b, c are in A. P , then f '(a), f '(b), f '(c) are in
The value of a for which the sum of the squares of the roots of the equation x2 – (a – 2) x – a – 1 = 0 assume the least value is
If the roots of th e equation x2 – bx + c = 0 be two consecutive integers, then b2 – 4c equals
Let f : R → R be a differentiable function having f (2) = 6,
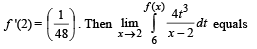
The set of points where 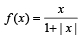 is differentiable is
is differentiable is
If xm. yn = (x+y) m+n , then dy/dx is
Let y be an implicit function of x defined by x2x – 2xx cot y – 1= 0. Then y'(1) equals
Let f : (–1, 1) → R be a differentiable function with f(0) = – 1 and f '(0) = 1. Let g(x) = [f (2f (x) + 2)]2. Then g'(0) =
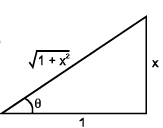
If y = sec(tan–1x), then dy/dx at x = 1 is equal to :
If g is the inverse of a function 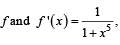 then g ' (x) is equal to:
then g ' (x) is equal to:
If x = –1 and x = 2 are extreme points of f (x) = a log |x| + βx2+x then
|
149 videos|192 docs|197 tests
|
|
149 videos|192 docs|197 tests
|