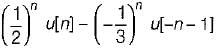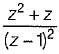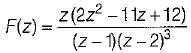Test: Z-Transform- 2 - Electrical Engineering (EE) MCQ
20 Questions MCQ Test Topicwise Question Bank for Electrical Engineering - Test: Z-Transform- 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The z-transform of discrete time signal x[n] = {1, 0, -1,0, 1,-1} is
A all pass causal LTI system is described by the difference equation:
y[n] - ay[n - 1] = bx[n] + x [n - 1]
The value of ’b’ will be
Consider a discrete time signal x(n) having z-transform given by 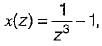 if x(n) is right sided then z-transform x(z) belongs to
if x(n) is right sided then z-transform x(z) belongs to
The sequence x[n] corresponding tox (z) defined as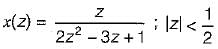 will have the value at n = - 2
will have the value at n = - 2
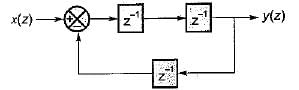
The direct.form-II realisation of []R filter is shown below.
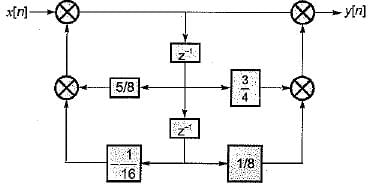
The transfer function is
A sequence of x(n) with the z-transform x(z) = z4 + z2 - 2z + 2 - 3z-4 is applied as an input to a L.T.I system with the impulse response 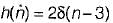 where,
where, 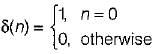 the output at n = 2 is
the output at n = 2 is
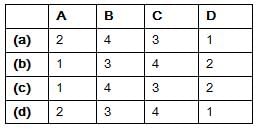
Match List-I {x(n)} with List-II {x(z)} and select the correct answer using the codes given below the lists:
List-I
A. ∝n u(n)
B. ∝n u(-n -1)
C. ∝n u(-n-1)
D. ∝n u(n)
List-II
1. 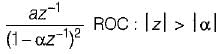
2.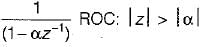
3.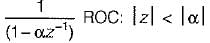
4.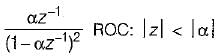
Codes
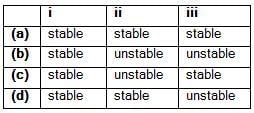
Choose the correct option for given causal systems, stable or not
i. 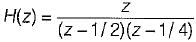
ii. 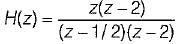
iii.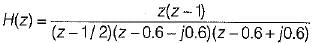
Consider discrete time signal x(n) is given by
x(n) = 4n u(n)
Also, 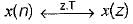
Consider another signal 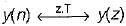
Also, y(z) = x2(z)
then, y(n) is
Consider a z-transform of a signal x(n)
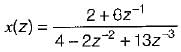
value of x(1) is equal to
Consider a discrete time signal x(n), who use z-transform x(z) is given by,
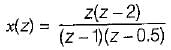
value of x(n) for n = 0 is
If z-transform is given by x(z) = cos(z-3), |z| > 0 the value of x[12] is


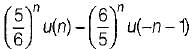 must be
must be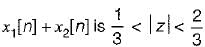 then the ROC of x1[n]-x2[n] includes
then the ROC of x1[n]-x2[n] includes