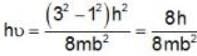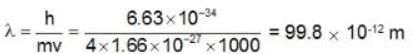IIT JAM Physics MCQ Test 10 - Physics MCQ
30 Questions MCQ Test IIT JAM Physics Mock Test Series 2025 - IIT JAM Physics MCQ Test 10
If a beam of electrons impinges on an energy barrier of height 0.03 eV and of infinite width. Find the fraction of electrons reflected and transmitted at the barrier if the impinging electrons
are having energy 0.04 eV.
are having energy 0.04 eV.
The probability of finding a particle inside the classical limits for an oscillator in its normal state is
The expected value of P for the wave function 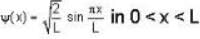 is given
is given
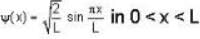 is given
is givenIn rectangular box with sides L and 2L, the two states |2, 8 > and | 4, 4 > have
The energy of a linear harmonic oscillator in third excited state is 0.1 eV. Then what is the frequency of vibration.
The wavefunctions of two identical particles in stated n and s are given by 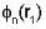 and
and 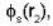 respectively. The particles obey Maxwell-Boltzmann statistics. The state of the combined two-particle system is expressed as
respectively. The particles obey Maxwell-Boltzmann statistics. The state of the combined two-particle system is expressed as
Two identical particles have to be distributed among three energy levels. Let rB, rr and rc represent the ratios of probability of finding two particles to that of finding one particle in a given energy state. The subscripts B. F and C correspond to whether the particles are bosons, fermions and classic particles, respectively. The, rB : rF: rc is equal to
Ratio of the radius of 79Au197 nucleus with the radius of its innermost Bohr orbit is
What is the value of distance of closest approach of a 3 MeV proton to a gold nucleus.
Find the binding energy of lithium nucleus for the given data
Mp= 1.00814 a.m.u
Mn = 1.0089 a.m.u.
MLi = 7.01822 a.m.u.
The electronic energy levels in a hydrogen atom are given by by En = - 13.6/n2 eV. If a selective excitation to the n = 50 level is to be made using a laser, the maximum allowed frequency line - width of the laser is
A particle of mass m is confined in the potential
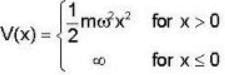
let the wave function of the particle be given by
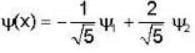
where 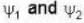 are the eigenfunction s of the first excite d state and the second excited state respectively. The expectation value of the energy is
are the eigenfunction s of the first excite d state and the second excited state respectively. The expectation value of the energy is
The normalized ground state wavefunction of a hydrogen atom is given by  where a is the Bohr radius and r is the distance of the electron from the nucleus, located, at the origin. The expectation value
where a is the Bohr radius and r is the distance of the electron from the nucleus, located, at the origin. The expectation value 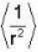 is
is
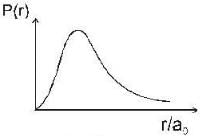
The ground state wavefunction for the hydrogen atom is given by 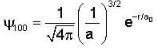 where a0 is the Bobr radius. The plot of the radial probability density, P(r) for the hydrogen atom in the ground state is
where a0 is the Bobr radius. The plot of the radial probability density, P(r) for the hydrogen atom in the ground state is
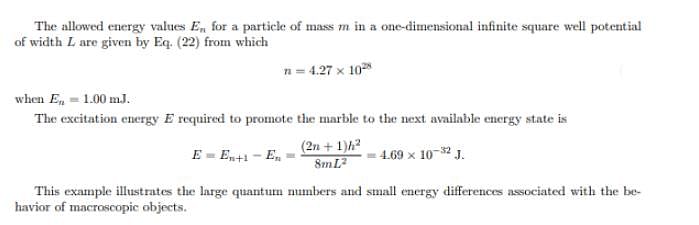
A 1.00 g marble is constrained to roll inside a tube of length L = 1.00 cm. The tube is capped at both ends. Modelling this as a one-dimensional infinite square well, determine the value of the quantum number n if the marble is initially given an energy of 1.00 mJ. and then Calculate the exitation energy required to promote the marble to the next available energy state.
and the exitation energy is A x 10-32 J. The value of A is.
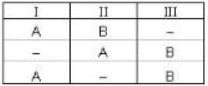
Which of the following nuclear reactions are possible?
A particle of mass m0 moves with speed c/√2, then its
A rod has length 100 cm. when the rod is in a satellite moving with a velocity that is one-half of the velocity of light relative to the laboratory, then
If the momentum of an electron is 10 times its rest mass times the speed light, then
A particle of rest mass m is moving with a with a velocity 0.9c; then
Let 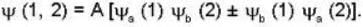 Find the real value of A which normalises
Find the real value of A which normalises 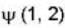 in this equation.
in this equation.
An electron has a speed of 100 m/s with an accuracy of 0.005%. The uncertainty in position will be in x meter,
the value of x is ?
Sum of all Eigen values of  (pauli spin matrix) are___.
(pauli spin matrix) are___.
A particle is described by the wave function 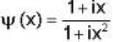 where is the particle most likely to be found?
where is the particle most likely to be found?
Determine the time [in day as measured by a clock at rest (on the rocket) taken by a rocket to reach a distant star and return to earth with a constant velocity 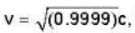 if the distance to the star is 4 light years (A light year is defined as the distance travelled by a light beam in vacuum in one year).
if the distance to the star is 4 light years (A light year is defined as the distance travelled by a light beam in vacuum in one year).
Consider a system of eight non interacting, identical quantum particles of spin -3/2 in a one dimensional box of length L. The minimum excitation energy of the system , in units of 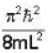 is____.
is____.
A Two- dimensional square rigid box of side L contains eight non-interacting electrons at T = ok. The mass of the electron is m. The ground state energy of the system of electrons, in units of  is ____.
is ____.
An electron of mass m is confined to a one-dimensional box of length b. If it makes a radiative transition from second excited state to the ground state, the frequency of the photon emitted is _____
The de-Broglie wavelength for a He atom travelling at 1000 m/5 (typical speed at room temperature) i s __________ x10-12m•
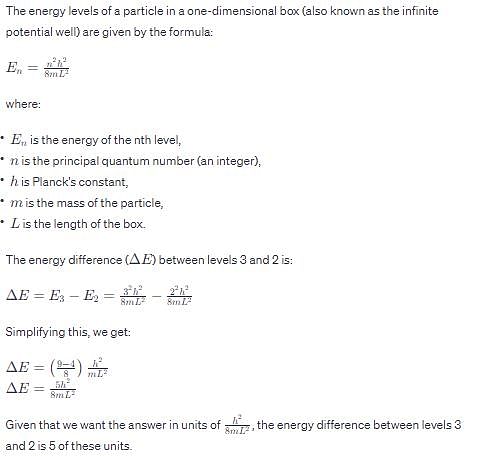
ln units of 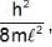 the energy difference between levels corresponding to 3 and 2 node dimensional box of length l is ______.
the energy difference between levels corresponding to 3 and 2 node dimensional box of length l is ______.
|
4 docs|21 tests
|



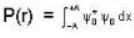 ...(I)
...(I)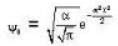
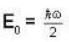 and classically, the total energy of the particle is
and classically, the total energy of the particle is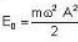 (where A-Amplitude)
(where A-Amplitude)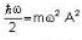
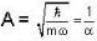
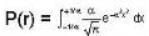

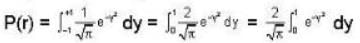

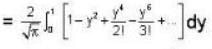
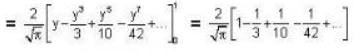

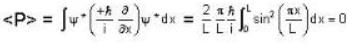
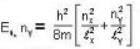 ...(i)
...(i)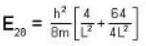
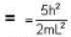 ...(ii)
...(ii)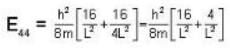
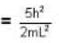 ...(iii)
...(iii)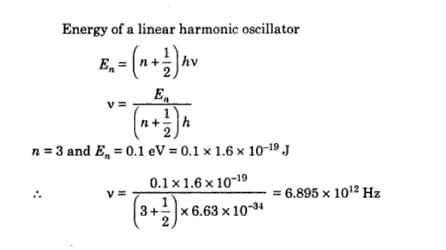
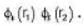
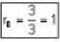 ...(i)
...(i)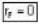 ...(ii)
...(ii)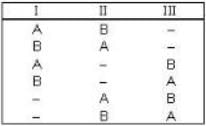
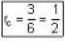 ...(iii)
...(iii)
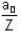


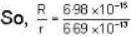
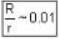
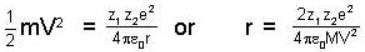
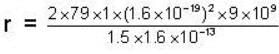 = 1.5 x 10-13m
= 1.5 x 10-13m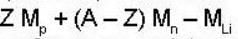
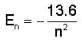
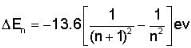
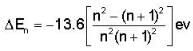
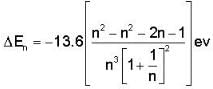
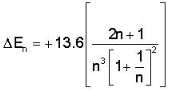
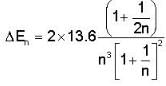
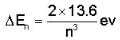
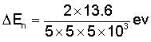
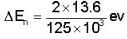
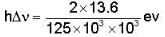
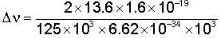
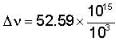
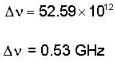

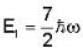
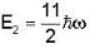

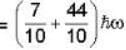
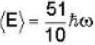
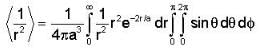
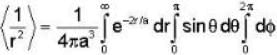
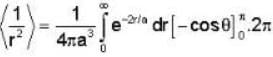
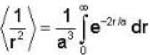
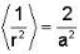
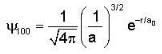
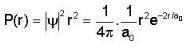

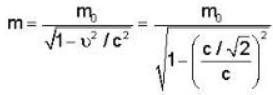
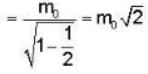
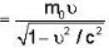
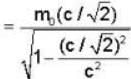
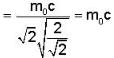
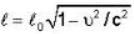

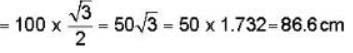
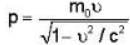
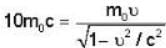
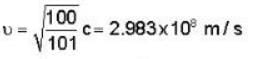
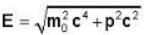
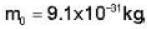
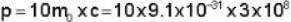
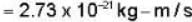
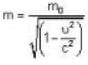
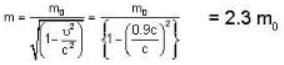
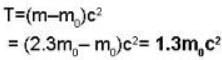
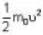 is correct only for low velocities which may be verified as follows
is correct only for low velocities which may be verified as follows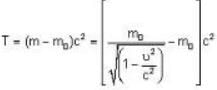
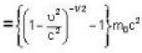

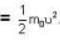
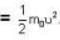
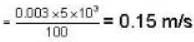
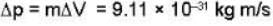

 or
or 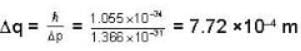
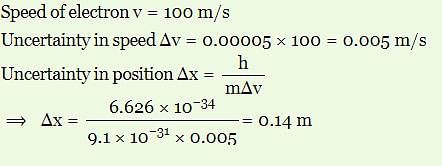
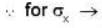
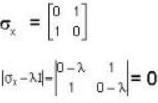
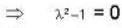
 Ans.
Ans.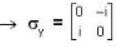
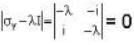
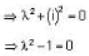
 Ans.
Ans.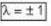
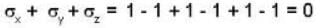
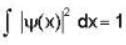
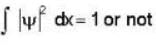
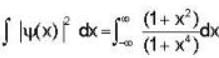
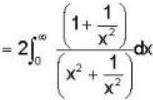
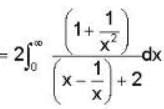
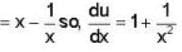
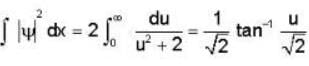
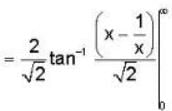

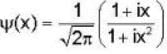
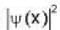 is maximum.
is maximum.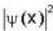 is maximum where
is maximum where 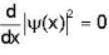
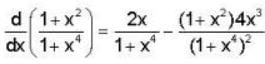
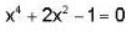
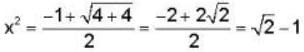
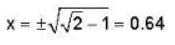

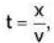 where
where 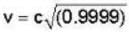
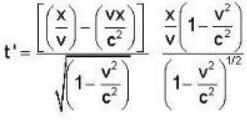
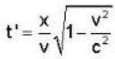 ...(ii)
...(ii)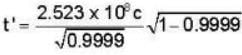
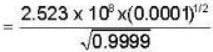
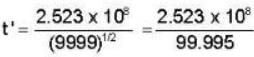
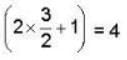
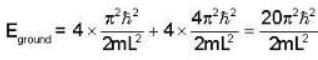
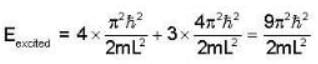
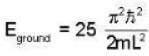

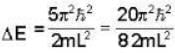
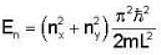
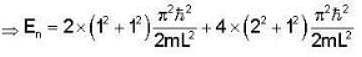
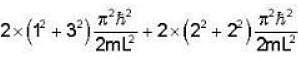
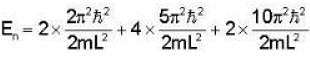

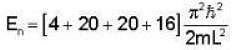
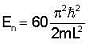

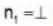 and for second state n2 = 3
and for second state n2 = 3