Test: Parabola - 1 - JEE MCQ
10 Questions MCQ Test Mathematics (Maths) for JEE Main & Advanced - Test: Parabola - 1
The equation of parabola whose focus is (– 3, 0) and directrix x + 5 = 0 is:
A parabola whose axis is along the y-axis, vertex is (0,0) and point from the first and second quadrants lie on it, has the equation of the type
The equation y2 + 3 = 2(2x+y) represents a parabola with the vertex at
The equation of the parabola with vertex at (0, 0) and focus at (0, – 2) is:
The …… of a conic is the chord passing through the focus and perpendicular to the axis.
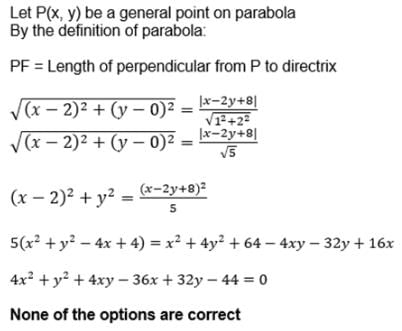
The equation of the parabola with vertex at (1, 2) and focus at (2, 0) is:
The equation of the parabola with focus at (a,0) where a>0 and directrix x = -a is
If the focus of a parabola is (-2,1) and the directrix has the equation x + y = 3 then the vertex is
Any point on the parabola whose focus is (0,1) and the directrix is x + 2 = 0 is given by
|
176 videos|582 docs|160 tests
|
|
176 videos|582 docs|160 tests
|