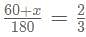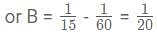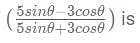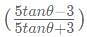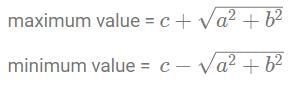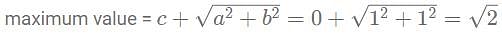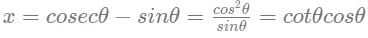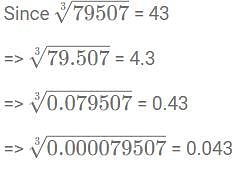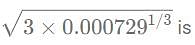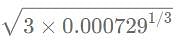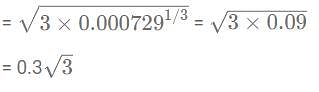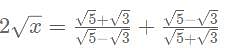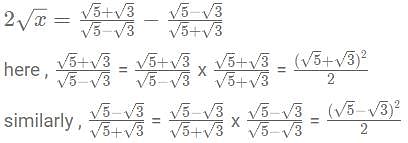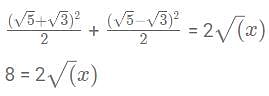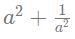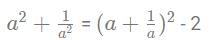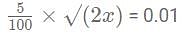Practice Test: Quantitative Aptitude - 14 - SSC CGL MCQ
25 Questions MCQ Test SSC CGL Tier 1 Mock Test Series 2025 - Practice Test: Quantitative Aptitude - 14
Directions: The following pie chart shows the marks scored by a student in different subjects- viz. Physics (Ph), Chemistry (Ch), Mathematics (M), Social Science (SS) and English (E) in an examination. Assuming that total marks obtained for the examination is 810, answer the questions given below.
The marks obtained in English, Physics and Social Science exceed the marks obtained in Mathematics and Chemistry by
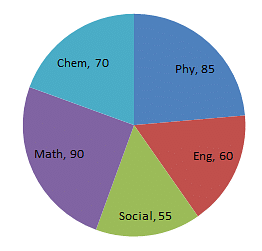
The difference of marks between Physics and Chemistry is same as that between

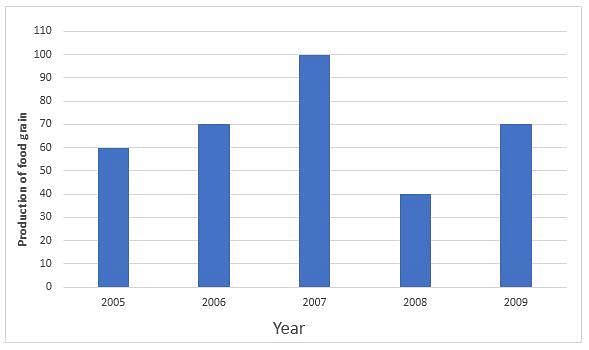
Study the above bar graph showing the production of food grains (in million tons). What is the ratio between the maximum production and the minimum production during the given period?
Graph should be drawn
Graph should be drawn
Rs. 700 is divided among A, B, C in such a way that the ratio of the amounts of A and B is 2 : 3 and that of B and C is 4 : 5. Find the amounts in Rs. each received, in order A, B, C.
ABC is a right angled triangle, B being the right angle. Mid-points of BC and AC are respectively B’ and A’. The ratio of the area of the quadrilateral AA’ B’B to the area of the triangle ABC is
The ratio of the volumes of water and glycerine in 240cc of a mixture is 1 : 3. The quantity of water (in cc that should be added to the mixture so that the new ratio of the volume of water and glycerine becomes 2 : 3 is
If the distance between two point (0, -5) and (x, 0) is 13 unit, then x =
A and B together can do a works in 12 days. B and C together do it in 15 days. If A's efficiency is twice that of C, then the days required for B alone to finish the work is
A drum of kerosene is 3/4 full. When 30 litres of kerosene is drawn from it, it remains 1/2 full. The capacity of the drum is
A rectangular sheet of metal is 40 cm by 15 cm. Equal squares of side 4 cm are cut off at the corners and the remainder is folded up to form an open rectangular box. The volume of the box is
Water flows into a tank which is 200m long and 150m wide, through a pipe of cross-section 0.3m × 0.2m at 20 km/hour. Then the time (in minutes) for the water level in the tank to reach 8cm is
If x = cosecθ − sinθ and y = secθ − cosθ, then the value of x2y2(x2 + y2 + 3)
The value of sin2 22° + sin2 68° + cot2 30° is
If the number of vertices, edges and faces of a rectangualr parallelopiped are denoted by v, e and f respectively, the value of (v - e + f) is
The average age of a jury of 5 is 40. If a member aged 35 resigns and man aged 25 becomes a member, then the average age of the new jury is
I am three times as old as my son. 15 years hence, I will by twice as old as my son. The sum of our ages is
If the cube root of 79507 is 43, then the value of 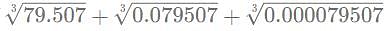 is
is
If √6 × √15 = x×√10, then the value of x is
If x + y = 15, then (x − 10)3 + (y − 5)3 is
If a + 1/a = 1, then the value of a2 + 1/a2 is:
The value of x when 5% of √2x is 0.01 will be:
If a * b = 2a + 3b - ab, then the value of (3 * 5 + 5 * 3) is
|
237 docs|133 tests
|