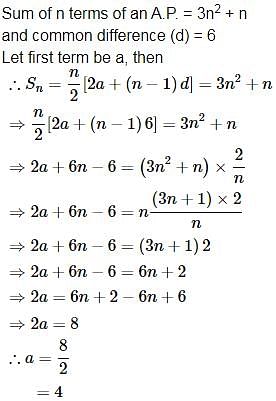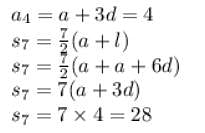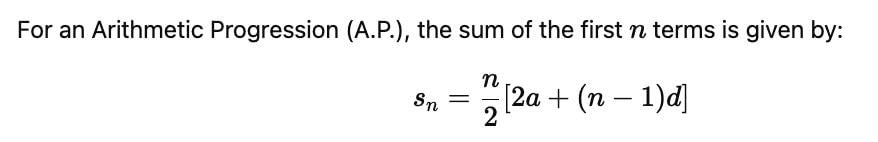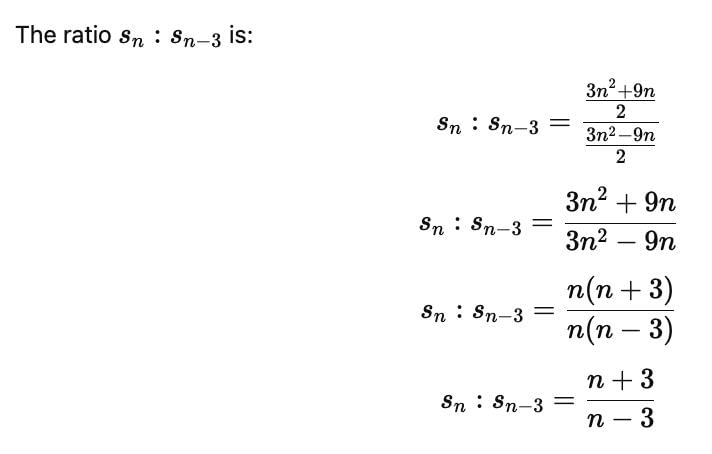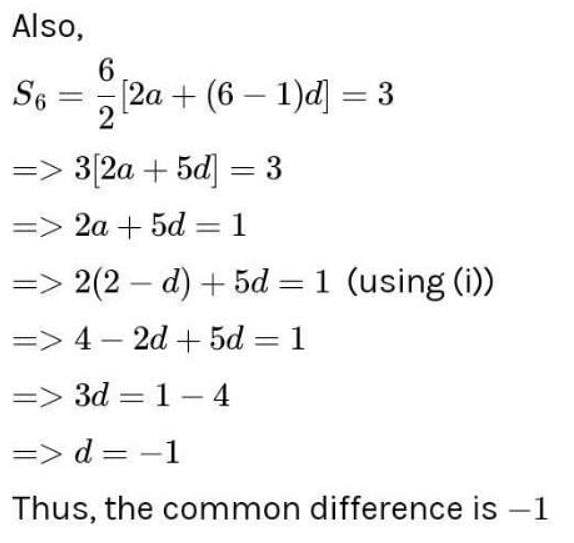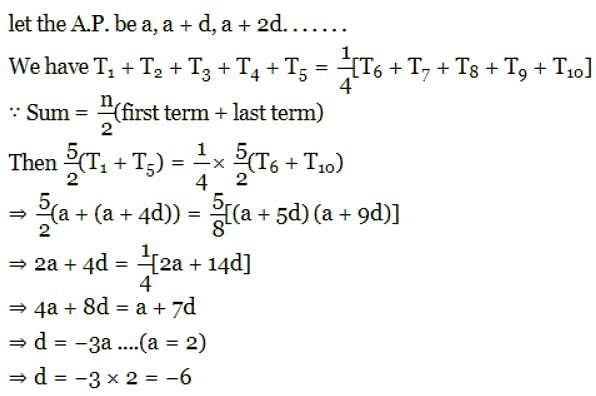Practice Test: Arithmetic Progressions - Class 10 MCQ
15 Questions MCQ Test Mathematics (Maths) Class 10 - Practice Test: Arithmetic Progressions
If the sum of first n terms of an AP be 3n2 + n and it's common difference is 6, then its first term is :
If 7th and 13th terms of an A.P. be 34 and 64, respectively, then it's 18th term is :
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The sum of all 2-digit odd positive numbers is :
The fourth term of an A.P. is 4. Then the sum of the first 7 terms is :
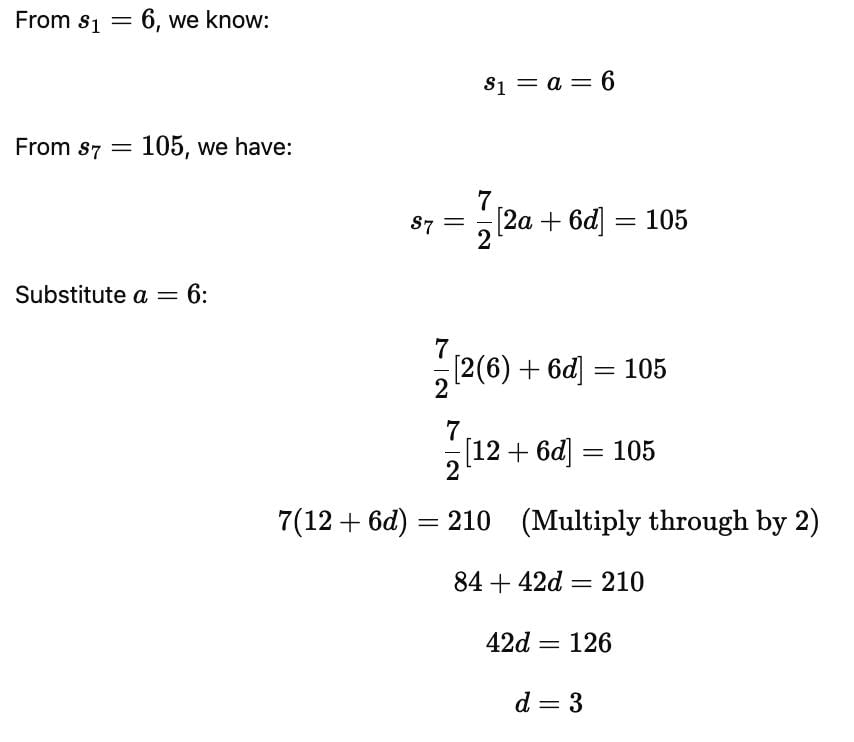
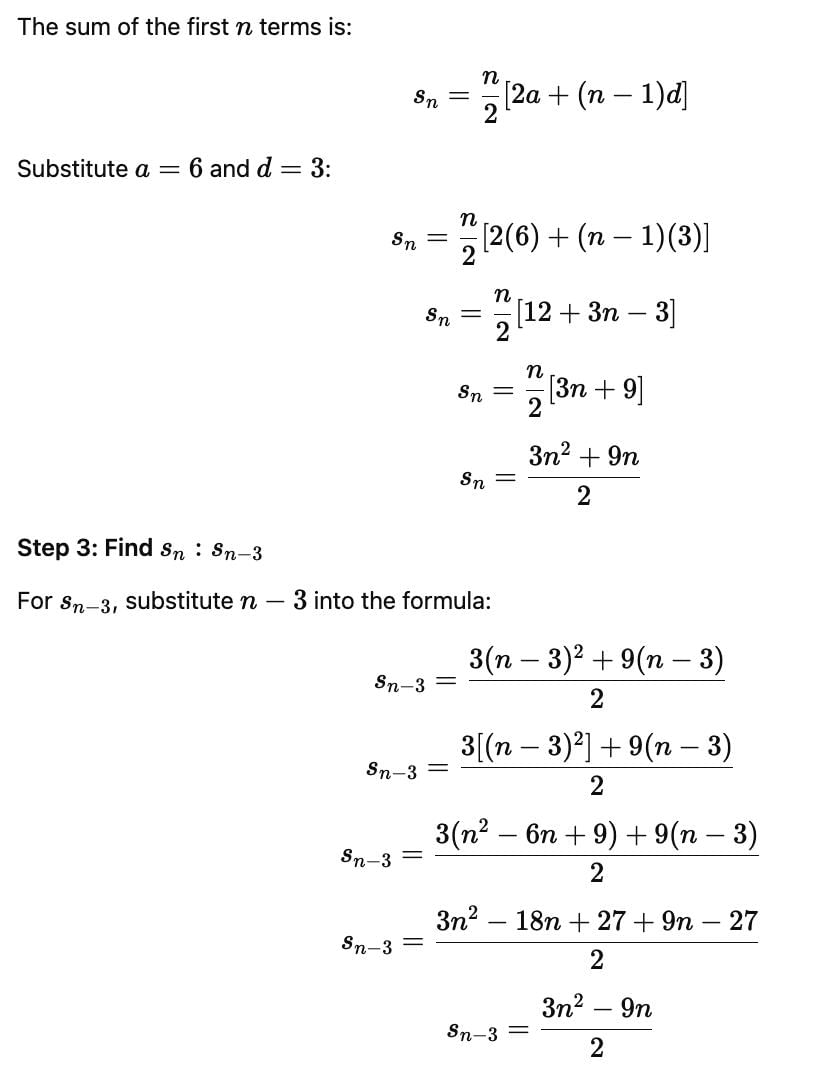
In an A.P., s1 = 6, s7 = 105, then sn : sn-3 is same as :
In an A.P. s3 = 6, s6 = 3, then it's common difference is equal to :
The number of terms common to the two A.P. s 2 + 5 + 8 + 11 + ...+ 98 and 3 + 8 + 13 + 18 +...+198
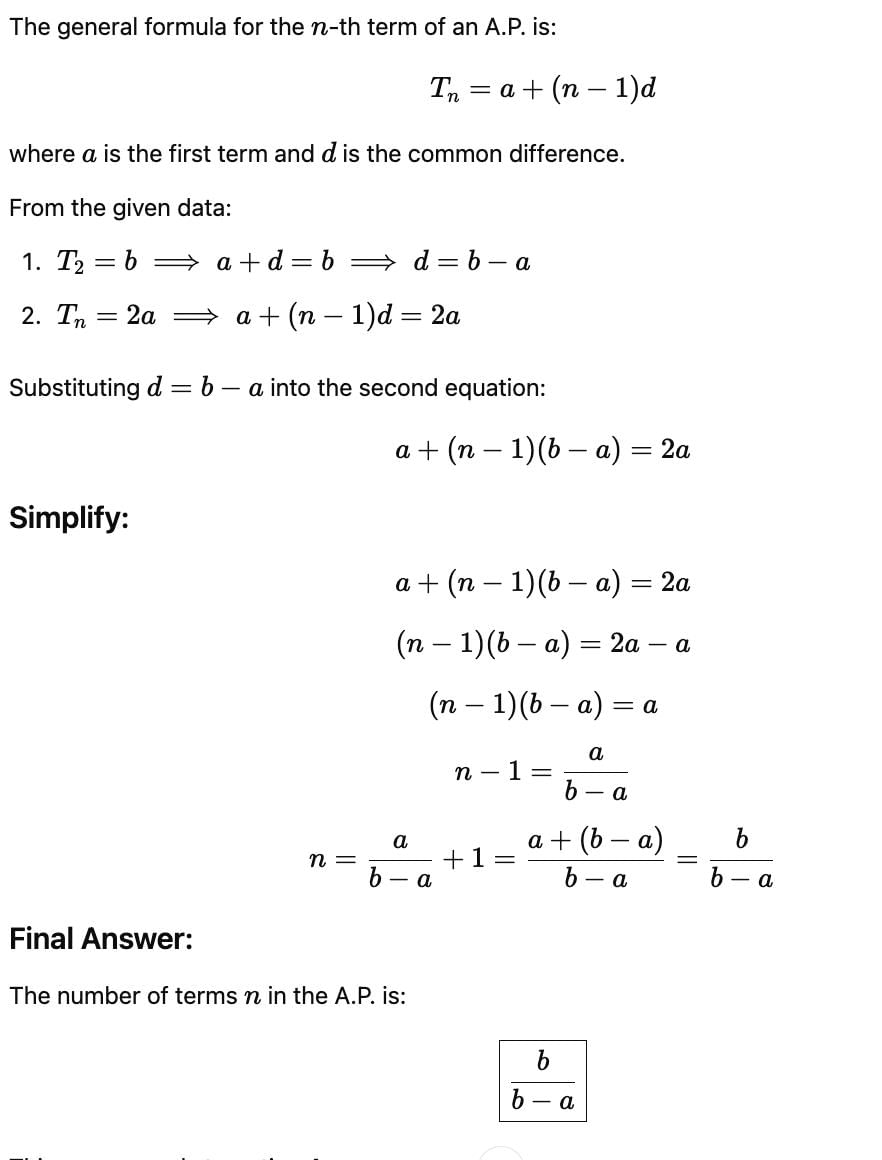
The first, second and last terms of an A.P. are a,b and 2a. The number of terms in the A.P. is :
Sum of first 5 terms of an A.P. is one fourth of the sum of next five terms. If the first term = 2, then the common difference of the A.P. is :
If sn denotes the sum of first n terms of an A.P., whose common difference is d, then sn – 2sn-1 + sn-2 (n >2) is equal to :
The sum of all 2-digited numbers which leave remainder 1 when divided by 3 is :
If {an} = {2.5, 2.51, 2.52,...} and {bn} = {3.72, 3.73, 3.74,...} be two AP's, then a100005 – b100005 =
If A1 and A2 be the two A.M.s between two numbers a and b, then (2A1 – A2) (2A2 – A1) is equal to :
In an Arithmetic Progression, if a = 28, d = -4, n = 7, then an is:
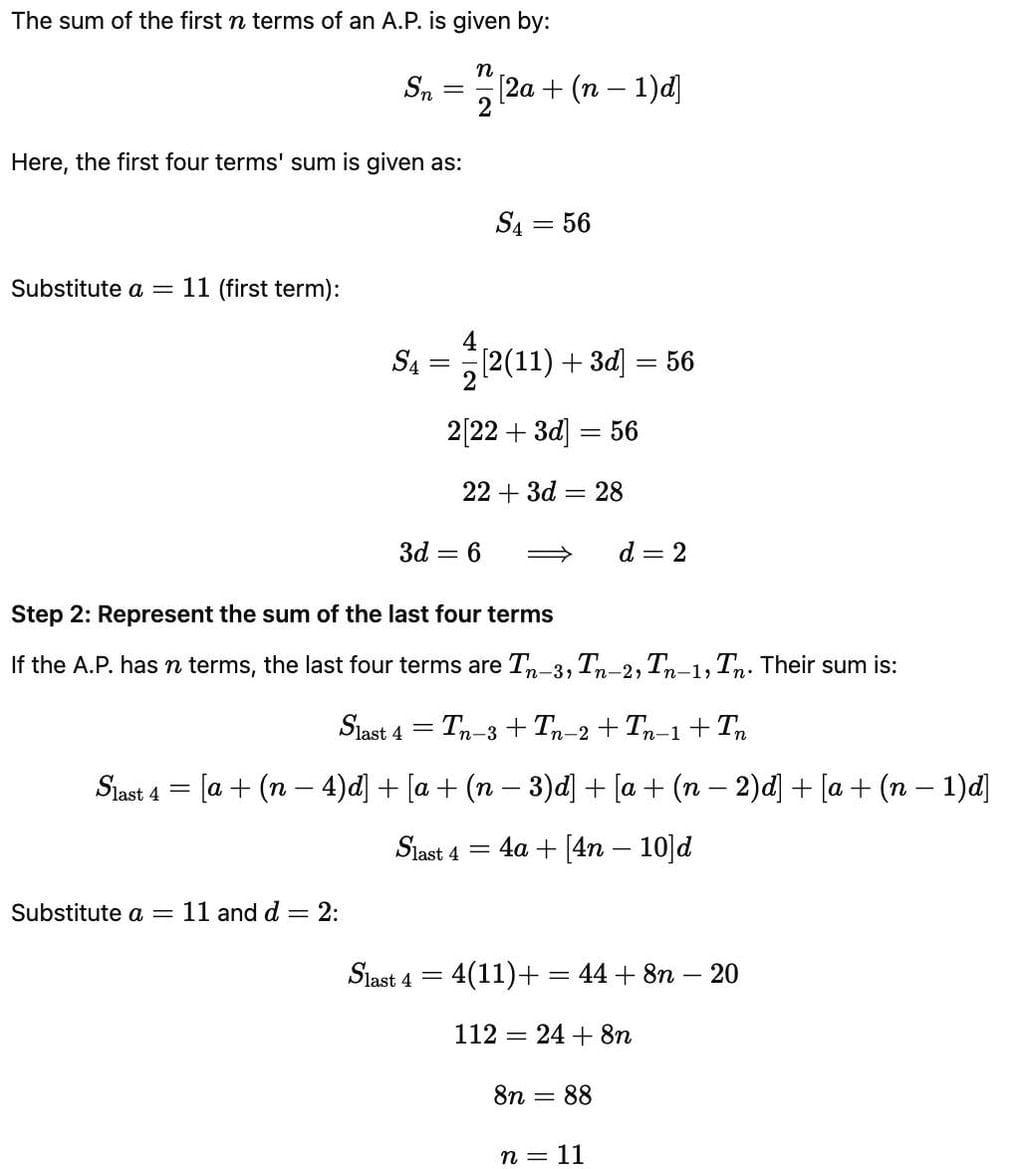
The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.
|
123 videos|457 docs|77 tests
|
|
123 videos|457 docs|77 tests
|