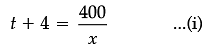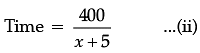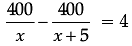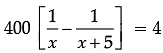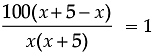Case Based Questions Test: Quadratic Equations - Class 10 MCQ
15 Questions MCQ Test Mathematics (Maths) Class 10 - Case Based Questions Test: Quadratic Equations
Read the following text and answer the following questions on the basis of the same:
Nidhi and Ria are very close friends. Nidhi’s parents own a Maruti Alto and Ria’s parents own a Toyota Liva. Both the families decided to go for picnic to Somnath temple in Gujarat by their own cars. Nidhi car travels x km/h when Ria’s car travels 5 km/h more than Nidhi’s car Nidhi’s car took 4 hours more than Ria’s car in covering 400 km.

Q. Which of the following quadratic equations describe the speed of Nidhi’s car?

Read the following text and answer the following questions on the basis of the same:
Nidhi and Ria are very close friends. Nidhi’s parents own a Maruti Alto and Ria’s parents own a Toyota Liva. Both the families decided to go for picnic to Somnath temple in Gujarat by their own cars. Nidhi car travels x km/h when Ria’s car travels 5 km/h more than Nidhi’s car Nidhi’s car took 4 hours more than Ria’s car in covering 400 km.

Q. How much time took Ria to travel 400 km?

| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Read the following text and answer the following questions on the basis of the same:
Nidhi and Ria are very close friends. Nidhi’s parents own a Maruti Alto and Ria’s parents own a Toyota Liva. Both the families decided to go for picnic to Somnath temple in Gujarat by their own cars. Nidhi car travels x km/h when Ria’s car travels 5 km/h more than Nidhi’s car Nidhi’s car took 4 hours more than Ria’s car in covering 400 km.

Q. What will be the distance covered by Ria’s car in two hours?

Read the following text and answer the following questions on the basis of the same:
Nidhi and Ria are very close friends. Nidhi’s parents own a Maruti Alto and Ria’s parents own a Toyota Liva. Both the families decided to go for picnic to Somnath temple in Gujarat by their own cars. Nidhi car travels x km/h when Ria’s car travels 5 km/h more than Nidhi’s car Nidhi’s car took 4 hours more than Ria’s car in covering 400 km.

Q. What is the speed of Nidhi’s car?
Read the following text and answer the following questions on the basis of the same:
Nidhi and Ria are very close friends. Nidhi’s parents own a Maruti Alto and Ria’s parents own a Toyota Liva. Both the families decided to go for picnic to Somnath temple in Gujarat by their own cars. Nidhi car travels x km/h when Ria’s car travels 5 km/h more than Nidhi’s car Nidhi’s car took 4 hours more than Ria’s car in covering 400 km.

Read the following text and answer the following questions on the basis of the same:
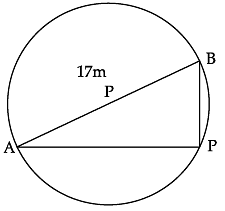
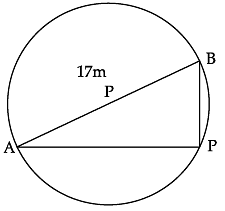
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7m.
Q. Find the distance between pole and gate B.Read the following text and answer the following questions on the basis of the same:
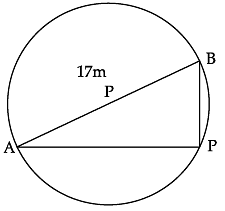
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7m.
Q. What is the length of (AP + BP)?
Read the following text and answer the following questions on the basis of the same:
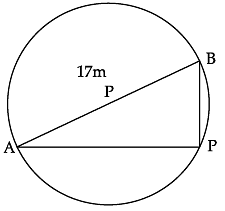
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7m.
Q. Find a quadratic equations in variable x for above situation.Read the following text and answer the following questions on the basis of the same:
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7m.
Q. Find the distance between pole and gate A.Read the following text and answer the following questions on the basis of the same:
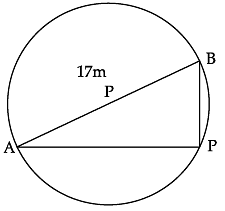
Aniket is studying in X standard. He is created a pole at on the boundary of a circular park of diameter 17 m in such a way that the difference of its distances from two diametrically opposite fixed gates A and B on the boundary is 7m.
Q. Find the area of triangle ABP.
Read the following text and answer the following questions on the basis of the same:
A small scale industry produces a certain boxes of candles in a day. Number of boxes prepared by each worked on a particular day was 2 more than thrice the number of workers working in the industry. The number of boxes produced in a particular day was 85.
Q. Represent the above equation in quadratic equation.
Read the following text and answer the following questions on the basis of the same:
A small scale industry produces a certain boxes of candles in a day. Number of boxes prepared by each worked on a particular day was 2 more than thrice the number of workers working in the industry. The number of boxes produced in a particular day was 85.
Q. Number of workers working in the industry:
Read the following text and answer the following questions on the basis of the same:
A small scale industry produces a certain boxes of candles in a day. Number of boxes prepared by each worked on a particular day was 2 more than thrice the number of workers working in the industry. The number of boxes produced in a particular day was 85.
Q. If the number of workers working in the industry is x. What was the number of boxes of candles prepared by each worker on that particular day?
Read the following text and answer the following questions on the basis of the same:
A small scale industry produces a certain boxes of candles in a day. Number of boxes prepared by each worked on a particular day was 2 more than thrice the number of workers working in the industry. The number of boxes produced in a particular day was 85.
Q. Nature of roots of the above quadratic equation are:
Read the following text and answer the following questions on the basis of the same:
A small scale industry produces a certain boxes of candles in a day. Number of boxes prepared by each worked on a particular day was 2 more than thrice the number of workers working in the industry. The number of boxes produced in a particular day was 85.
Q. How many boxes will be prepared when number of workers are increased by 2.
|
123 videos|457 docs|77 tests
|
|
123 videos|457 docs|77 tests
|