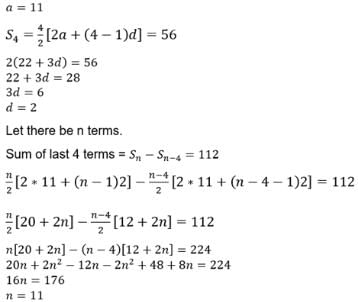Test: Sequences And Series- 2 - Commerce MCQ
25 Questions MCQ Test Online MCQ Tests for Commerce - Test: Sequences And Series- 2
If the numbers a, b, c, d, e form an A.P. then the value of a – 4b + 6c – 4d + e is
The sum of all non-reducible fractions with the denominator 3 lying between the numbers 5 and 8 is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The next term of the sequence 1, 1, 2, 4, 7, 13,…. Is
An A.P. consists of n (odd) terms and its middle term is m. Then the sum of the A.P. is
The terms equidistant from a given term of an A.P. are multiplied together, then the difference of the successive terms of the series so formed are in
If the numbers a, b, c are in A.P., b, c, d are in G.P. and c, d , e are in H.P., then a, c, e are in
If x, y, z are in A.P., then (x + 2y – z) (x + z – y) (z + 2y – x) is equal to
If a, b , c are in A.P. and also 1/a, 1/b, 1/c are A. P., then
The sum of terms equidistant from the beginning and end in A.P. is equal to
Sum of first 5 terms of an A.P. is one fourth of the sum of next five terms. If the first term = 2, then the common difference of the A.P. is
The first, second and last terms of an A.P. are a, b and 2 a. The number of terms in the A.P. is
The number of terms common to the two A.P.s 2 + 5 + 8 + 11 + … + 98 and 3 + 8+ 13 + 18+ 23 + … + 198
The values of x for which the solutions of the equation cos θ = x, θ ≥ 0 form an A.P. are
If a ∈ R, then the roots of the equation tan x = a are in
The values of ‘a’ for which the roots of the equation sin x = a in A.P. are
The sum of first four terms of an A. P. is 56 and sum of last four terms is 112. If the first term is 11, then the number of terms is
The ratio of the 7th to the (n –1)th mean between 1 and 31, when n arithmetic means are inserted between them, is 5 : 9. The value of n is
The sum of first n terms of the series 1 – 1 + 1 – 1 + … is
The product of first n odd terms of a G.P. whose middle term is m is
The fourth term of a G. P. is 2, then product of first 7 terms is
If three positive numbers a, b, c are in G. P. then log a, log b, log c are in
|
705 tests
|