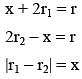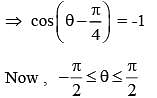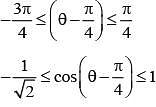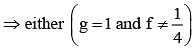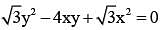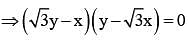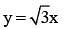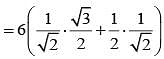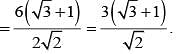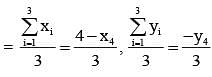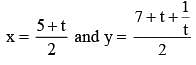Test: Circles - 4 - JEE MCQ
30 Questions MCQ Test Chapter-wise Tests for JEE Main & Advanced - Test: Circles - 4
The triangle PQR is inscribed in the circle x2 + y2 = 25. If Q and R have coordinates (3, 4) and (–4, 3) respectively, then ∠QPR may be equal to
If the circles x2 + y2 + 2ax + b = 0 and x2 + y2 + 2cx + b = 0 touch each other, then
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Two circles with radii ‘r1’ and ‘r2’, r1 > r2 > 2, touch each other externally. If ‘θ’ be the angle between the direct common tangents, then
The common chord of x2 + y2 – 4x – 4y = 0 and x2 + y2 = 16 subtends at the origin an angle equal to
A circle C and the circle x2 + y2 = 1 are orthogonal and have radical axis parallel to y-axis, then C can be
The tangents drawn from the origin to the circle x2 + y2 + 2gx + 2fy + f2 = 0 are perpendicular if
If one of the diameters of the circle x2 + y2 - 2x - 6y + 6 = 0 is a chord to the circle with centre (2, 1), then the radius of the circle is
Let A0 A1A2A3 A4A5 be a regular hexagon inscribed in a circle of unit radius. Then the product of the lengths of the line segments A0A1, A0 A2 and A0 A4 is
If the point (k + 1, k) lies inside the region bound by the curve and the y-axis, then k belong to the interval
A line meets the coordinate axes in A and B, and a circle is circumscribing triangle AOB where O is the origin. If m, n are the distances of the tangents to this circle at the origin from the points A and B respectively, then the diameter of the circle is
The locus of the centre of a circle which passes through the origin and cuts off a length 2b from the line x = c, is
If the curves ax2 + 4xy + 2y2 + x + y + 5 = 0 and ax2+ 6xy + 5y2+ 2 x + 3y + 8 = 0 intersect at four concyclic points then the value of a is
Equation of chord AB of circle x2 + y2 = 2 passing through P(2, 2) such that PB/PA = 3, is given by
If P(2, 8) is an interior point of a circle x2 + y2 –2x + 4y – p = 0 which neither touches nor intersects the axes, then set for p is
Radii of the smallest and the largest circle passing through a point lying on the sides of a rectangle with vertices (± 2, ± 1) and touching the circle x2 + y2 = 9, are r1 and r2 respectively. Let d = |r1 – r2| then minimum value of d is
If the point (2cosθ, 2sinθ) does not lie in the angle between the lines x + y = 2 and x -y = 2 in which the origin lies, then number of solutions of the equation √2 + cosθ + sinθ = 0 is
The radical axis of the two distinct circles x2 + y2 + 2gx + 2fy + c = 0 and 2x2 + 2y2 + 4x + y + 2c = 0 touches the circle x2 + y2 - 4x - 4y + 4 = 0. Then the centre of the circle x2 + y2 + 2gx + 2fy + c = 0 can be
Equation of a circle that cuts the circle x2 + y2 + 2gx + 2fy + c = 0, lines x = – g and y = –f orthogonally, is;
The range of values of α for which the line 2y = gx + a is a normal to the circle x2 + y2 + 2gx +2g y - 2 = 0 for all values of g is
The point of intersection of the tangents of the circle x2 + y2 = 10, drawn at end points of the chord x + y = 2 is
The line x + y = 5 intersects the circle x2 +y2 - 6x - 8y + 21 = 0 at points A and B, then the locus of the point C such that AC is perpendicular to BC is
If a circle of radius 3 units is touching the lines in the first quadrant then length of chord of contact to this circle is
(x – 1)(y – 2) = 5 and (x – 1)2 + (y + 2)2 = r2 intersect at four points A, B, C, D and if centroid of ΔABC lies on line y = 3x - 4, then locus of D is
Tangents PA and PB are drawn to circle (x - 5)2 + (y - 7)2 = 1 from point P lying on Locus of circumcentre of triangle PAB is
The point on the straight line x + y = 2, which is nearest to the circle x2 + y2 –10x + 2y + 22 = 0
If circumference of circle is 64π then area of circle (in terms of π) is
|
446 docs|930 tests
|
|
446 docs|930 tests
|


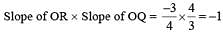
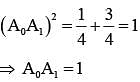
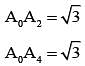
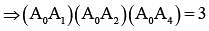

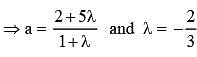
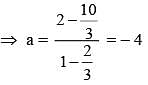
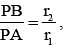 now if r1 = α, r2 = 3α then 4α
now if r1 = α, r2 = 3α then 4α