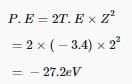Bohr's Theory Of Hydrogen Atom
19 Questions MCQ Test Chemistry Class 11 | Bohr's Theory Of Hydrogen Atom
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Because isotones means the same number of neutron, So, from the question, option c is right, number of neutron in k is,39-19= 20,and,the number of neutron in ca is also 40-20=20 so it is isotones.
‘Hartree’ is the atomic unit of energy and is equal to
The potential energy of an electron in the first Bohr’s orbit in the H-atom is
This energy is defined as an atomic unit of energy called HARTREE.
Wave number of a spectral line for a given transition is x cm-1 for He+, then its value for Be3+ (isoelectronic of He+)for the same transition is
Which of the following electronic transitions requires that the greatest quantity of energy be absorbed by a hydrogen atom ?
Therefore, electronic transition (a) requires greatest quantity of energy.
An electron in H-atom in its ground state absorbs 1.5 times as much as energy as the minimum required for its escape from the atom
Q.
Thus, kinetic energy given to the emitted electron is
E1 = Energy of H-atom in the ground state = 13.6 eV
Energy absorbed = (13.6 x 1.5) = 20.4 eV
E2 = Energy of the excited state
= 13.6+ 20.4= 34.0 eV
ΔE = KE = (E2 - E1)
= 34.0 - 13.6 = 20.4 eV
Ionisation energy of He+ is 19.6x10-18 J atom -1. The energy of the first stationary state (n = 1)of Li2+ is
Ionisation energy = - Energy of the electron
The kinetic energy of an electron in the second Bohr orbit of a hydrogen atom is (a0 is Bohr radius)
[AIEEE 2012]
Also, angular momentum is quantised
Energy of the electron in nth orbit is given by E 
Wavelength of light required to excite an electron in an H-atom from level n = 1 to n = 2 will be (h = 6.62 x 10-34 J s ; c = 3.0 x 108ms -1)
[AIEEE 2012]
For H - atom , Z = 1
The potential energy of an electron in the second Bohr's orbit of the he±
In Lyman series, shortest wavelength of H-atom appears at x m, then longest wavelength in Balmer series of He+ appear at
For the spectral line in H -atom and H -like species (one electron)
For Lyman series
For shortest wavelength (maximum wave number) n2 → ∞
For longest wave length (minimum wave number), n2 = (n1, + 1).
For Balmer series, n1 = 2
If the radius of the first Bohr orbit is x, then de-Broglie wavelength of the electron in the third orbit is nearly
Angular momentum is quantised , hence
Direction (Q. Nos. 12-13) This section contains a paragraph, wach describing theory, experiments, data etc. three Questions related to paragraph have been given.Each question have only one correct answer among the four given ptions (a),(b),(c),(d)
Radius of Bohr’s orbit of H-atom is 52.9 pm. An emission in H-atom starts from the orbit having radius 1.3225 nm and ends at 211.6 pm.
Q.
Wavelength (in nm) associated with this emission is
Radius of Bohr’s orbit of H-atom is 52.9 pm. An emission in H-atom starts from the orbit having radius 1.3225 nm and ends at 211.6 pm.
Q. Spectral line appears in .......... region.
Emission (n2 = 5 to n1 = 2) is called Balmer series and appears in visible region.
Direction (Q. Nos. 14 and 15) Choice the correct combination of elements and column I and coloumn II are given as option (a), (b), (c) and (d), out of which ONE option is correct.
Q.
Match the equation in Column I with the name type in Column II.
lf En = total energy, Kn = kinetic energy, Vn = potential energy and rn = radius of the nth orbit, then based on Bohr’s theory, match the parameter in Column I with the values in Column II.
Direction (Q. Nos. 16 - 19) This section contains 4 questions. when worked out will result in an integer from 0 to 9 (both inclusive)
Q. The energy of an electron in the first Bohr orbit of H-atom is -13.6 eV. What is the possible value of quantum number for the excited state to have energy -3.4 eV?
For Bohr radius;
E = -13.6×Z2/ n2
For H atom, Z=1 and given energy = -3.4
So, we have, -3.4 = -13.6/n2
Or n = 2
An emission is Be3+ in observed at 2.116 A°. In which orbit is it placed?
At what minimum atomic number, a transition from n = 2 to n = 1 energy level results in the emission of X-rays with wavelength 3.0 x 10-8 m?
Find the number of waves made by a Bohr’s electron in one complete revolution in its 3rd orbit
For radius r,
circumference of the orbit = 2πrn
Number of waves in one complete revolution = nλ
For third orbit = nλ = 3λ
Thus, three waves are formed in one revolution.
|
199 videos|330 docs|229 tests
|
|
199 videos|330 docs|229 tests
|
How to Prepare for NEET
Download free EduRev App
(Scan QR code)
|
|
|
© EduRev
|
Education Revolution
|
Follow Us
|
