CAT Exam > CAT Tests > Daily Test for CAT Preparation > CAT Previous Year Questions: Games and Tournaments (July 18) - CAT MCQ
CAT Previous Year Questions: Games and Tournaments (July 18) - CAT MCQ
Test Description
10 Questions MCQ Test Daily Test for CAT Preparation - CAT Previous Year Questions: Games and Tournaments (July 18)
CAT Previous Year Questions: Games and Tournaments (July 18) for CAT 2025 is part of Daily Test for CAT Preparation preparation. The CAT Previous Year Questions: Games and Tournaments (July 18) questions and answers have been
prepared according to the CAT exam syllabus.The CAT Previous Year Questions: Games and Tournaments (July 18) MCQs are made for CAT 2025 Exam. Find important
definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for CAT Previous Year Questions: Games and Tournaments (July 18) below.
Solutions of CAT Previous Year Questions: Games and Tournaments (July 18) questions in English are available as part of our Daily Test for CAT Preparation for CAT & CAT Previous Year Questions: Games and Tournaments (July 18) solutions in
Hindi for Daily Test for CAT Preparation course. Download more important topics, notes, lectures and mock
test series for CAT Exam by signing up for free. Attempt CAT Previous Year Questions: Games and Tournaments (July 18) | 10 questions in 20 minutes | Mock test for CAT preparation | Free important questions MCQ to study Daily Test for CAT Preparation for CAT Exam | Download free PDF with solutions
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 1
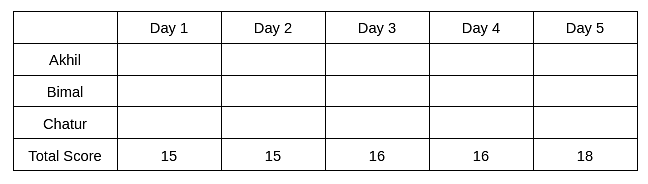
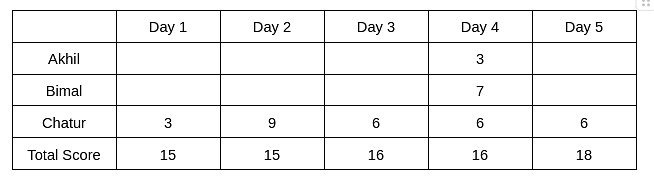
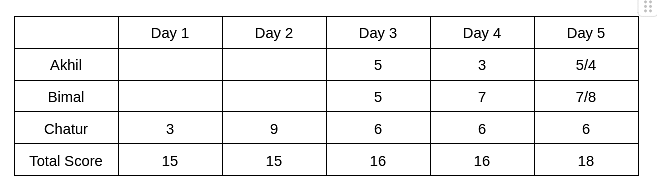
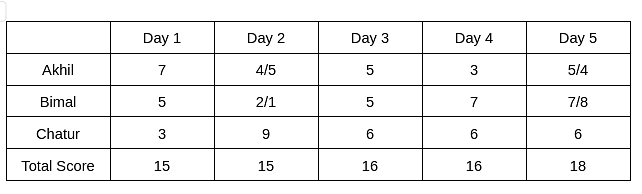
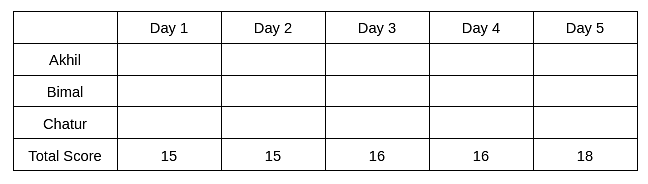
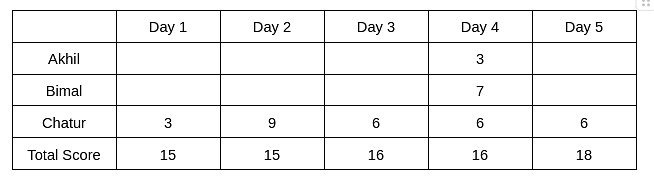
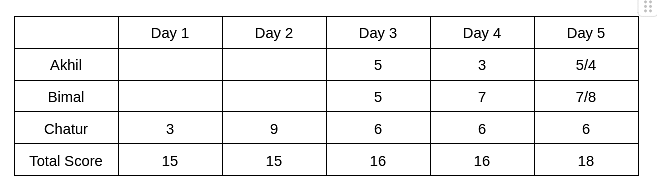
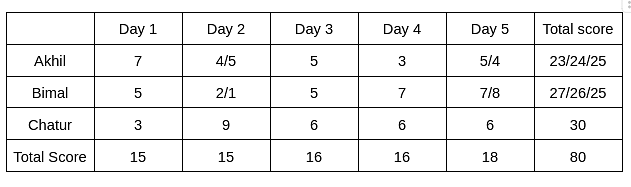
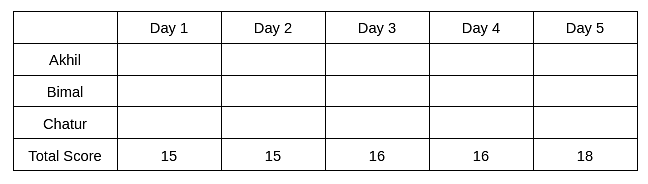
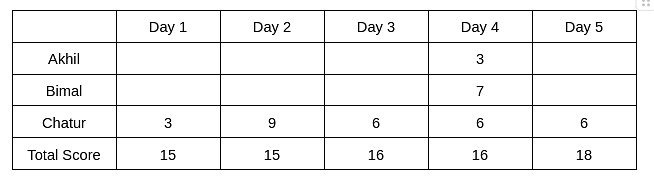
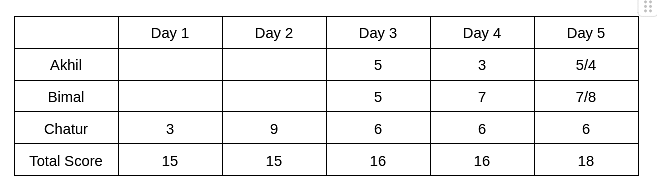
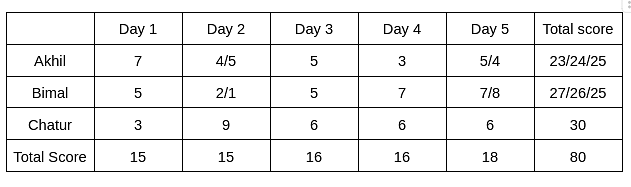
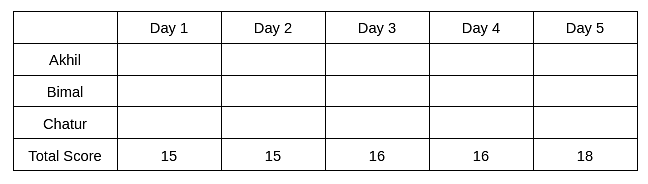
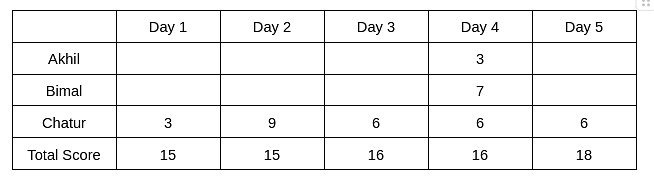
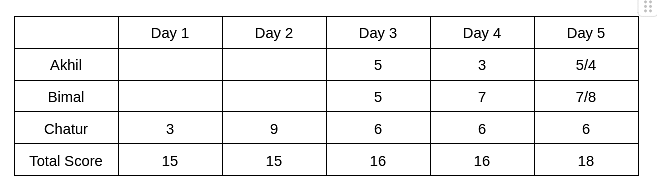
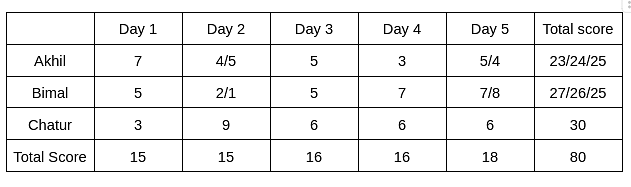
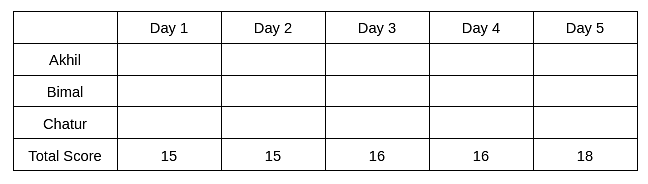
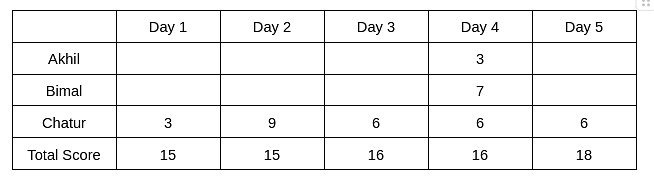
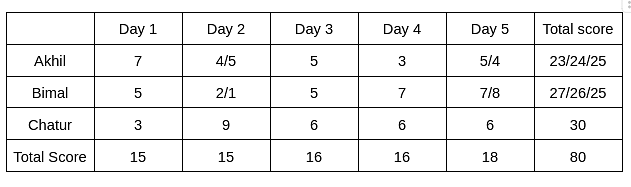
What is Akhil's score on Day 1?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 1
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 2
Who attains the maximum total score?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 2
*Answer can only contain numeric values
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 3
What is the minimum possible total score of Bimal?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 3
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 4
If the total score of Bimal is a multiple of 3, what is the score of Akhil on Day 2?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 4
*Answer can only contain numeric values
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 5
If Akhil attains a total score of 24, then what is the total score of Bimal?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 5
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 6
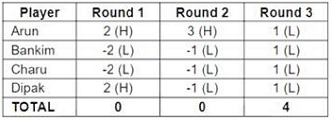
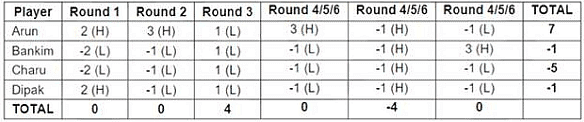
What were the bids by Arun, Bankim, Charu and Dipak, respectively in the first round?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 6
*Answer can only contain numeric values
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 7
In how many rounds did Bankim bid Lo?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 7
*Answer can only contain numeric values
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 8
In how many rounds did Dipak gain exactly 1 point?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 8
*Answer can only contain numeric values
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 9
In how many rounds did Arun bid Hi?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 9
CAT Previous Year Questions: Games and Tournaments (July 18) - Question 10
In which of the following rounds, was Arun DEFINITELY the only player to bid Hi?
Detailed Solution for CAT Previous Year Questions: Games and Tournaments (July 18) - Question 10
|
152 docs|327 tests
|
Information about CAT Previous Year Questions: Games and Tournaments (July 18) Page
In this test you can find the Exam questions for CAT Previous Year Questions: Games and Tournaments (July 18) solved & explained in the simplest way possible.
Besides giving Questions and answers for CAT Previous Year Questions: Games and Tournaments (July 18), EduRev gives you an ample number of Online tests for practice