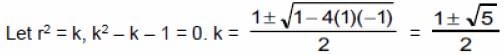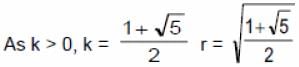CAT Quant Mock Test - 1 - CAT MCQ
25 Questions MCQ Test - CAT Quant Mock Test - 1
The cost of one helmet, two pairs of gloves, three pairs of pads, four bats and five balls increased by 11%, 22%, 33%, 44% and 55% respectively, over that of the previous year. Last year, if I had spent equal amounts purchasing each of the given varieties of items (i.e., helmets, gloves, pads, bats and balls), by what percentage would the total cost of all the items I purchased increase this year, over that in the previous year?
A real number x satisfies the following inequation 2 – 1 / y < x ≤ 5 + 1 / y, for each positive integer y. Which of the following represents the best description of x?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
DIRECTIONS for questions: Select the correct alternative from the given choices.
If (101110101101110)2 = (X)16, then find the number of digits in X each of which is less than 9.
DIRECTIONS for questions: Select the correct alternative from the given choices.
Ajay has a certain number of Rs.1, Rs.2 and Rs.10 notes in his wallet. The number of Rs.1 notes and Rs.2 notes are in the ratio 4 : 3. If the number of Rs.10 notes exceeds the total number of Rs.1 and Rs.2 notes, which of the following is a possible value of the total amount in his wallet?
DIRECTIONS for questions: Select the correct alternative from the given choices.
If the volume of a cylinder decreased by half and its radius decreased by 25%, what is the percentage reduction in its height?
DIRECTIONS for questions: Select the correct alternative from the given choices.
The sides of a rightangled triangle are in geometric progression. Find the common ratio, if it is greater than 1.
DIRECTIONS for questions: Select the correct alternative from the given choices.
A party was attended by twenty five persons. There were a lot of handshakes. Which of the following statements can never be true?
DIRECTIONS for questions: Select the correct alternative from the given choices.
A square sheet of paper has a side of 30 cm. Four squares, each of side x‘ ’ cm, are cut away from each of its corners and the remaining sheet is folded into an open cuboid (i.e., without the top face). What is the maximum possible volume (in cc.) of the cuboid formed?
DIRECTIONS for questions: Select the correct alternative from the given choices.
Fourteen children are playing a game on a beach. Initially they begin the game by making one huge pile of sand and then take turns to divide the pile into smaller piles. If each child, in his or her turn, can divide an existing pile into only m or n smaller piles, for what value of (m, n) is it not possible to get 14 piles of sand at any stage in the game?
DIRECTIONS for questions: Select the correct alternative from the given choices.
If log10 a2b = 5 and log10(a / b2) = 8, then find the value of a.
DIRECTIONS for questions: Select the correct alternative from the given choices.
Two vessels, A and B, contain milk and water respectively. The volume of milk in A and the volume of water in B are in the ratio 4 : 5. Now, x litres of milk from A is poured into B and then y litres of the solution from B is poured into A. If as a result, the concentration of milk in A and that of water in B, both dropped from 100% to 66 x 2/3%, find x : y.
DIRECTIONS for questions: Select the correct alternative from the given choices.
The ithterm, ai, of a sequence is given by the product of the firsti prime numbers starting from 7. The sum of the firstj terms of this sequence is denoted by sj. Which of the following is true?
DIRECTIONS for questions: Select the correct alternative from the given choices.
ABCD is a square, of side 7 units, in the co-ordinate plane, such that the origin lies within the square. If AB is parallel to the y-axis and A is at (−4, 2), what are the coordinates of C?
DIRECTIONS for questions: Select the correct alternative from the given choices. There are two groups of workers, G1 and G2. There are 30 workers in G1 and 60 in G2. Each worker in G1 works three times as fast as any worker in G2. If all the workers in G1 together ploughed a certain field in half a day, then find the time taken by all the workers in G1 and G2 together to plough a field whose area is 50% more than that of the first field.
DIRECTIONS for questions: Select the correct alternative from the given choices.
Consider two cubes of equal volume. In one of the cubes, the biggest possible sphere is inscribed. The other cube is cut into exactly 64 identical small cubes. In each of them, the biggest possible sphere is placed. The volume of the sphere in the first cube is denoted by V1. The total volume of the spheres in the small cubes is denoted by V2. Find V1 : V2.
DIRECTIONS for questions: Select the correct alternative from the given choices.
Mrs. Ahluwalia spent less than Rs.10,000 to buy some cotton sarees and some silk sarees. If Mrs. Ahluwalia had spent the amount of money she actually spent on cotton sarees to buy silk sarees and vice versa, she could have purchased two more sarees. Find the maximum number of sarees that Mrs. Ahluwalia could have originally purchased, if each cotton saree and silk saree costs Rs.300 and Rs.400 respectively.
DIRECTIONS for questions: Select the correct alternative from the given choices.
How many distinct rectangles of area 900 sq.cm are possible with both their length and breadth being integers, when expressed in cm?
DIRECTIONS for questions: Select the correct alternative from the given choices.
If S1 is a set comprising ten integers and S2 is a subset of S1 comprising nine elements, which of the following statements are true?
I.The range of S2 can be more than that of S1
II.The median of S2 can be less than that of S1
III.The mean of S2 can be more than that of S1
DIRECTIONS for questions: Select the correct alternative from the given choices.
Sam and Ram together started a business with initial investments in the ratio of 3 : 4 but Ram left the business aftexr months. If at the end of the year, Sam and Ram shared the profit in the ratio 3 : 2, what is the value of x?
DIRECTIONS for question: Select the correct alternative from the given choices.
If a Ψb = ab − (a + b) + 1
a π b = LCM (a, b) / a
a Λ b = ab / a + b
what is the value of (6 Ψ 13)π (12 Λ 3)?
Fresh dates contain 80% water by weight while dry dates contain 20% water by weight. What is the weight (in kg) of dry dates available from 300 kg of fresh dates?
In an obtuse triangle, the difference between the obtuse angle and one of the acute angles of a triangle is 20°, while the difference between the two acute angles is 56°. Find the measure (in degrees) of the smallest angle of the triangle.
If x is an integer and x3 − 2(x + 2)2 = 993, find the value of x.
In a school, there are three houses, A, B and C. If 170 students leave house B and join house C, the ratio of the number of students in house A and house C would be reversed. Instead, if 40 students leave house A and join house C, then the number of students in house A and house C will be equal. Find the number of students in house A and house C together.
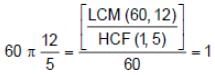
The product of three numbers is 1620. If the HCF of any two out of the three numbers is 3, find the LCM of the three numbers.