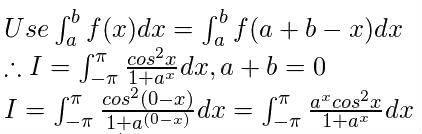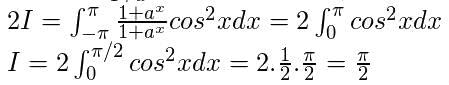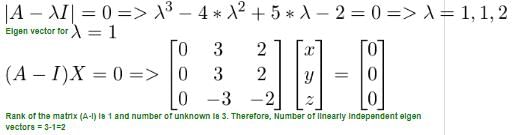Computer Science And IT (CS/IT) Mock Test - 8 For Gate - GATE MCQ
30 Questions MCQ Test - Computer Science And IT (CS/IT) Mock Test - 8 For Gate
Suppose there are two teams A and B. Both of them are competing with each other on a racing track of 1 km. But team A has got the advantage of starting the race from 280 m from the starting point. If the ratio of speed of A to B is 3:4.
Select the correct option from the options given below:
Select the correct option from the options given below:
There are 5 brothers in a family. All were born at a gap of 3 years. If the sum total of ages of 5 brothers is 100. What is the age of 2nd most elder brother ?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If there are 5 persons who can work individually and complete a work in 2, 4, 5, 6 and 8 hours respectively. If they work together then how much time will it take to finish the work ?
If we increase the sides of a rectangular park by 20% , then, what is the total increase in the area of that park?
The sum of the digits in the unit place of all the 4 digit numbers formed with the help of 3, 4, 5 and 6 taken all at a time is:
Choose the one that best fits the sentence below.
The state’s duty is to _______ the safety of its citizen
Cocaine will have pernicious effect on your physical fitness.
Find synonym of the underlined word.
Choose the one that best fits the sentence below.
The _______ of glory lead but to the ______
Gardener said to me, “Don’t pluck the beautiful flowers.”
Select the appropriate Indirect Speech of the above.
I haven’t had a call from the office about the meeting ________ last Monday.
Select the appropriate word to fill in the blank from the given options
Let T(n) = 2T(n/4) + 100√n
The value of T(n) can be written as:
Consider a sorted array of n elements. Let k inversions (swapping) have been performed on the sorted array and we want to sort it again to reverse effect of inversions. If K is very small in comparison to n, then which sorting technique will prove efficient for making again the list in sorted increasing order array.
What will the output of the following C code ?
#include <stdio.h>
int main()
{
int i=2, j=2;
while (i+1 ? --i : j++)
printf("%d",i);
return 0;
}
A balanced tree is given below. How many nodes will become unbalanced when a node is inserted as a child of node G?
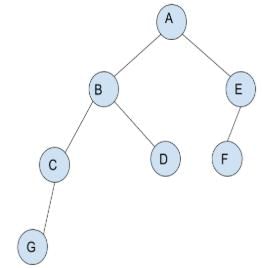
Note: A node in a tree is balanced if absolute difference between its left and right subtrees is less than or equal to 1.
Consider the C code Below.
void function(int n)
{
if (n == 1)
return;
for (int i = 0; i<n; i++)
{
for (int j = 1; j< = n; j++)
{
printf("*");
break;
}
}
}
Which of the following is the tightest upper bound on time complexity of above function.
Consider the following statements about Bellman ford algorithm for finding shortest path in a directed connected graph G having integral edge weights. Statement I: It will always find out negative edge weight cycle in G reachable from source. Statement II: It will always give correct answer for the graph G. Choose from the options given below.
Consider the case: f(n) = O(g(n)). Then, following two statements are claimed to be inferred from the above case.
Statement I: 2f(n) = O(2g(n))
Statement II: 2g(n) = O(2f(n))
Choose the correct option from the given.
Let X and Y be the integers representing the number of simple graphs possible with 3 labeled vertices and 3 unlabeled vertices respectively. Let X - Y = N. Then, find the number of spanning trees possible with N labeled vertices complete graph.
Consider the following pseudo code.
x = 0;
for (i = 1 to n)
for (j = 1 to 4i – 3)
x = x + 1
Find the value of x, when the above code is executed in a function.
Consider the following statements:
S1 : DFS of a directed graph always produces the same number of edges in the traversal, irrespective of the starting vertex.
S2 : If all of the back edges that are found while DFS traversal on directed graph are removed, the resulting graph is acyclic.
Which of the following statements above are valid ?
Which data structure would be the most appropriate to implement a collection of values with the following three characteristics?
i) Items are retrieved and removed from the collection in FIFO order.
ii) There is no priori limit to the number of items in the collection.
iii) The size of an item is large relative to storage required for a memory address.
Stack A has the entries as following sequence a, b, c (with ‘a’ on top), stack B is empty, as shown in the diagram below.
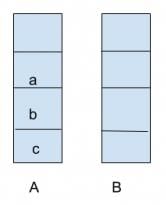 An entry popped
An entry popped
out of stack A can be printed or pushed to stack B. An entry popped out of stack B can only be printed. In this arrangement which of the following permutation of a, b, c are not possible to print?
(i) bac
(ii) bca
(iii) cab
(iv) abc
Considering the data given in the previous question, if the stack A had 4 entries, then the number of possible permutations that can be printed will be:
Let f(n) = Σ [(log(n/2i) +100] where i limits from 0 to k, and n = 2k.
Find the time complexity of f(n).
Given a hash table with n keys and m slots with simple uniform hashing. If collisions are resolved by chaining then what is the probability that the first slot ends up empty ?
Consider the following instance of knapsack problem:
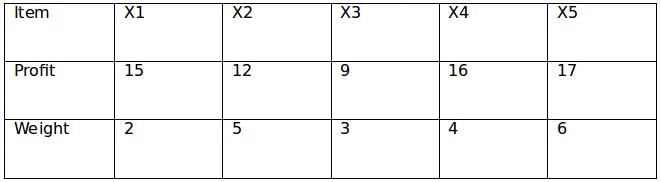
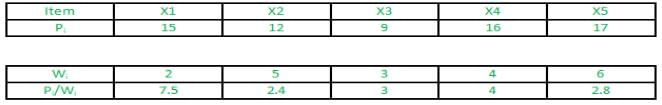
The maximum weight of 12 is allowed in the knapsack. Find the value of maximum profit with the optimal solution of the fractional knapsack problem.
Find the maximum value of the expression (x+y+k) where (x,y) satisfies the equation (x-2)2 + (y-3)2 = 25
Which of the following argument is invalid ?
The value of the following Integral is:
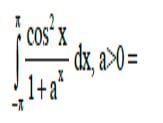
consider a Matrix 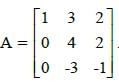 . The number of linearly independhent Eigen Vector for the Eigen value 1 is ______________.
. The number of linearly independhent Eigen Vector for the Eigen value 1 is ______________.