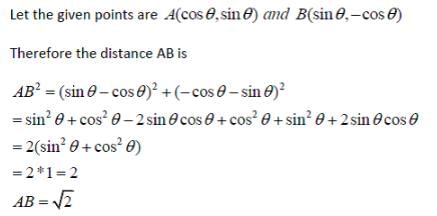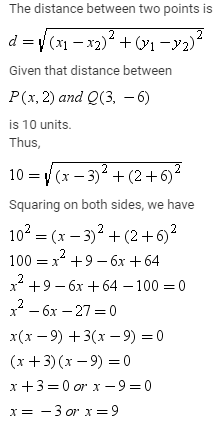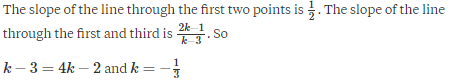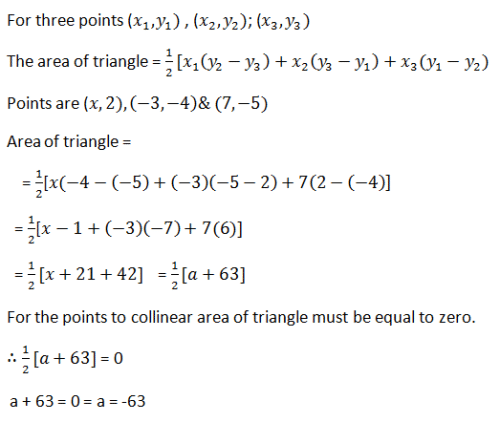Coordinate Geometry - Olympiad Level MCQ, Class 9 Mathematics - Class 9 MCQ

The distance between the points (a cos θ + b sin θ, 0) and (0, a sin θ – b cos θ) is :

If the distance between the points (4, p) and (1, 0) is 5, then p =

A line segment is of length 10 units. If the coordinates of its one end are (2, –3) and the abscissa of the other end is 10, then its ordinate is :

The perimeter of the triangle formed by the points (0, 0), (1, 0) and (0, 1) is :-

If A(2, 2), B(–4, –4) and C(5, –8) are the vertices of a triangle, then the length of the median through vertex C is :

If three points (0, 0), (3, 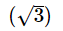 and (3, λ) form an equilateral triangle, then λ =

If the points (k, 2k), (3k, 3k) and (3, 1) are collinear, then k :

The coordinate of the point on X-axis which are equidistant from the points (–3, 4) and (2, 5) are :

If A(5, 3), B(11, –5) and P(12, y) are the vertices of a right triangle right angled at P, then y =

The area of the triangle formed by (a, b + c), (b, c + a) and (c, a + b) is :

If (x – 2), (–3, –4) and (7, –5) are collinear, then x =

If points (t, 2t), (–2, 6) and (3, 1) are collinear, then t =

If the area of the triangle formed by the points (x, 2x), (–2, 6) and (3, 1) is 5 square units, then x =

The line segment joining points (–3, –4), and (1, –2) is divided by y-axis in the ratio :

The ratio in which (4, 5) divides the join of (2, 3) and (7, 8) is :

The ratio in which the x-axis divides the segment joining (3, 6) and (12, –3) is :

If points (1, 2), (–5, 6) and (a, –2) are collinear, then a =

The point A(3, 4) lies in

The point B(-3, 4) lies in

The point C(-5, -2) lies in

The point D(3, -6) lies in

The point P(3, 0) lies