IIFT Quantitative Ability MCQ Quiz - 1 - CAT MCQ
22 Questions MCQ Test - IIFT Quantitative Ability MCQ Quiz - 1
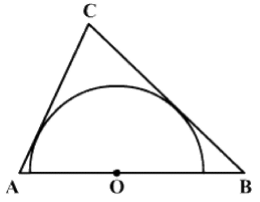
In the figure below, AB = 20 cm, BC = 13 cm and AC = 11 cm. It is given that the area of ΔABC is 66 sq. cm. What is the area (in sq. cm) of the semicircle?
In a 4000 metre race around a circular stadium having circumference 1000 metres, the fastest runner and the slowest runner reach the same point at the end of the 5th minute, for the first time after the start of the race. All the runners start running from the same point and each runner maintains uniform speed throughout the race. If the fastest runner runs at twice the speed of the slowest runner, what is the time taken by the fastest runner to finish the race?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A cow is tethered, using a rope of 20 ft, to a comer of a rectangular house which measures 16 ft by 10 ft, situated in the middle of a very big grass land. What is the maximum area that can be grazed by the cow?
What is the arithmetic mean of {xn, xn + 1, ..., x2n}, where x, n > 1?
Mani invested a sum of Rs. 60,000 with IPO of Accrue Corporation in return for shares of face/issue value of Rs. 10 in the year 2006. In the year 2007, Accrue declared a 2 for 1 stock split together with a bonus share allotment in the ratio 5 : 12. A dividend of 15% was also declared in the year 2007. In the year 2008, the company announced a bonus shares allotment in the ratio 11 : 17 and a dividend of 12%. What is the percentage return for Mani in terms of the dividend obtained? Assume that the dividend is not invested and the value is not affected by inflation.
In a building on the eve of Holi, the residents put up six identical tanks filled with water colours, each with three outlet hoses. Each hose can individually empty the tank to which it is attached in 20 minutes, 30 minutes and 15 minutes respectively. In order to prevent the colours from getting depleted soon, there is also an overhead tap over each tank that pours in fresh colours. The tap can fill the tank underneath it completely in 10 minutes. How long can the residents play Holi with one tank before it gets depleted completely, assuming that all the three outlet hoses and the overhead tap are working simultaneously and continuously?
A bartender pilfers wine from casks. He removes daily 3 litres of Soolah wine from a 30 litres cask of pure soolah wine and replaces it with 2 litres of water and 1 litres of Marcus wine. He continues doing this for a whole week. What is the ratio of soolah wine to water to marcus wine in the cask at the end of the week?
A sheet of paper is cut in the form of an equilateral triangle of side x cm. A long pin is passed through the centroid of the triangle such that it is perpendicular to the paper. If the paper is now spun around the pin, then what is the area of the figure thus formed?
A population of 16000 x 1054 bacteria is required to decompose waste in a landfill. The amount of waste in the landfill is 256 x 1027 kg. How many bacteria would be required to decompose 144 x 109 kg of waste?
Rachel needs a pink hair band. Monica has 2 pink and 3 blue hair bands and Phoebe has 3 pink and 4 blue hair bands. Because they are friends, Monica takes one hair band from Phoebe in exchange for a chocolate muffin. Monica then allows Rachel to pick one hair band from her hair band bag without taking a look at the contents of the bag. What is the probability that Rachel will get what she needs?
Answer the following question based on the information given below.
f(x) = x3 - 2x2 - 5x + 6
g(x) = ln(sin x)
What is the range of g(f(x))?
Centre-point is a town named so because its centre lies on the origin when its country’s map is made using Cartesian co-ordinates. The only railway line in this town runs parallel to the y-axis at a distance of 4 km to the right of the centre of the town. The town planners now plan to build a highway perpendicular to the railway line. The road engineer has specified that the highway should satisfy (2x - y + 1) + k(x + 3y + 2) = 0, but has missed out on specifying k. What is the value of k?
Priya bought chocolates worth Rs. 358 for school kids. She gave each girl an almond flavored chocolate and every boy was given a coffee flavored chocolate. The cost of one coffee and one almond flavored chocolate was Rs. 4.80 and Rs. 3.70 respectively. What can be the number of chocolates of almond and coffee flavors that she bought?
Mr. Mickey went to buy a suit. The marked price of the suit was Rs. 2,000. The shopkeeper was ready to offer a 20% discount on the marked price. Also, Mr. Mickey had a voucher which he could use anytime in the next 12 months to avail a further discount of 25% on this selling price. When he was just about to purchase the suit, he saw that there was an upcoming sale in the next month which would guarantee him a reduction in the marked price by 30%. If the shopkeeper is ready to give him the same discount (i.e. 20%) on this reduced price during the sale, what is the percentage saving of Mr. Mickey if he buys the suit during the sale?
Given the following system of equations:
[log5(2x + 5y)]2 - log5(2x + 5y)6 + 5 = 0 {where (2x + 5+) < 10}
4log2log3(3x +2y) - log3(3x +2y)5 + 6 = 0 {where (3x + 2y) < 10}
What will be the value of 8x + 12y?
What is the difference between the count of odd numbers between and including 300 and 500 and not divisible by 11, and the count of even numbers between and including 100 and 300 and not divisible by 5?
A train takes 40 seconds to cross a cyclist travelling at a speed of 10 kmph in the opposite direction. Another train of same length and 50% faster speed takes 30 seconds to cross a man standing in a station. What is the length of the train?
There are a certain number of stones arranged along a straight line. Mohan picks up the first stone and puts it inside his bag. He breaks the second stone into 2 parts and puts them inside his bag. He breaks the third stone into 3 parts and puts them inside his bag and so on until the last stone. Later he realized that he forgot to break one more stone other than the first stone. Unable to recollect which stone it was, he went to a local mathematician for help. The mathematician counted the number of stones inside the bag and told him that he forgot to break the 17th stone. How many stones were there in the bag?
A ball rebounds to 4/5th of the height it is dropped from. What is the total distance (in m) that the ball travels before coming to rest if it is dropped from 250 m?
A survey was conducted by a firm that develops games to identify the games that kids like. It is perceived that 90 percent of the surveyed kids like GTA and 80 percent of the surveyed kids like FIFA. Further, it is believed that those who like GTA also like Assassin’s Creed but not FIFA, with a conditional probability as high as 0.90. Also, those who like GTA also like FIFA and not Assasin's Creed with a conditional probability of 0.067. There is no kid who likes only GTA. If a randomly surveyed kid likes GTA, what is the probability that he likes all three games?
There are 10 distinct consonants and 5 distinct vowels. How many words can be made using 6 consonants and 4 vowels such that no two vowels are adjacent to each other?

















