Math - 2015 Past Year Paper - IIT JAM MCQ
30 Questions MCQ Test IIT JAM Past Year Papers and Model Test Paper (All Branches) - Math - 2015 Past Year Paper
Suppose N is a normal subgroup of a group G. Which one of the following is true?
Let y(x) = u(x) sin sin x + v(x) cos x be a solution of the differential equation y” + y = sec x.
Then u(x) is
Then u(x) is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Let a, b, c, d be distinct non- zero real numbers with a + b = c + d. Then an eigenvalue of the
matrix 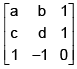 is
is
matrix
Let S be a nonempty subset of R. If S is a finite union of disjoint bounded intervals, then which one of the following is true?
Let {xn} be a convergent sequence of real numbers.
for n ≥ 1, then which one of the following is the limit of this sequence?
The volume of the portion of the solid cylinder x2 + y2 ≤ 2 bounded above by the surface z = x2 + y2 and bounded below by the xy- plane is
Let f: R→R be a differentiable function with f(0) = 0. If for all x ∈ R, 1 < f'(x) < 2, then which one of the following statements is true on (0, ∝)?
If an integral curve of the differential equation passes through (0, 0) and (α, 1),
then α is equal to
Let A be a nonempty subset of R Let I(A) denote the set of interior points of A. Then I(A) can be
Let S3 be the group of permutations of three distinct symbols. The direct sum has an element of order
The orthogonal trajectories of the family of curves y = C1x3 are
Let G be a nonabelian group. Let α ∈ G have order 4 and let β ∈ G have order 3. Then the order of the element αβ in G
Let S be the bounded surface of the cylinder x2 + y2 = 1 cut by the planes z = 0 and z = 1 + x. Then the value of the surface integral is equal to
Suppose that the dependent variables z and w are functions of the independent variables x and y, defined by the equations f(x, y, z, w) = 0 and g(x, y, z, w) = 0, where fzgw – fwgz = 1.
Which one of the following is correct
Let P2(R) be the vector space of polynomials in x of degree at most 2 with real coefficients. Let M2(R) be the vector space of 2 × 2 real matrices. If a linear transformation is defined as
then
Let B1 = {(1, 2), (2, –1)} and B2 = {(1, 0), (0, 1} be ordered bases of R2 . If T : R2 → R2 is a linear transformation such that
then T(5, 5) is equal to
Let f : R → R be a strictly increasing continuous function. If {an} is a sequence in [0, 1], then the sequence {f(an)} is
Which one of the following statements is true for the series
If y(t) is a solution of the differential equation y” + 4y = 2et, then
is equal to
For what real values of x and y, does the integral attain its maximum?
The area of the planar region bounded by the curves x = 6y2 – 2 and x = 2y2 is
For n ≥ 2, let fn: R → R be given by fn(x) = xn sin x. Then at x = 0, fn has a
Let G and H be nonempty subsets of R , where G is connected and G U H is not connected.
Which one of the following statements is true for all such G and H ?
|
29 docs|48 tests
|
|
29 docs|48 tests
|