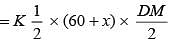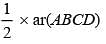Math Olympiad Test: Area of Parallelograms and Triangles- 1 - Class 9 MCQ
10 Questions MCQ Test - Math Olympiad Test: Area of Parallelograms and Triangles- 1
Tick the incorrect statement:
A parallelogram and a rectangle are constructed on same base and between the same parallels, they have
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Point O is the point of intersection of AC and BD of parallelogram ABCD and, also, PQ ∥ AD, then,


P and Q are the points on AB and BC respectively of ∥gm ABCD, then

In ∆ABC, P, Q, R are the midpoints of sides BC, CA, AB respectively, then ar(∆PQR) =

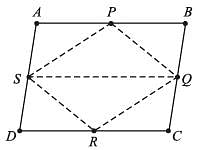
If P, Q, R and S are midpoints of AB, BC, CD and DA of parallelogram ABCD and ar(ABCD) = 26 m2, then ar(PQRS) =
ABC is a right angled ∆ at A, BCED, ACFG and ABMN are squares on sides ABC, AC, AB respectively. AX ⊥ DE meets BC at Y then,

Ar(trap. DCYX) = K, Ar(XYBA), then K =
ABCD is a trapezium in which AB ∥ DC, then

ABCD is a rectangle with O as any point in its interior. If ar (∆AOD) = 3 cm2, and ar(∆BOC) = 6cm2, then ar(ABCD) =


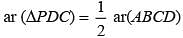
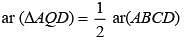

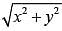 (Pythagoras’ theorem)
(Pythagoras’ theorem)