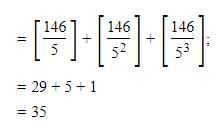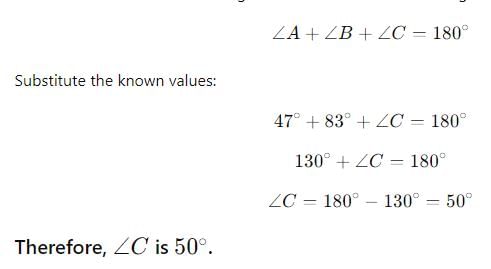Maths Mock Test- 3 - Class 9 MCQ
30 Questions MCQ Test - Maths Mock Test- 3
A rectangular sand box is 5 m wide and 2 m long. How many cubic metres of sand are needed to fill the box upto a depth of 10 cm ?
In Parallelogram ABCD, bisectors of angles A and B intersect each other at O. The measure of ∠AOB is
Three statements are given below:
(I) In a Rectangle ABCD, the diagonals AC bisects ∠A as well as ∠C.
(II) In a Square ABCD, the diagonals AC bisects ∠A as well as ∠C.
(III) In rhombus ABCD, the diagonals AC bisects ∠Aas well as ∠C.
Which is True?
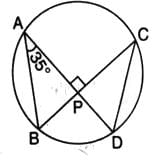
Chords AD and BC intersect each other at right angles at point P. ∠DAB = 35o, then ∠ADC is equal to
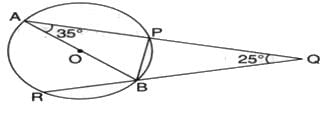
In the given figure, AB is a diameter of the circle APBR. APQ and RBQ are straight lines. If ∠A = 35o and , then the measure of ∠PBR is
The point which lies on y-axis at a distance of 6 units in the positive direction of y-axis is
The length of the longest rod that can fit in a cubical vessel of side 10 cm, is
D and E are the mid-points of the sides AB and AC of △ABC and O is any point on the side BC, O is joined to A. If P and Q are the mid-points of OB and OC res, Then DEQP is
Given Rectangle ABCD and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA res. If length of a diagonal of Rectangle is 8cm, then the quadrilateral PQRS is a
If 146! Is divisible by 5n, and then find the maximum value of n.
In the given figure, if ∠AOB = 80o and ∠ABC = 30o , then ∠CAO is equal to
In the figure, O is the centre of eh circle and ∠AOB = 80o. The value of x is :
The consecutive class marks of a data having continuous class intervals having class width as 5, have a gap of ________ between them.
The point which lies on x-axis at a distance of 3 units in the positive direction of x-axis is
If ABC and PQR are similar triangles in which ∠ A = 470 and ∠ Q = 830, then ∠ C is:
If x = 1,y = 1 is a solution of equation 9ax + 12ay = 63 then, the value of a is :
A polynomial containing two nonzero terms is called a ________.
Opposite angles of a Quadrilateral ABCD are equal. If AB = 4cm, find the length of CD.
In quadrilateral ABCD, ∠B=90∘, ∠C−∠D = 60∘ and ∠A−∠C−∠D = 10∘. Find ∠A, ∠C and ∠D.