CTET & State TET Exam > CTET & State TET Tests > Maths Pedagogy Paper 1 (Nature of Mathematics) - CTET & State TET MCQ
Maths Pedagogy Paper 1 (Nature of Mathematics) - CTET & State TET MCQ
Test Description
10 Questions MCQ Test - Maths Pedagogy Paper 1 (Nature of Mathematics)
Maths Pedagogy Paper 1 (Nature of Mathematics) for CTET & State TET 2025 is part of CTET & State TET preparation. The Maths Pedagogy Paper 1 (Nature of Mathematics) questions and answers have been prepared
according to the CTET & State TET exam syllabus.The Maths Pedagogy Paper 1 (Nature of Mathematics) MCQs are made for CTET & State TET 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Maths Pedagogy Paper 1 (Nature of Mathematics) below.
Solutions of Maths Pedagogy Paper 1 (Nature of Mathematics) questions in English are available as part of our course for CTET & State TET & Maths Pedagogy Paper 1 (Nature of Mathematics) solutions in
Hindi for CTET & State TET course.
Download more important topics, notes, lectures and mock test series for CTET & State TET Exam by signing up for free. Attempt Maths Pedagogy Paper 1 (Nature of Mathematics) | 10 questions in 10 minutes | Mock test for CTET & State TET preparation | Free important questions MCQ to study for CTET & State TET Exam | Download free PDF with solutions
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 1
A teacher asks her students to make a greatest 4 digit number using any four digits with the condition that 5 should be fixed at one's place. This is an example of:
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 1
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 2
Which one of the following is a merit of heuristic method?
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 2
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 3
Major reasons of anxiety and failure in mathematics classroom does not includes
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 3
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 4
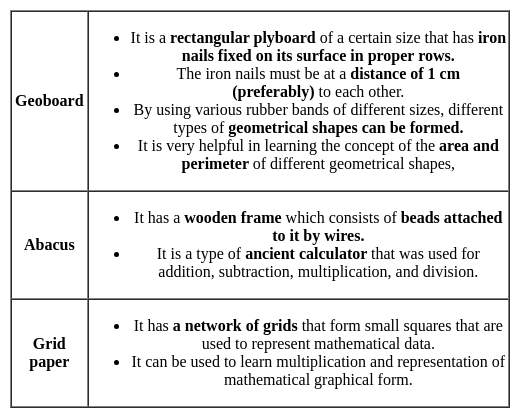
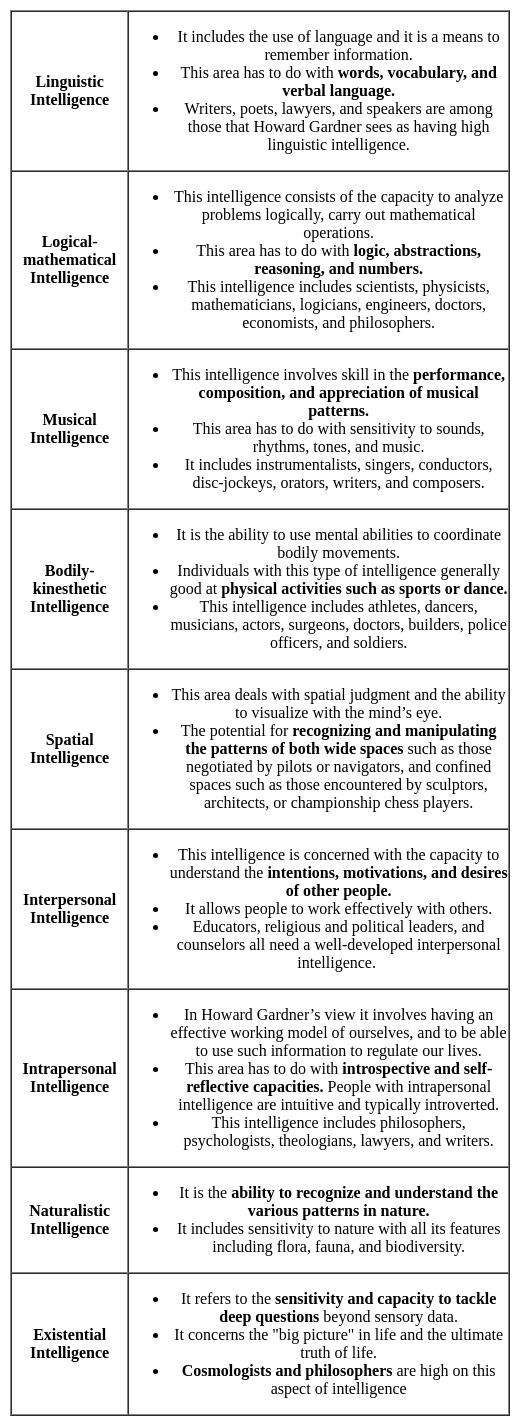
Which of the following manipulative tools are best used to check a child's spatial intelligence ?
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 4
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 5
A mathematics teacher asks this question in class, "The average mass of 15 fishes caught in a pond is 2.5 kg. The mode is 3 kg. What are the possible masses of the 15 fishes? Explain your thinking". This question is
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 5
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 6
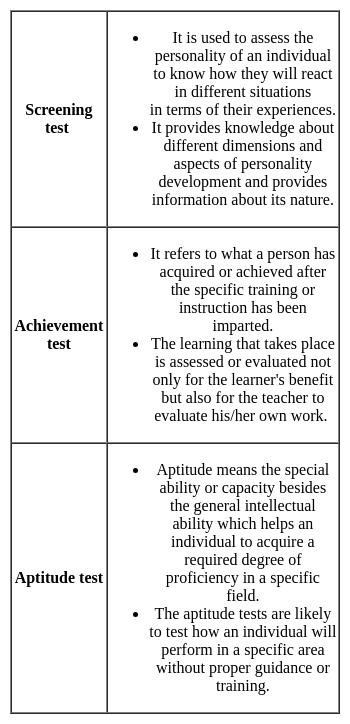
Learning disability in mathematics can be most appropriately assessed by which of the following tests
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 6
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 7
Curriculum of mathematics in primary level in school education should be-
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 7
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 8
Which one of the following is most appropriate to assess children's understanding of Mathematical concept in Class I?
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 8
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 9
Identify the correct one(s)
I. Mathematics puts great emphasis on the teacher's methods of calculating.
II. Mathematics can not be brought out of the child’s everyday situations.
III. Mathematics with reason is rooted in action- learning through doing.
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 9
Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 10
Which one of the following characteristic is not associated with the Social Aspects of teaching Mathematics?
Detailed Solution for Maths Pedagogy Paper 1 (Nature of Mathematics) - Question 10
Information about Maths Pedagogy Paper 1 (Nature of Mathematics) Page
In this test you can find the Exam questions for Maths Pedagogy Paper 1 (Nature of Mathematics) solved & explained in the simplest way possible.
Besides giving Questions and answers for Maths Pedagogy Paper 1 (Nature of Mathematics), EduRev gives you an ample number of Online tests for practice
Download as PDF