Polynomials - Olympiad Level MCQ, Class 9 Mathematics - Class 9 MCQ
30 Questions MCQ Test - Polynomials - Olympiad Level MCQ, Class 9 Mathematics
If 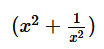 = 102, the value of
= 102, the value of 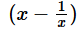 is :-
is :-
If x + 2 is a factor of x3 – 2ax2 + 16, then value of a is
If 3 + 5 – 8 = 0, then the value of (3)3 + (5)3 – (8)3 is
If t2 – 4t + 1 = 0, then the value of is :-
If x + y = 5 and xy = 6, the value of (x3 + y3) is :-
If = 1, then x is equal to :-
If 2x – 2x-1 = 16, then the value of x2 is :-
If x and y are non-zero rational unequal numbers, then is equal to :-
If the value of (x + y + z) is :-
If (x – 2) is a factor of (x2 + 3Qx – 2Q), then the value of Q is :-
If x + 2 is a factor of x3 – 2ax2 + 16, then value of a is
If (x – a) is a factor of (x3 – 3x2a + 2a2x + b), then the value of b is :-
If (x + 2) and (x – 1) are the factors of (x3 + 10x2 + mx + n), the values of m and n are :-
If (x5 – 9x2 + 12x – 14) is divided by (x – 3), the remainder is :-
If (x11 + 1) is divided by (x + 1), the remainder is :-
The value of expression (16x2 + 24x + 9) for x = (-3/4) is :-
When (x3 – 2x2 + px – q) is divided by (x2 – 2x – 3), the remainder is (x – 6). The values of p and q are:-
For making (x4 – 11x2y2 + y4) a perfect square, the expression to be added is :-
The factors of (x4 + 625) are :-
The factors of (x2 – 8x – 20) are :-
The factors of (x2 – 11xy – 60y2) are :-
The factor of (216x3 – 64y3) are :-
The factors of (x3 – 5x2 + 8x – 4) are :-
(x + y)3 – (x – y)3 can be factorized as :-
The factors of x3 – 7x + 6 are :-
If a + b + c = 0, then a2 + b2 + c2 is :-
When x13 + 1 is divided by x + 1, the remainder is :-
When x3 + 2x2 + 2x – 4 and x3 + 2x2 – 3x + 6 are divided by x – 2, the remainder are R1 and R2 respectively.
Which of the following statements is true for R1 and R2 ?



















