RRB JE ME (CBT I) Mock Test- 7 - Mechanical Engineering MCQ
30 Questions MCQ Test - RRB JE ME (CBT I) Mock Test- 7
If x4 - 3x3 + 5x2 - 6x + 2k is divisible by (x - 4), then what is the value of k?
In a pet shop, a number of 9 different types of pets are given below. Find the median number of pets of different types in the shop.
12, 7, 8, 18, 14, 21, 5, 7 and 16
A boatman can row 2 km against the stream in 20 minutes and return in 18 minutes. Find the rate of current?
A boat goes 60 km. downstream in 4 hr. If the speed of the boat is double of the speed of the river, then tell how much distance it travels upstream in 2hr.
In a △ABC, ratio of AB: BC: AC = 5: 8: 7 and perpendicular drawn from A to BC is (5√3) cm long. What is the circum-radius of △ABC?
The simple interest on a certain sum is Rs.240 for 3 years at 8% per annum. The corresponding compound interest is
If (x + 1/x) = 4 and 'x' is less than 2, then what is the value of (x2 - 4x + 6)?
A fraction is equivalent to 4/5. If 8 is added to the numerator and 6 is subtracted from the denominator, the resultant fraction is equivalent to 4/3. Find the difference between the numerator and the denominator of the original fraction.
What is the value of 25% of 40% of [{(√16 − √9) X 5 ÷ 2.5} - 2 + √(80% of 27 ÷ 3 X 5)]?
A series is given: 167, 98, 23, A, 67, 217, B and 134 and mean of all the numbers is 100 and mean of first 4 numbers is 84, then what is the difference between A and B?
A watch which gains 5 seconds in 3 minutes was set right at 7 a.m. In the afternoon of the same day, when the watch indicated quarter past 4 O'clock, the true time is -
A certain number is factorized into 4 parts such that all the parts are in an increasing AP and sum of first and last number is 12 and multiplication of those two numbers is 27, then what is that number?
In what time a cistern be filled by three pipes whose diameters are 1cm, 1(1/3) cm, and 2 cm is running together, when the largest alone will fill it in 61 minutes. It is found that the amount of water flowing in each pipe is proportional to the square of the diameter?
(a+b)% of (a-b) is equal to 2% of the average of a and b. Suppose a2 is 44% more than b2. Then (a+b) is what percent more than (a-b) where a and b both are positive numbers?.
If A:B = 2/3:1/4, B:C = 1/5:1/3, C:D = 1/6:1/7, then (A+B+D):(B+C+D) is equal to
Following is the data regarding the percentage distribution of the number of students in 5 Colleges. A total number of students in all 5 Colleges combined = 12000. First-year and Second year students are known as Junior Year Students and Third year and Fourth year students are known as Senior Year students. The ratio of the number of Junior year and Senior Year students in College A and College B is 5:4 and 3:2.
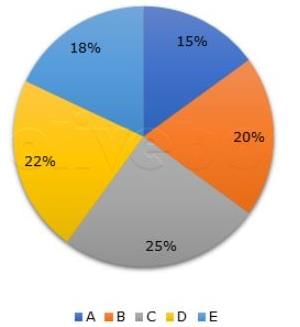
What is the sum of the number of Junior Year students in College A and the number of Senior Year students in College B?
There is a town named Ratanpur. In this town the number of shops are 1/4 of the number of house. The total population of the town is 5 times of the total number of houses. Out of total population 3/5 of the population is adult. The total numbers of females are 7/12 of the total adult population. What is the ratio between the number of houses to the number of females in the given town?
If three numbers are in the ratio 1:3:5 and one-third the sum is 18, then half the sum of their squares is?
The sum of two numbers is 216 and their H.C.F is 27. What are those two numbers?
An accurate clock shows 8 o'clock in the morning. Through how many degrees will the hour hand rotate when the clock shows 2 o'clock in the afternoon?
The Speed of a boat in still water is 15 km/hr and the rate of current is 3 km/hr. The distance travelled downstream in 12 minutes is?
If the nature of roots of two equations: 4x2 - 21x + 35 = 0 and Ax2 + 2x + C = 0 are same, then which of the following can be a value of 'AC'?
In an ODI cricket match Rohit scored 132 runs which included 12 boundaries and 3 sixes. What percents of his runs he made by running between wickets? (1 boundary = 4 runs and 1 six = 6 runs)
Solve and Arrange the following in the proper sequence and mark the answer.
Two articles A and B are marked at Rs.400 and Rs.500 respectively. Discount given on A is 15% while amount of discount on B is double than that given on A. What is the profit per cent if the cost price of both the articles is Rs.300 each?
Given below are the steps involved. Arrange them in the sequential order.
(A) Amount of discount given on B = 2 * 60 = Rs.120
(B) Profit per cent = [(720 - 600)/600] * 100 = 20%
(C) Amount of discount given on A = 15% of 400 = Rs.60
(D) Total cost price = 300 + 300 = Rs.600 and Total selling price = (400 - 60) + (500 - 120) = Rs.720
What is the value of [5 X {25 ÷ (2.5 ÷ 0.5)}] ÷ 2.5 X 2 - 16 ÷ 4 + 16 ÷ 2?
Six men and four boys working together, can complete a piece of work in 8 days. The same piece of work can be completed by four men in 'd' days only and by eight boys in (d +5) days only. By what percentage is the work efficiency of a boy less than that of a man?
Which among the following is irrational number: ∜(7.22 * 202), ∛(52 * 135), (√43.2)/5 and √(82 + 32 + 23)?
A student gets an aggregate of 60% marks in five subjects in the ratio 10:9:8:7:6. If the passing marks are 50% of the maximum marks and each subject has the same maximum marks, in how many subjects did he pass the exam?
Ages of A and B after 2 years will be in the ratio 2: 3 respectively, ages of B and C before two years were in the ratio 7: 8 respectively and present ages of A and C are in the ratio 5: 9 respectively. Find the present age of B.