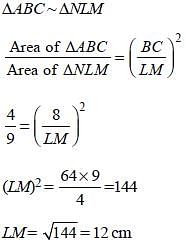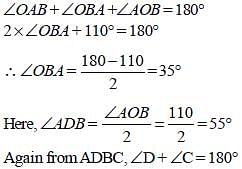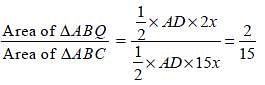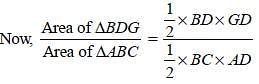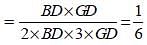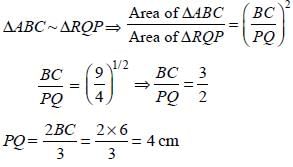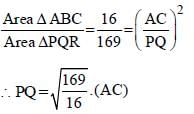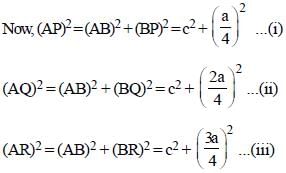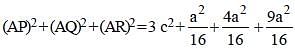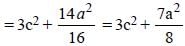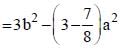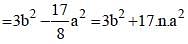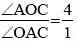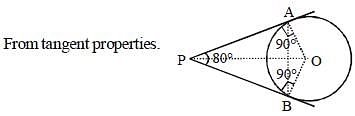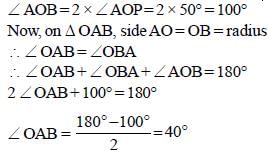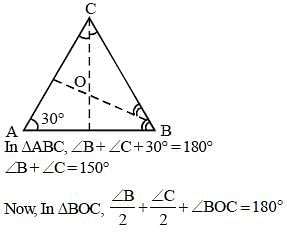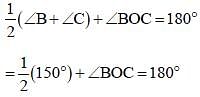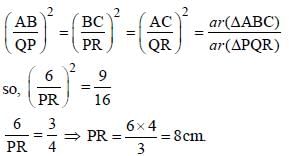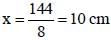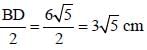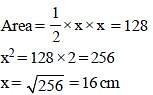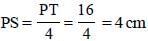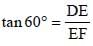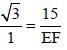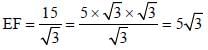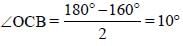SSC CGL Previous Year Questions: Geometry- 1 - SSC CGL MCQ
20 Questions MCQ Test SSC CGL Mathematics Previous Year Paper (Topic-wise) - SSC CGL Previous Year Questions: Geometry- 1
ΔABC ~ ΔNLM and ar(ΔABC) : ar(ΔLMN) = 4 : 9. If AB = 6 cm, BC = 8 cm and AC =12 cm, then ML is equal to: (SSC CGL-2018)
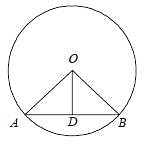
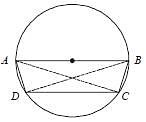
In a circle of radius 17 cm, a chord is at a distance of 8 cm from the centre of the circle. What is the length of the chord? (SSC CGL-2018)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
In a circle with centre O, an arc ABC subtends an angle of 110° at the centre of the circle. The chord AB is produced to a point P. Then ∠CBP is equal to: (SSC CGL-2018)
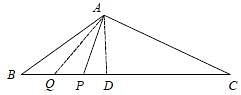
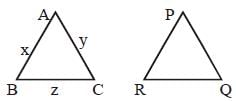
In ΔABC, P is a point on BC such that BP : PC = 4 : 11. If Q is the midpoint of BP, then ar(ΔABQ) : ar(ΔABC) is equal to: (SSC CGL-2018)
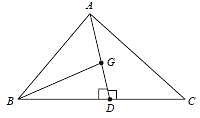
In ΔABC, AD is the median and G is a point on AD such that AG : GD = 2 : 1. Then ar(ΔBDG) : ar(ΔABC) is equal to: (SSC CGL-2018)
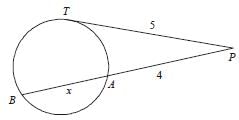
From a point P outside a cir cle, PAB is a secan t and PT is a tangent to the circle, where, A, B and T are points on the circle. If PT = 5 cm, PA = 4 cm and AB = x cm, then x is equal to: (SSC CGL-2018)
ΔABC ~ ΔRQP and AB = 4 cm, BC = 6 cm and AC = 5 cm. If ar(ΔABC) : ar(ΔPQR) = 9 : 4, then PQ is equal to: (SSC CGL-2018)
In a circle with centre O, AB is the diameter and CD is a chord such that ABCD is a trapezium. If ∠BAC = 40°, then ∠CAD is equal to: (SSC CGL-2018)
It is given that ΔABC ~ ΔPRQ and that Area ABC : Area PRQ = 16 : 169. If AB = x, AC = y, BC = z (all in cm), then PQ is equal to: (SSC CHSL-2018)
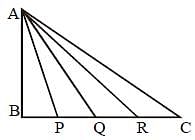
The side BC of a right-angled triangle ABC (∠ABC = 90º) is divided into four equal parts at P, Q and R respectively. If AP2 + AQ2 + AR2 = 3b2 + 17na2, then n is equal to : (SSC CHSL-2018)
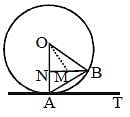
A and B are two points on a circle with centre O. AT is a tangent, such that ∠BAT = 45º. N is a point on OA, such that BN = 10 cm. The length of the median OM of the Δ NOB is : (SSC CHSL-2018)
OABC is a quadrilateral, where O is the centre of a circle and A, B, C are points in the circle, such that ∠ABC = 120º. What is the ratio of the measure of ∠AOC to that of ∠OAC? (SSC CHSL-2018)
PA and PB are two tangents from a point P outside a circle with centre O. If A and B are points on the circle such that ∠APB = 80°, then ∠OAB is equal to: (SSC Sub. Ins. 2018)
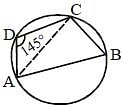
ABCD is a cyclic quadrilateral such that AB is the diameter of the circle circumscribing it and ∠ADC = 145°. What is the measure of ∠BAC? (SSC Sub. Ins. 2018)
In ΔABC, ∠A = 30°. If the bisectors of the angle B and angle C meet at a point O in the interior of the triangle, then ∠BOC is equal to: (SSC Sub. Ins. 2018)
Let ΔABC ~ ΔQPR and 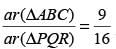 . If AB = 12cm, BC = 6cm and AC = 9cm. Then PR is equal to: (SSC Sub. Ins. 2018)
. If AB = 12cm, BC = 6cm and AC = 9cm. Then PR is equal to: (SSC Sub. Ins. 2018)
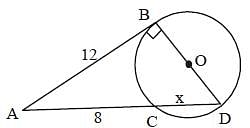
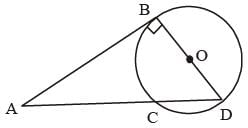
In the given figure , BD passes through centr e O, AB = 12 and AC = 8. What is the radius of the circle? (SSC Sub. Ins. 2017)
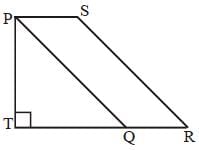
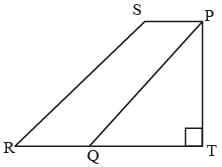
In the given figure, area of isosceles triangle PQT is 128 cm2 and QT = PQ and PQ = 4 PS, PT || SR, then what is the area (in cm2) of the quadrilateral PTRS? (SSC Sub. Ins. 2017)
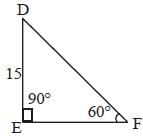
If ΔDEF is right angled at E, DE = 15 and ∠DFE = 60°, then what is the value of EF? (SSC Sub. Ins. 2017)
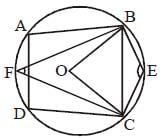
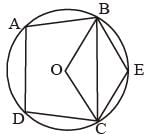
In the given figure, O is the centre of the circle, ∠DAB = 110°and ∠BEC = 100°. What is the value (in degrees) of ∠OCB? (SSC Sub. Ins. 2017)
|
21 docs|55 tests
|
|
21 docs|55 tests
|