All Courses
Get the App Signup / Login
Sign in Open App
CAT Exam  >  CAT Tests  >  Topic-wise Past Year Questions for CAT  >  Test: Analytical Reasoning - 2 - CAT MCQ

Test: Analytical Reasoning - 2 - CAT MCQ


Test Description

20 Questions MCQ Test Topic-wise Past Year Questions for CAT - Test: Analytical Reasoning - 2

Test: Analytical Reasoning - 2 for CAT 2024 is part of Topic-wise Past Year Questions for CAT preparation. The Test: Analytical Reasoning - 2 questions and answers have been prepared according to the CAT exam syllabus.The Test: Analytical Reasoning - 2 MCQs are made for CAT 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Analytical Reasoning - 2 below.
Solutions of Test: Analytical Reasoning - 2 questions in English are available as part of our Topic-wise Past Year Questions for CAT for CAT & Test: Analytical Reasoning - 2 solutions in Hindi for Topic-wise Past Year Questions for CAT course. Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free. Attempt Test: Analytical Reasoning - 2 | 20 questions in 35 minutes | Mock test for CAT preparation | Free important questions MCQ to study Topic-wise Past Year Questions for CAT for CAT Exam | Download free PDF with solutions
Test: Analytical Reasoning - 2 - Question 1
Save

Directions for Questions: Answer the questions on the basis of the information given below.
From ISBT, buses ply on 6 different routes viz. 414, 413, 427, 966, 893 and 181 at an interval of 10 min, 10 min, 12 min, 15 min, 20 min and 30 min, not necessarily in that order, to four different destinations viz. Mehrauli, Badarpur, Uttam Nagar and Azadpur. There is at least one bus for each destination. Further information is also known:
i. Two buses to the same destination cannot start at the same time.
ii. If the timings of two buses plying different routes but heading towards the same destination clash, then the bus of the route number having the shorter time interval will skip this journey.
iii. Buses on two different routes ply between ISBT and Mehrauli.
iv. The difference between the time intervals of a route to Mehrauli and Uttam Nagar is equal to the difference between the time intervals of the two routes to Uttam Nagar.
v. Buses on a route to Mehrauli leaves after every 10 min.
vi. 414 leaves for Badarpur after every 30 min.
vii. Time intervals between two different routes heading towards the same destination cannot be equal.
viii. Buses on one of the routes to Uttam Nagar leave after every 15 min.
ix. Buses to any destination can leave from ISBT with an interval of at least one minute or an integral multiple of one minute.

(2015)

Q. If 427 leaves to Mehrauli after every 10 min, then in a given hour a minimum of how many buses can ply on route 427?

Detailed Solution for Test: Analytical Reasoning - 2 - Question 1

A bus to Uttam Nagar departs after every 15 min.
One of the buses to Mehrauli leaves after every 10 min.
Other bus to Mehrauli can leave after every 12 min or 20 min. 
Let us assume bus on route no. 427 leaves atter every 10 min between 9:00 am & 10:00 am c.e. at 9:00. 9:10. 9:20, 9:30, 9:40, 9:50 and 10:00 a.m. 12 min If timings of buses plying after 12 & 10 min clash then the bus plying after every 12 min will go.
If timings of any of the buses plying after 12 min coincides with departure time of 427 then the next bus timings will clash only after 60 min (LCM of 12 & 10) So maximum of 1 bus timings can clash with route no. 427 in a given hour.
Hence, a minimum 7 – 1= 6 buses on route 427 can depart in an hour.
20 min lf timings of buses plying after 20 & 10 min clash then the bus plying after every 20 min will go.
if timings of any of the bus plying after 20 min coincides with departure time of 427 then the next bus timings will clash again after 20 min (LCM of 20 & 10) If the timings of two buses clash at 9:00 AM then timings will again clash at 9:20. 9:40 and 10:00 Hence, a minimum 7– 4 = 3 buses on route 427 can depart in an hour.

Test: Analytical Reasoning - 2 - Question 2
Save

Directions for Questions: Answer the questions on the basis of the information given below.
From ISBT, buses ply on 6 different routes viz. 414, 413, 427, 966, 893 and 181 at an interval of 10 min, 10 min, 12 min, 15 min, 20 min and 30 min, not necessarily in that order, to four different destinations viz. Mehrauli, Badarpur, Uttam Nagar and Azadpur. There is at least one bus for each destination. Further information is also known:
i. Two buses to the same destination cannot start at the same time.
ii. If the timings of two buses plying different routes but heading towards the same destination clash, then the bus of the route number having the shorter time interval will skip this journey.
iii. Buses on two different routes ply between ISBT and Mehrauli.
iv. The difference between the time intervals of a route to Mehrauli and Uttam Nagar is equal to the difference between the time intervals of the two routes to Uttam Nagar.
v. Buses on a route to Mehrauli leaves after every 10 min.
vi. 414 leaves for Badarpur after every 30 min.
vii. Time intervals between two different routes heading towards the same destination cannot be equal.
viii. Buses on one of the routes to Uttam Nagar leave after every 15 min.
ix. Buses to any destination can leave from ISBT with an interval of at least one minute or an integral multiple of one minute.

(2015)

Q. On a festival day, if frequency of all buses was increased by decreasing the time interval of all the routes by 5 min, then what can be the minimum time difference between any two buses plying to Mehrauli?

Detailed Solution for Test: Analytical Reasoning - 2 - Question 2

If frequency of all buses increases by 5 min then new time intervals become 5, 5, 7, 10, 15 and 25 min.
So now one of the bus to Mehrauli departs after every 5 min. The Other bus can depart after every 7 min or 15 min.
The minimum time difference between buses plying after 5 and 7 min can be 1 min (GCD of 5 and 7).

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Analytical Reasoning - 2 - Question 3
Save

Directions for Questions: Answer the questions on the basis of the information given below.
From ISBT, buses ply on 6 different routes viz. 414, 413, 427, 966, 893 and 181 at an interval of 10 min, 10 min, 12 min, 15 min, 20 min and 30 min, not necessarily in that order, to four different destinations viz. Mehrauli, Badarpur, Uttam Nagar and Azadpur. There is at least one bus for each destination. Further information is also known:
i. Two buses to the same destination cannot start at the same time.
ii. If the timings of two buses plying different routes but heading towards the same destination clash, then the bus of the route number having the shorter time interval will skip this journey.
iii. Buses on two different routes ply between ISBT and Mehrauli.
iv. The difference between the time intervals of a route to Mehrauli and Uttam Nagar is equal to the difference between the time intervals of the two routes to Uttam Nagar.
v. Buses on a route to Mehrauli leaves after every 10 min.
vi. 414 leaves for Badarpur after every 30 min.
vii. Time intervals between two different routes heading towards the same destination cannot be equal.
viii. Buses on one of the routes to Uttam Nagar leave after every 15 min.
ix. Buses to any destination can leave from ISBT with an interval of at least one minute or an integral multiple of one minute.

(2015)

Q. Which of the following statements is necessarily TRUE?

Detailed Solution for Test: Analytical Reasoning - 2 - Question 3

The difference in time intervals between a particular bus to Mehrauli and Uttam Nagar is same as the difference in time intervals between two buses plying towards Uttam Nagar.
Hence, time intervals between Buses for Mehrauli and Uttam Nagar can only be:
Mehrauli – 10 and 12 / 20
Uttam Nagar– 15 and 10 /20
So the time interval between two different routes to Uttam Nagar is always a multiple of 5.

Test: Analytical Reasoning - 2 - Question 4
Save

Directions for Questions: Answer the questions on the basis of the information given below.
From ISBT, buses ply on 6 different routes viz. 414, 413, 427, 966, 893 and 181 at an interval of 10 min, 10 min, 12 min, 15 min, 20 min and 30 min, not necessarily in that order, to four different destinations viz. Mehrauli, Badarpur, Uttam Nagar and Azadpur. There is at least one bus for each destination. Further information is also known:
i. Two buses to the same destination cannot start at the same time.
ii. If the timings of two buses plying different routes but heading towards the same destination clash, then the bus of the route number having the shorter time interval will skip this journey.
iii. Buses on two different routes ply between ISBT and Mehrauli.
iv. The difference between the time intervals of a route to Mehrauli and Uttam Nagar is equal to the difference between the time intervals of the two routes to Uttam Nagar.
v. Buses on a route to Mehrauli leaves after every 10 min.
vi. 414 leaves for Badarpur after every 30 min.
vii. Time intervals between two different routes heading towards the same destination cannot be equal.
viii. Buses on one of the routes to Uttam Nagar leave after every 15 min.
ix. Buses to any destination can leave from ISBT with an interval of at least one minute or an integral multiple of one minute.

(2015)

Q. If condition (iii) is not there, then what can be the minimum difference between the time intervals between the buses plying to Uttam Nagar?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 4

If condition (iii) is waved off then th er e can be possibility of 3 buses plying to Uttam Nagar.
Then 3 buses to Uttam Nagar can ply between intervals 10, 12 and 15 mins or between intervals of 10, 15, 20 mins.
So, the minimum time interval can be in the first case i.e. when 3 buses ply after an interval of 10, 12, 15 mins.
Minimum difference between time interval =12–10 = 2 minute.

Test: Analytical Reasoning - 2 - Question 5
Save

Directions for Questions: Answer the questions on the basis of the information given below.
In a given season of F1 racing, 9 races are to be held. There are 8 teams with two drivers in each team and the points are awarded to the drivers in each race as per to the following table.

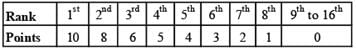
Two championships viz. ‘Driver’s Championship’ and ‘Constructor’s Championship’ take place simultaneously. ‘Driver’s Championship’ is given to the player who has the maximum number of points at the end of the season. ‘Constructor’s Championship’ is given to the team for which the sum of the points of two its drivers is the maximum. A driver is said to get the podium finish only when he is among the top 3 rankers in a race. After the first 6 races, the point standings of the 16 drivers is as follows:

(2015)

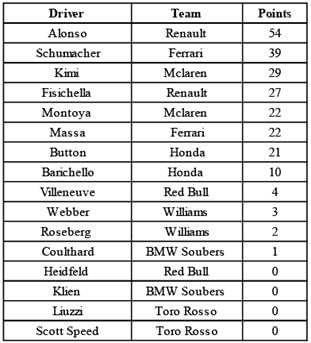
Q. If Alonso got the podium finish in each of the first 6 races, then what was the maximum number of races in which he had 2nd rank?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 5

Alonso finished on podium in each of the first six races and scores 54 points.
He can score 54 points as 10, 10, 10, 8, 8, 8 [in any order] 10, 10, 10, 10, 8, 6
So. he can get 2nd rank in at most 3 races.

Test: Analytical Reasoning - 2 - Question 6
Save

Directions for Questions: Answer the questions on the basis of the information given below.
In a given season of F1 racing, 9 races are to be held. There are 8 teams with two drivers in each team and the points are awarded to the drivers in each race as per to the following table.

Two championships viz. ‘Driver’s Championship’ and ‘Constructor’s Championship’ take place simultaneously. ‘Driver’s Championship’ is given to the player who has the maximum number of points at the end of the season. ‘Constructor’s Championship’ is given to the team for which the sum of the points of two its drivers is the maximum. A driver is said to get the podium finish only when he is among the top 3 rankers in a race. After the first 6 races, the point standings of the 16 drivers is as follows:

(2015)

Q. Apart from the first six races, Alonso got the podium finish in the 7th race as well. However, he was not allowed to participate in the subsequent races due to mechanical failure. At the end of the season, if Schumacher won the ‘Driver’s Championship’, then which of the following could have been his lowest rank in any of the last three races?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 6

Alonso finishes the next race on podium.
⇒ his total points are 60 or 62 or 64.
For finding lowest rank obtained by Schumacher, we take Alonso's score as 60 (lowest among 60, 62, 64) To win the championship Schumacher needs 61 points.
⇒ in the last three races he has to score 61 – 39 = 22 points.
For lowest rank 22 can be scored as 10, 10, 2 (in any order).
Hence, the lowest rank obtained by Schumacher is 7th (corresponding to 2 points).

Test: Analytical Reasoning - 2 - Question 7
Save

Directions for Questions: Answer the questions on the basis of the information given below.
In a given season of F1 racing, 9 races are to be held. There are 8 teams with two drivers in each team and the points are awarded to the drivers in each race as per to the following table.

Two championships viz. ‘Driver’s Championship’ and ‘Constructor’s Championship’ take place simultaneously. ‘Driver’s Championship’ is given to the player who has the maximum number of points at the end of the season. ‘Constructor’s Championship’ is given to the team for which the sum of the points of two its drivers is the maximum. A driver is said to get the podium finish only when he is among the top 3 rankers in a race. After the first 6 races, the point standings of the 16 drivers is as follows:

(2015)


Q. Which of the following statements CANNOT be true?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 7

If Fisichella finishes on podium in race 9 (or in any of the last 3 races), the points scored by Renault will be 87 (or more).
Hence, even if Honda drivers take top two ranks in all three races they will end up with 85 points. i.e. 31 + 3 (8 +10) = 31+ 54 = 85 points.
Hence, in this case Honda won't be able to win the ' 'Constructor's Championship'.

Test: Analytical Reasoning - 2 - Question 8
Save

Directions for Questions: Answer the questions on the basis of the information given below.
In a given season of F1 racing, 9 races are to be held. There are 8 teams with two drivers in each team and the points are awarded to the drivers in each race as per to the following table.

Two championships viz. ‘Driver’s Championship’ and ‘Constructor’s Championship’ take place simultaneously. ‘Driver’s Championship’ is given to the player who has the maximum number of points at the end of the season. ‘Constructor’s Championship’ is given to the team for which the sum of the points of two its drivers is the maximum. A driver is said to get the podium finish only when he is among the top 3 rankers in a race. After the first 6 races, the point standings of the 16 drivers is as follows:

(2015)

Q. If Schumacher ranked 9th in one of the first six races, then which of the following CANNOT be the points scored by him in any one of the first six races?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 8

After first six races Schumacher's total points are 39.
He didn't score any point in 1 race. Hence effectively, he scored 39 points in 5 races and 0 points in 1 race.
If in any of the 5 races he scores 7th rank or 2 points. then in other four races he has to score 37 points, which is not possible in any combination.

Test: Analytical Reasoning - 2 - Question 9
Save

Directions for Questions: Answer the questions on the basis of the information given below.
A group has to be selected from seven persons containing two women (Rehana and Kavya) and five men (Rohit, Rahul, Kamal, Nusarat and John). Rohit would not like to be in the group if Rahul is selected. Rahul and John want to be selected together in the group. Kavya would like to be in the group only if Kamal is also there. Kamal, if selected, would not like Nusarat in the group.
Rohit would like to be in the group only if Nusarat is also there. Kamal insists that Rehana must be selected in case he is there in the group.

(2015)

Q. Which of the following is an acceptable combination of a group of three?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 9

Option (a) Violates the condition that Rahul and John want to be selected together.
Option (b) Violates the condition that Kamal cannot be in the group with Nusarat.
Option (c) Violates the condition that Rahul and John are to be selected together.
Option (d) Rohit, Nusarat, Rehana – is acceptable

Test: Analytical Reasoning - 2 - Question 10
Save

Directions for Questions: Answer the questions on the basis of the information given below.
A group has to be selected from seven persons containing two women (Rehana and Kavya) and five men (Rohit, Rahul, Kamal, Nusarat and John). Rohit would not like to be in the group if Rahul is selected. Rahul and John want to be selected together in the group. Kavya would like to be in the group only if Kamal is also there. Kamal, if selected, would not like Nusarat in the group.
Rohit would like to be in the group only if Nusarat is also there. Kamal insists that Rehana must be selected in case he is there in the group.

(2015)

Q. Which of the following is an acceptable combination of a group of four?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 10

Option (a) Violates the condition that John and Rahul are selected together.
Option (b) Violates the condition that Kamal has to be with Rehana.
Option (c) Rahul, John, Rehana, Kamal – is acceptable
Option (d) Violates the condition that Nusarat cannot be with Kamal.

Test: Analytical Reasoning - 2 - Question 11
Save

Directions for Questions: Answer the questions on the basis of the information given below.
A group has to be selected from seven persons containing two women (Rehana and Kavya) and five men (Rohit, Rahul, Kamal, Nusarat and John). Rohit would not like to be in the group if Rahul is selected. Rahul and John want to be selected together in the group. Kavya would like to be in the group only if Kamal is also there. Kamal, if selected, would not like Nusarat in the group.
Rohit would like to be in the group only if Nusarat is also there. Kamal insists that Rehana must be selected in case he is there in the group.

(2015)

Q. Which of the following statements is true?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 11

Option (a) is not correct as if Kavya and Rohit both the selected then Rahul and John cannot be selected and Kamal and Rehana must be selected. If Kamal is selected then Nusarat cannot be selected but as Rohit is selected Nusarat must be selected which is contradictory.
Option (b) is also incorrect.
Both women  ⇒ Rehana and Kavya
Kavya ⇒ Kamal
Now. one more male is required. He cannot be Rahul or John because they should necessarily be together.
Rohit cannot exist in the group without Nusarat and Nusarat cannot exist because Kamal is already selected.
Hence. a group of 4 having both women is also not possible.
Option (c) is not correct as Kamal should not be with Nusarat and Rohit cannot be with Rahul.

Test: Analytical Reasoning - 2 - Question 12
Save

Directions for Questions: Answer the questions on the basis of the information given below.
A group has to be selected from seven persons containing two women (Rehana and Kavya) and five men (Rohit, Rahul, Kamal, Nusarat and John). Rohit would not like to be in the group if Rahul is selected. Rahul and John want to be selected together in the group. Kavya would like to be in the group only if Kamal is also there. Kamal, if selected, would not like Nusarat in the group.
Rohit would like to be in the group only if Nusarat is also there. Kamal insists that Rehana must be selected in case he is there in the group.

(2015)

Q. If a group of five members has to be selected, then in how many ways is it possible such that Kamal is definitely a member of the group?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 12

The only possible group: Kamal, Kavya, Rehana, Rahul and John.

Test: Analytical Reasoning - 2 - Question 13
Save

Directions for Questions: Answer the questions on the basis of the information given below.
Sixteen teams – A through P – participated in the Hockey World Cup,2013. The tournament was conducted in two stages. In the first stage, the teams were divided into two groups – teams A to H in group 1 and teams I to P in group 2. In the first stage, each team in a group played exactly one match against every other team in that group. At the end of the first stage, the top four teams from each group advanced to the second stage while the rest got eliminated. The second stage comprised three rounds – Quarterfinals, Semi-finals and Finals. A round involves one match for each team. The winner of a match in a round advanced to the next round, while the loser got eliminated. The team that remains undefeated in the second stage was declared the winner of the tournament.
At the end of the first stage, top four teams in each group were determined on the basis of total number of matches won by individual teams; in case, two or more teams in a group were ended up with the same number of wins, ties were resolved by a series of complex tiebreaking rules to determine the top four positions. The teams qualifying for the second stage from group 1 were A, B, C and D and those from group 2 were I, J, K and L. No match in the tournament ended in a draw/tie.

(2015)

Q. In the tournament, if E and L won the same number of matches and L was the winner of the tournament, then what was the sum of the number of matches won by E and that by L?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 13

Since. L is the winner of the tournament. it must have won at least five matches. E is not qualified for second stage, it means E definitely won less than six matches.
Only possible case is shown below:
Number of matches won by E = Number of matches won by L = 5
Hence. required number = 5 + 5 =10.

Test: Analytical Reasoning - 2 - Question 14
Save

Directions for Questions: Answer the questions on the basis of the information given below.
Sixteen teams – A through P – participated in the Hockey World Cup,2013. The tournament was conducted in two stages. In the first stage, the teams were divided into two groups – teams A to H in group 1 and teams I to P in group 2. In the first stage, each team in a group played exactly one match against every other team in that group. At the end of the first stage, the top four teams from each group advanced to the second stage while the rest got eliminated. The second stage comprised three rounds – Quarterfinals, Semi-finals and Finals. A round involves one match for each team. The winner of a match in a round advanced to the next round, while the loser got eliminated. The team that remains undefeated in the second stage was declared the winner of the tournament.
At the end of the first stage, top four teams in each group were determined on the basis of total number of matches won by individual teams; in case, two or more teams in a group were ended up with the same number of wins, ties were resolved by a series of complex tiebreaking rules to determine the top four positions. The teams qualifying for the second stage from group 1 were A, B, C and D and those from group 2 were I, J, K and L. No match in the tournament ended in a draw/tie.

(2015)

Q. The number of matches won in the first stage by a team that advanced to the second stage could not be less than.
Test: Analytical Reasoning - 2 - Question 15
Save

Directions for Questions: Answer the questions on the basis of the information given below.
Sixteen teams – A through P – participated in the Hockey World Cup,2013. The tournament was conducted in two stages. In the first stage, the teams were divided into two groups – teams A to H in group 1 and teams I to P in group 2. In the first stage, each team in a group played exactly one match against every other team in that group. At the end of the first stage, the top four teams from each group advanced to the second stage while the rest got eliminated. The second stage comprised three rounds – Quarterfinals, Semi-finals and Finals. A round involves one match for each team. The winner of a match in a round advanced to the next round, while the loser got eliminated. The team that remains undefeated in the second stage was declared the winner of the tournament.
At the end of the first stage, top four teams in each group were determined on the basis of total number of matches won by individual teams; in case, two or more teams in a group were ended up with the same number of wins, ties were resolved by a series of complex tiebreaking rules to determine the top four positions. The teams qualifying for the second stage from group 1 were A, B, C and D and those from group 2 were I, J, K and L. No match in the tournament ended in a draw/tie.

(2015)

Q. How many of the following statements is/are true?
(i) Maximum number of teams which could have one win in the first stage was 6.
(ii) Maximum number of teams which could have three wins in the first stage was 12.
(iii) Number of teams which had exactly 2 wins in the second stage was 2.
Detailed Solution for Test: Analytical Reasoning - 2 - Question 15

Statement (i) is obviously true.
Three teams in group 1 and three teams in group 2 can win one match each in stage 1.
Statement (ii) is incorrect because maximum number of teams which could have three wins in the first stage would be 14.
Possible case: 3 3 3 3 3 3 3 7 i.e. seven teams in each group would have three wins in the first stage.
Statement (iii) is clearly correct.
Hence, statement (i) and (iii) are correct

Test: Analytical Reasoning - 2 - Question 16
Save

Directions for Questions: Answer the questions on the basis of the information given below.
Sixteen teams – A through P – participated in the Hockey World Cup,2013. The tournament was conducted in two stages. In the first stage, the teams were divided into two groups – teams A to H in group 1 and teams I to P in group 2. In the first stage, each team in a group played exactly one match against every other team in that group. At the end of the first stage, the top four teams from each group advanced to the second stage while the rest got eliminated. The second stage comprised three rounds – Quarterfinals, Semi-finals and Finals. A round involves one match for each team. The winner of a match in a round advanced to the next round, while the loser got eliminated. The team that remains undefeated in the second stage was declared the winner of the tournament.
At the end of the first stage, top four teams in each group were determined on the basis of total number of matches won by individual teams; in case, two or more teams in a group were ended up with the same number of wins, ties were resolved by a series of complex tiebreaking rules to determine the top four positions. The teams qualifying for the second stage from group 1 were A, B, C and D and those from group 2 were I, J, K and L. No match in the tournament ended in a draw/tie.

(2015)

Q. The value of the total of number of matches won, in the first stage, by teams A, B, C and D together could not be more than.
Detailed Solution for Test: Analytical Reasoning - 2 - Question 16

Four teams cannot have six wins each hence maximum number of matches won in the first stage by teams A, B, C and D together would be 22
Possible case for number of wins: 2 1 1 2 4 6 6 6.
Required number = 4 + 6 + 6 + 6 = 22.

Test: Analytical Reasoning - 2 - Question 17
Save

Directions for Questions: Answer the questions on the basis of information given below.
Volleyball is a sport played by two teams on a playing court divided by a net.
The object of the game is to send the ball over the net in order to ground it on the opponent’s court, and to prevent the same effort by the opponent.
The team has three hits for returning the ball. The rally continues until the ball is grounded on the playing court, goes “out” or a team fails to return it properly. In Volleyball, the team winning a rally scores a point (Rally Point System).
There are six players on court in a volleyball team.
Matches are played in five sets. The first four sets are played to 25 points, with the final set being played to 15 points. A team must win a set by at least two points.
There is no ceiling, so a set continues until one of the teams gains a two-point advantage.

(2014)

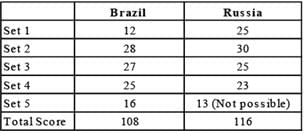
A match was played between Brazil and Russia in which
(i) Only three sets finished with the minimum threshold  points.
(ii) The final score of Russia was same in two of the sets in which it won one of the sets.
(iii) In one of the sets, the final score of Brazil was less than half of Russia.
(iv) The score of Brazil in one of the sets is same as the score of Russia in one of the other set. Both of them lost their respective sets with a different margin.
(v) The total score of five sets of Brazil and Russia were 108 and 116 respectively. Also, Brazil won 3 sets.
(vi) The maximum score by any team in the five sets was 30 and the minimum was 12. Russia scored 23 points in one of the sets.
(vii) There were only three sets in which a team won by exactly two-point advantage.
Q. What was the maximum difference by which a team won the set?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 17

According to the given conditions, the minimum score that a winning team can score in the first four sets is 25 and in the last set is 15.
From statements (iii) and (vi), it can be inferred that in one of the sets the score of Brazil and Russia were 12 and 25 respectively.
From statements (ii), (v) and (vi), there are two possibilities–
Case I: Russia scored the maximum score 30. In this case Russia cannot win any other set as it can win only two sets.
In this scenario, Russia must lose a set with a score of either 25 or 30. With a score of 30 it cannot lose a set as 30 is the maximum score, thus it must have lost a set with a score of 25. In that case, Brazil must have scored 27.
Also, Russia scored 23 points in one of sets, which means Brazil must have scored 25 points (as Russia lostthe set), Now, to make the total of Russia as 116, if must have scored 13 and to make the total of Brazil as 108, it must have scored 16 in the final set, which is not possible. Brazil can reach a score of 1 6 only when Russia scored 14 otherwise Brazil must have won at the score of 15. Thus, it can be said that Russia did not score 30 points.

Case II: Brazil scored 30 points and Russia scored 28. Also, in one of the sets Russia scored 23 points. Now, there are again two possibilities – either Russia won the set as Brazil scored 21 points them it must be the fifth set or Russia lost the set as Brazil scored 25 points and it is one of the first four sets. If the first possibility is considered, in that case. Brazil must win the remaining two sets as Russia has already won two sets (12–25, 21–23) and to win two sets the minimum score of Brazil must be 25 in each set. But in this case. the total score of Brazil in all the five sets will become more than 108.
Hence, Russia lost the setwith the score of 23.
So the scores of three sets are tabulated below–

Now, the sum of the scores of Russia in the remaining two sets is 116 – (25 + 28 + 23) = 40.
Also, from statement (ii) one of the scores of the remaining two sets of Russia must be one of 23 or 25 or 28.
So, the possible scores of Russia in the remaining two sets are (23, 17), (25, 15) and (28, 12). Also, the sum of the scores of Brazil in the remaining two sets must be 108– (12 + 30 + 25) = 41. The various possibilities are–






The maximum difference by which a team won a set is 13 points.

Test: Analytical Reasoning - 2 - Question 18
Save

Directions for Questions: Answer the questions on the basis of information given below.
Volleyball is a sport played by two teams on a playing court divided by a net.
The object of the game is to send the ball over the net in order to ground it on the opponent’s court, and to prevent the same effort by the opponent.
The team has three hits for returning the ball. The rally continues until the ball is grounded on the playing court, goes “out” or a team fails to return it properly. In Volleyball, the team winning a rally scores a point (Rally Point System).
There are six players on court in a volleyball team.
Matches are played in five sets. The first four sets are played to 25 points, with the final set being played to 15 points. A team must win a set by at least two points.
There is no ceiling, so a set continues until one of the teams gains a two-point advantage.

(2014)

A match was played between Brazil and Russia in which
(i) Only three sets finished with the minimum threshold  points.
(ii) The final score of Russia was same in two of the sets in which it won one of the sets.
(iii) In one of the sets, the final score of Brazil was less than half of Russia.
(iv) The score of Brazil in one of the sets is same as the score of Russia in one of the other set. Both of them lost their respective sets with a different margin.
(v) The total score of five sets of Brazil and Russia were 108 and 116 respectively. Also, Brazil won 3 sets.
(vi) The maximum score by any team in the five sets was 30 and the minimum was 12. Russia scored 23 points in one of the sets.
(vii) There were only three sets in which a team won by exactly two-point advantage.
Q. What was the score of Russia in the fifth set?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 18

According to the given conditions, the minimum score that a winning team can score in the first four sets is 25 and in the last set is 15.
From statements (iii) and (vi), it can be inferred that in one of the sets the score of Brazil and Russia were 12 and 25 respectively.
From statements (ii), (v) and (vi), there are two possibilities–
Case I: Russia scored the maximum score 30. In this case Russia cannot win any other set as it can win only two sets.
In this scenario, Russia must lose a set with a score of either 25 or 30. With a score of 30 it cannot lose a set as 30 is the maximum score, thus it must have lost a set with a score of 25. In that case, Brazil must have scored 27.
Also, Russia scored 23 points in one of sets, which means Brazil must have scored 25 points (as Russia lostthe set), Now, to make the total of Russia as 116, if must have scored 13 and to make the total of Brazil as 108, it must have scored 16 in the final set, which is not possible. Brazil can reach a score of 1 6 only when Russia scored 14 otherwise Brazil must have won at the score of 15. Thus, it can be said that Russia did not score 30 points.

Case II: Brazil scored 30 points and Russia scored 28. Also, in one of the sets Russia scored 23 points. Now, there are again two possibilities – either Russia won the set as Brazil scored 21 points them it must be the fifth set or Russia lost the set as Brazil scored 25 points and it is one of the first four sets. If the first possibility is considered, in that case. Brazil must win the remaining two sets as Russia has already won two sets (12–25, 21–23) and to win two sets the minimum score of Brazil must be 25 in each set. But in this case. the total score of Brazil in all the five sets will become more than 108.
Hence, Russia lost the setwith the score of 23.
So the scores of three sets are tabulated below–

Now, the sum of the scores of Russia in the remaining two sets is 116 – (25 + 28 + 23) = 40.
Also, from statement (ii) one of the scores of the remaining two sets of Russia must be one of 23 or 25 or 28.
So, the possible scores of Russia in the remaining two sets are (23, 17), (25, 15) and (28, 12). Also, the sum of the scores of Brazil in the remaining two sets must be 108– (12 + 30 + 25) = 41. The various possibilities are–






* NP – Not Possible
The score of Russia in the fifth set is 12 points.

Test: Analytical Reasoning - 2 - Question 19
Save

Directions for Questions: Answer the questions on the basis of information given below.
Volleyball is a sport played by two teams on a playing court divided by a net.
The object of the game is to send the ball over the net in order to ground it on the opponent’s court, and to prevent the same effort by the opponent.
The team has three hits for returning the ball. The rally continues until the ball is grounded on the playing court, goes “out” or a team fails to return it properly. In Volleyball, the team winning a rally scores a point (Rally Point System).
There are six players on court in a volleyball team.
Matches are played in five sets. The first four sets are played to 25 points, with the final set being played to 15 points. A team must win a set by at least two points.
There is no ceiling, so a set continues until one of the teams gains a two-point advantage.

(2014)

A match was played between Brazil and Russia in which
(i) Only three sets finished with the minimum threshold  points.
(ii) The final score of Russia was same in two of the sets in which it won one of the sets.
(iii) In one of the sets, the final score of Brazil was less than half of Russia.
(iv) The score of Brazil in one of the sets is same as the score of Russia in one of the other set. Both of them lost their respective sets with a different margin.
(v) The total score of five sets of Brazil and Russia were 108 and 116 respectively. Also, Brazil won 3 sets.
(vi) The maximum score by any team in the five sets was 30 and the minimum was 12. Russia scored 23 points in one of the sets.
(vii) There were only three sets in which a team won by exactly two-point advantage.
Q. In how many sets, the score of Brazil was an even number?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 19

According to the given conditions, the minimum score that a winning team can score in the first four sets is 25 and in the last set is 15.
From statements (iii) and (vi), it can be inferred that in one of the sets the score of Brazil and Russia were 12 and 25 respectively.
From statements (ii), (v) and (vi), there are two possibilities–
Case I: Russia scored the maximum score 30. In this case Russia cannot win any other set as it can win only two sets.
In this scenario, Russia must lose a set with a score of either 25 or 30. With a score of 30 it cannot lose a set as 30 is the maximum score, thus it must have lost a set with a score of 25. In that case, Brazil must have scored 27.
Also, Russia scored 23 points in one of sets, which means Brazil must have scored 25 points (as Russia lostthe set), Now, to make the total of Russia as 116, if must have scored 13 and to make the total of Brazil as 108, it must have scored 16 in the final set, which is not possible. Brazil can reach a score of 1 6 only when Russia scored 14 otherwise Brazil must have won at the score of 15. Thus, it can be said that Russia did not score 30 points.

Case II: Brazil scored 30 points and Russia scored 28. Also, in one of the sets Russia scored 23 points. Now, there are again two possibilities – either Russia won the set as Brazil scored 21 points them it must be the fifth set or Russia lost the set as Brazil scored 25 points and it is one of the first four sets. If the first possibility is considered, in that case. Brazil must win the remaining two sets as Russia has already won two sets (12–25, 21–23) and to win two sets the minimum score of Brazil must be 25 in each set. But in this case. the total score of Brazil in all the five sets will become more than 108.
Hence, Russia lost the setwith the score of 23.
So the scores of three sets are tabulated below–

Now, the sum of the scores of Russia in the remaining two sets is 116 – (25 + 28 + 23) = 40.
Also, from statement (ii) one of the scores of the remaining two sets of Russia must be one of 23 or 25 or 28.
So, the possible scores of Russia in the remaining two sets are (23, 17), (25, 15) and (28, 12). Also, the sum of the scores of Brazil in the remaining two sets must be 108– (12 + 30 + 25) = 41. The various possibilities are–






* NP – Not Possible
In three of the sets the score of Brazil was an even number.

Test: Analytical Reasoning - 2 - Question 20
Save

Directions for Questions: Answer the questions on the basis of information given below.
Volleyball is a sport played by two teams on a playing court divided by a net.
The object of the game is to send the ball over the net in order to ground it on the opponent’s court, and to prevent the same effort by the opponent.
The team has three hits for returning the ball. The rally continues until the ball is grounded on the playing court, goes “out” or a team fails to return it properly. In Volleyball, the team winning a rally scores a point (Rally Point System).
There are six players on court in a volleyball team.
Matches are played in five sets. The first four sets are played to 25 points, with the final set being played to 15 points. A team must win a set by at least two points.
There is no ceiling, so a set continues until one of the teams gains a two-point advantage.

(2014)

A match was played between Brazil and Russia in which
(i) Only three sets finished with the minimum threshold  points.
(ii) The final score of Russia was same in two of the sets in which it won one of the sets.
(iii) In one of the sets, the final score of Brazil was less than half of Russia.
(iv) The score of Brazil in one of the sets is same as the score of Russia in one of the other set. Both of them lost their respective sets with a different margin.
(v) The total score of five sets of Brazil and Russia were 108 and 116 respectively. Also, Brazil won 3 sets.
(vi) The maximum score by any team in the five sets was 30 and the minimum was 12. Russia scored 23 points in one of the sets.
(vii) There were only three sets in which a team won by exactly two-point advantage.
Q. What was the score which was common with both the teams, and in which both won their respective sets?
Detailed Solution for Test: Analytical Reasoning - 2 - Question 20

According to the given conditions, the minimum score that a winning team can score in the first four sets is 25 and in the last set is 15.
From statements (iii) and (vi), it can be inferred that in one of the sets the score of Brazil and Russia were 12 and 25 respectively.
From statements (ii), (v) and (vi), there are two possibilities–
Case I: Russia scored the maximum score 30. In this case Russia cannot win any other set as it can win only two sets.
In this scenario, Russia must lose a set with a score of either 25 or 30. With a score of 30 it cannot lose a set as 30 is the maximum score, thus it must have lost a set with a score of 25. In that case, Brazil must have scored 27.
Also, Russia scored 23 points in one of sets, which means Brazil must have scored 25 points (as Russia lostthe set), Now, to make the total of Russia as 116, if must have scored 13 and to make the total of Brazil as 108, it must have scored 16 in the final set, which is not possible. Brazil can reach a score of 1 6 only when Russia scored 14 otherwise Brazil must have won at the score of 15. Thus, it can be said that Russia did not score 30 points.

Case II: Brazil scored 30 points and Russia scored 28. Also, in one of the sets Russia scored 23 points. Now, there are again two possibilities – either Russia won the set as Brazil scored 21 points them it must be the fifth set or Russia lost the set as Brazil scored 25 points and it is one of the first four sets. If the first possibility is considered, in that case. Brazil must win the remaining two sets as Russia has already won two sets (12–25, 21–23) and to win two sets the minimum score of Brazil must be 25 in each set. But in this case. the total score of Brazil in all the five sets will become more than 108.
Hence, Russia lost the setwith the score of 23.
So the scores of three sets are tabulated below–

Now, the sum of the scores of Russia in the remaining two sets is 116 – (25 + 28 + 23) = 40.
Also, from statement (ii) one of the scores of the remaining two sets of Russia must be one of 23 or 25 or 28.
So, the possible scores of Russia in the remaining two sets are (23, 17), (25, 15) and (28, 12). Also, the sum of the scores of Brazil in the remaining two sets must be 108– (12 + 30 + 25) = 41. The various possibilities are–






* NP – Not Possible
The required common score was 25.

43 docs|31 tests
Join Course
Information about Test: Analytical Reasoning - 2 Page
In this test you can find the Exam questions for Test: Analytical Reasoning - 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Analytical Reasoning - 2, EduRev gives you an ample number of Online tests for practice

Top Courses for CAT

Download as PDF

Top Courses for CAT

Important Questions for Analytical Reasoning - 2

Find all the important questions for Analytical Reasoning - 2 at EduRev.Get fully prepared for Analytical Reasoning - 2 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Analytical Reasoning - 2 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Analytical Reasoning - 2 resources.

Analytical Reasoning - 2 MCQs with Answers

Prepare for the Analytical Reasoning - 2 within the CAT exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Analytical Reasoning - 2 Topic-wise Past Year Questions for CAT

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Analytical Reasoning - 2 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup