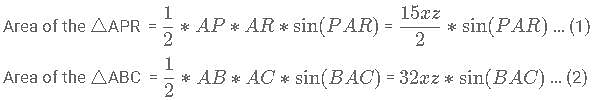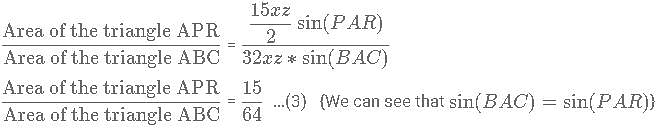Test: CAT Quantitative Aptitude- 2 (October 8) - CAT MCQ
10 Questions MCQ Test Daily Test for CAT Preparation - Test: CAT Quantitative Aptitude- 2 (October 8)
We are told that f(x) is a polynomial function such that f(a)f(b) = f(a) + f(b) + f(ab) - 2 and f(4) = 17, find the value of f(7).
A distinct number of contracts were given to each of the nine major construction companies. Find the least number of contracts that could have been given to the company that got the lowest number of contracts among the nine companies, such that sum of the number of contracts given to any five companies is greater than the sum of number of contracts given to the remaining four companies.
Find the area of the region enclosed between the lines y=2|x|+3 and y=-2|x|+8.
If it is known that xx and yy are two positive numbers such that  what is the minimum value of x + y ?
what is the minimum value of x + y ?
A shopkeeper has 5 dozens of apples. On selling 12 apples for Rs.600, he incurs a loss equal to the cost price of 2 apples. At what total price should he mark the remaining 4 dozens so that even after giving 20% discount, he would have an overall gain of 10%?
Ramesh went to a food joint and bought some burgers and rolls for his family members. In total, he spent less than Rs. 3000. Had Ramesh spent the amount he actually spent on buying burgers to buy rolls and vice versa, he would have ended up buying 3 more items in total. What is the maximum number of burgers that Ramesh could have bought if it is known that a burger and a roll cost Rs. 80 and Rs. 60 respectively?
A wire which was used to form a square of side 30 cm is divided into 3 parts such that the length of the longest part is 25% more than length of the second longest part. If the shortest part is 20 cm shorter than the longest part, then the area (in cm2) of the triangle formed by these three parts is
If log m=300\times×log1.0033333..
What is the approximate value of m?
If N = 823 - 623 - 203, then N can be expressed as a product of ________ with 3
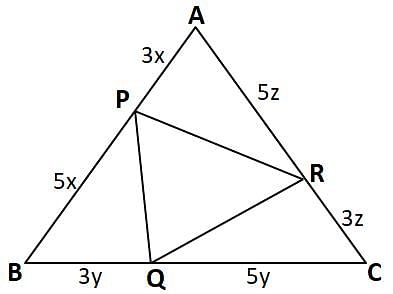
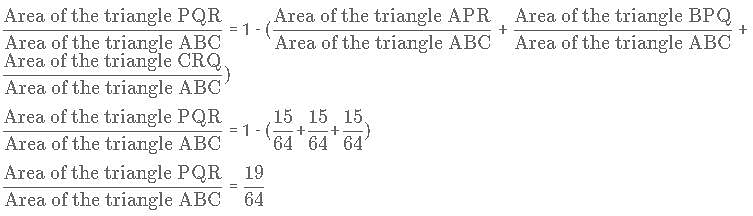
In a △ABC, three points P, Q and R lie on sides AB, BC and CA respectively such that AP : PB = BQ:QC = CR:RA = 3:5. Find out the ratio of the area of \triangle△PQR to that of △ABC.
|
152 docs|327 tests
|



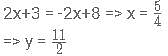
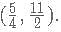
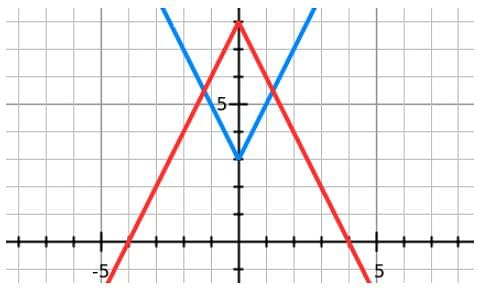

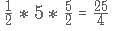 square units.
square units.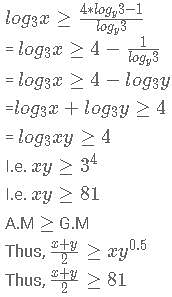

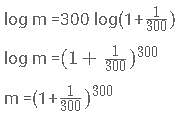
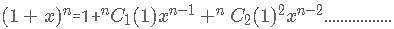
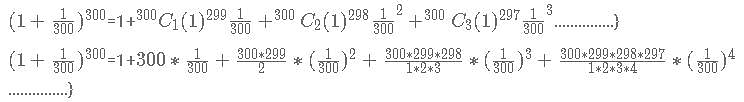
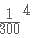 and the succeeding terms are very small, they can be ignored because the fraction at the denominator is increasing and at the numerator is decreasing with the subsequent terms of bionomial.
and the succeeding terms are very small, they can be ignored because the fraction at the denominator is increasing and at the numerator is decreasing with the subsequent terms of bionomial.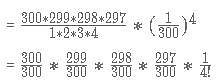
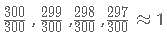 and with every subsequent term
and with every subsequent term 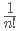 will get smaller. Thus we can neglect those terms of the expansion. )
will get smaller. Thus we can neglect those terms of the expansion. ) 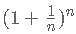 tends to the constant 'e' which is approximately equal to 2.718
tends to the constant 'e' which is approximately equal to 2.718