Test: Factors And Multiples- 2 - GMAT MCQ
15 Questions MCQ Test Quantitative for GMAT - Test: Factors And Multiples- 2
Which of the following CANNOT be the greatest common divisor of two positive integers a and b?
Which of the following CANNOT be the greatest common divisor of two different prime numbers a and b?
Which of the following can be the greatest common divisor of two prime numbers a and b?
Which of the following CANNOT be the least common multiple of two integers a and b, where a and b are both greater than 1?
Which of the following can be the least common multiple of two distinct integers a and b?
What is the value of integer x?
1) The lowest common multiple of x and 16 is 48. ?
2) The greatest common factor of x and 16 is 4.
What is the value of integer x?
1) The lowest common multiple of x and 7 is 28. ?
2) The greatest common factor of x and 7 is 1.
If 375y = x2 and x and y are positive integers, then which of the following must be an integer?
I. y/15
II. y/30
III. y2/25
If x, y, and z are distinct prime numbers, how many positive factors does (xy)z have?
1) z = 5 ?
2) x + y = 10
If x, y, and z are distinct integers, how many positive factors does (xy)z have? (D)
1) z = 5 ?
2) x + y = 10
If a, b, and c are positive integers and (a/6) + (b/5) = (c/30), is c divisible by 5?
1) b is divisible by 5
2) a is even
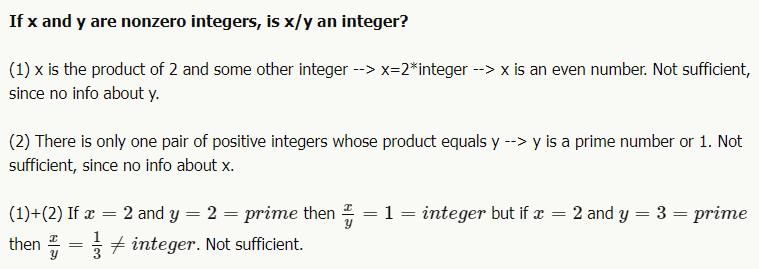
If x and y are nonzero integers, is x/y an integer?
1) x is the product of 2 and another integer. ?
2) There is only one pair of positive integers whose product equals y. ?
If x and y are nonzero integers, is x/y an integer?
(1) x is the product of 2 and some other integer.
(2) There is only one pair of positive integers whose product equals y.
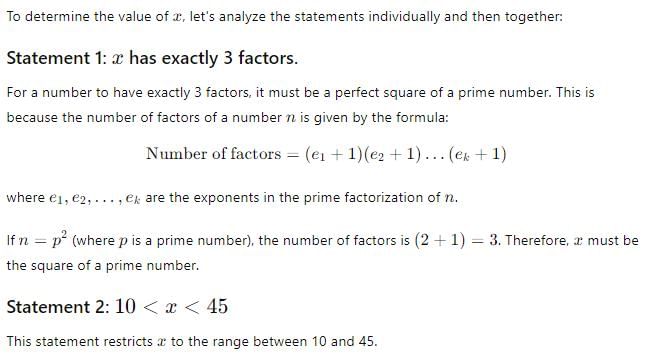
What is the value of x?
1) x has exactly 3 factors.
2) 10 < x < 45
Can a batch of identical cookies be split evenly between Laurel and Jean without leftovers and without breaking a cookie?
1) If the batch of cookies were split among Laurel, Jean and Marc, there would be one cookie left over.
2) If Peter eats three of the cookies before they are split, there will be no leftovers when the cookies are split evenly between Laurel and Jean.
|
127 videos|154 docs|111 tests
|