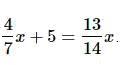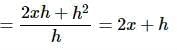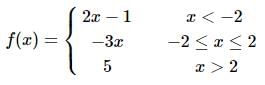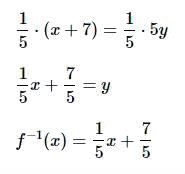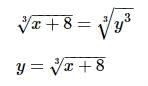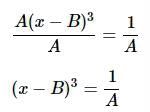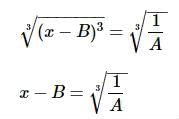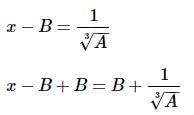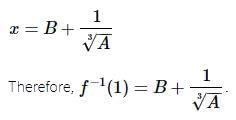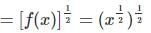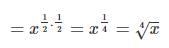Test: Functions- 3 - GMAT MCQ
15 Questions MCQ Test - Test: Functions- 3
There is water tank already 4/7 full. If Jose adds 5 gallons of water to the water tank, the tank will be 13/14 full. How many gallons of water would the water tank hold if it were full?
There exists a set X = {1, 2, 3, 4}. Which of the following defines a function of X?
Let f be a function that assigns x2 to each real number x. Which of the following is NOT an appropriate way to define f?
A sequence begins as follows:
8,12,...
It is formed the same way that the Fibonacci sequence is formed. What are the next two numbers in the sequence?
Define f(x)=x2−4 and g(x)=x2+4.
Give the definition of (f∘g)(x) .
Define the operation Θ as follows:
aΘb=ab+100
Solve for x : 4Θx=72
Define f(x)=A(x−B)3, where A≠0,B≠0.
Evaluate f−1(1) in terms of A and B.
Define an operation ⊙ as follows:
For any real numbers a,b ,
a⊙b=(a−1)(b−1)
Evaluate (5⊙5)⊙5.
An infinite sequence begins as follows:
1,2,−3,4,5,−6,7,8,−9...
Assuming this pattern continues infinitely, what is the sum of the 1000th, 1001st and 1002nd terms?


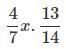 -full would be 13/14x Therefore, we can write out the equation as:
-full would be 13/14x Therefore, we can write out the equation as: