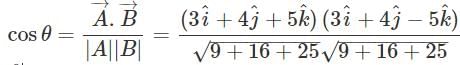NEET Exam > NEET Tests > Test: Introductory Vectors - NEET MCQ
Test: Introductory Vectors - NEET MCQ
Test Description
10 Questions MCQ Test - Test: Introductory Vectors
Test: Introductory Vectors for NEET 2025 is part of NEET preparation. The Test: Introductory Vectors questions and answers have been prepared
according to the NEET exam syllabus.The Test: Introductory Vectors MCQs are made for NEET 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Introductory Vectors below.
Solutions of Test: Introductory Vectors questions in English are available as part of our course for NEET & Test: Introductory Vectors solutions in
Hindi for NEET course.
Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free. Attempt Test: Introductory Vectors | 10 questions in 10 minutes | Mock test for NEET preparation | Free important questions MCQ to study for NEET Exam | Download free PDF with solutions
Test: Introductory Vectors - Question 1
The vector that must be added to the vector  so that the resultant vector is a unit vector along the y-axis is
so that the resultant vector is a unit vector along the y-axis is
 so that the resultant vector is a unit vector along the y-axis is
so that the resultant vector is a unit vector along the y-axis is
Detailed Solution for Test: Introductory Vectors - Question 1
Detailed Solution for Test: Introductory Vectors - Question 2
Detailed Solution for Test: Introductory Vectors - Question 3
Detailed Solution for Test: Introductory Vectors - Question 4
Test: Introductory Vectors - Question 5
Any vector in an arbitrary direction can always be replaced by two (or three)
Detailed Solution for Test: Introductory Vectors - Question 5
Detailed Solution for Test: Introductory Vectors - Question 6
Test: Introductory Vectors - Question 7
The position vector of a particle is 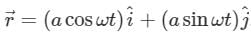 .The velocity of the particle is
.The velocity of the particle is
Detailed Solution for Test: Introductory Vectors - Question 7
Test: Introductory Vectors - Question 8
If a unit vector is represented by ![]() , then the value of c is
, then the value of c is
Detailed Solution for Test: Introductory Vectors - Question 8
Test: Introductory Vectors - Question 9
The position vector of a particle is determined by the expression 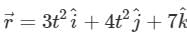 . The distance traversed in first 10 sec is
. The distance traversed in first 10 sec is
Detailed Solution for Test: Introductory Vectors - Question 9
Detailed Solution for Test: Introductory Vectors - Question 10
Information about Test: Introductory Vectors Page
In this test you can find the Exam questions for Test: Introductory Vectors solved & explained in the simplest way possible.
Besides giving Questions and answers for Test: Introductory Vectors, EduRev gives you an ample number of Online tests for practice
Download as PDF


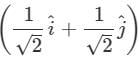 is a
is a 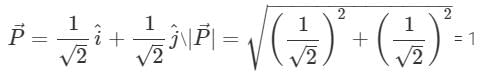

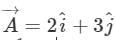 , the angle between
, the angle between 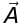 and y-axis is
and y-axis is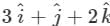 Its length in XY plane is
Its length in XY plane is 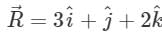
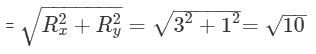
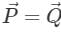 then which of the following is NOT correct
then which of the following is NOT correct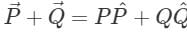
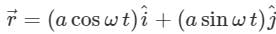
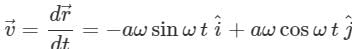
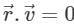 therefore velocity of the particle is perpendicular to the position vector.
therefore velocity of the particle is perpendicular to the position vector.
 will be
will be