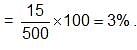Test Level 2: Set Theory - 2 - CAT MCQ
20 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Set Theory - 2
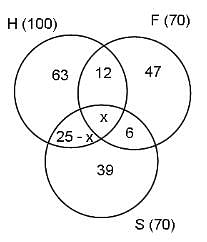
In a school, 100 students were offered Hindi as an optional subject and 70 students were offered French and Sanskrit each. Out of these, 63 were offered Hindi only, 47 were offered French only, 12 were offered Hindi and French only and 6 were offered French and Sanskrit only. The total number of students in the school is
In a group of 1000 people, 750 people can speak Hindi and 400 people can speak English. In the group, all the people speak at least one out of the two languages. How many people can speak only Hindi?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Out of 100 students in Holy Christ School of Science, 40 study Physics and 70 study Mathematics. If there are at least 20 students who study neither Mathematics nor Physics, what is the possible range of students who study both Mathematics and Physics?
In a survey of population of 450 people, it is found that 205 people can speak English, 210 people can speak Hindi and 120 people can speak Tamil. If 100 people can speak both English and Hindi, 80 people can speak both English and Tamil, 35 people can speak Hindi and Tamil, and 20 people can speak all the three languages, then find the number of people who cannot speak English, Hindi, or Tamil.
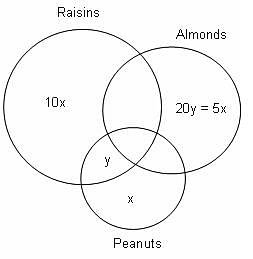
Each of 435 bags contains at least one of the following three items: raisins, almonds, and peanuts; 210 bags contain almonds. The number of bags that contain only raisins is 10 times the number of bags that contain only peanuts. The number of bags that contain only almonds is 20 times the number of bags that contain only raisins and peanuts. The number of bags that contain only peanuts is one-fifth the number of bags that contain only almonds. How many bags contain only one kind of item?
A merchant surveyed 400 people to determine the way they learned about an upcoming sale. The survey showed that 180 learned about the sale from radio, 190 from television, 190 from newspaper, 80 from radio and television, 90 from radio and newspaper, 50 from television and newspaper and 30 from all the three. How many people did not come to know about the sale from any of these sources?
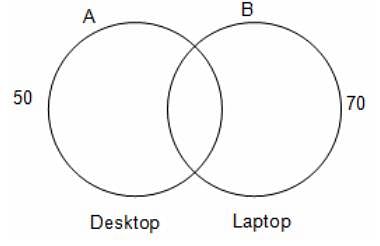
In a survey at Massachusetts Institute of Technology, 80 students were asked whether they used laptop or desktop or both. Of these, 50 students said they used desktop and 70 said they used laptop. What could be the respective minimum and maximum numbers of students who used both desktop and laptop?
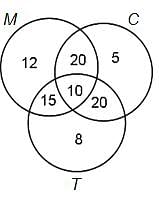
An investigator interviewed 100 students to determine their preferences for the three drinks: milk (M), coffee (C) and tea (T). He reported the following: 10 students liked M, C and T, 20 liked M and C only, 30 liked C and T, 25 liked M and T, 12 liked M only, 5 liked C only and 8 liked T only. How many did not like any of the three drinks?
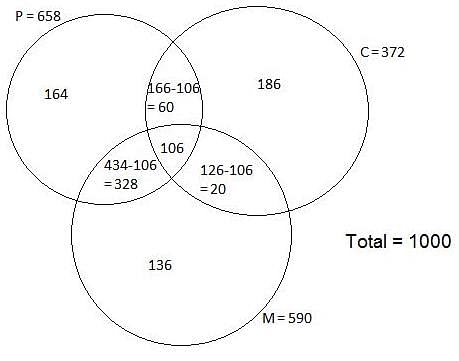
The result of an exam is given below.
Out of 1000 students who appeared-
(i) 658 failed in Physics
(ii) 166 failed in Physics and Chemistry
(iii) 372 failed in Chemistry and 434 failed in Physics and Maths
(iv) 590 failed in Maths and 126 failed in Maths and Chemistry
Find the number of students who failed in Physics but not in Chemistry or Maths.
A survey was conducted to know the number of households who owned either car or bike or both car and bike. It was determined that out of 200 households that were surveyed, 80 used neither bike nor car, 60 used only bike, and for every household that used both car and bike, 3 used only car. How many of the 200 households surveyed used both car and bike?"
Visitors to a modern art museum who watched a certain Picasso painting were asked to fill in a short questionnaire, indicating whether they had enjoyed looking at the picture, and whether they felt they had understood it. According to the results of the survey, all 120 visitors who did not enjoy the painting also did not feel they had understood the painting, and the number of visitors who enjoyed the painting was equal to the number of visitors who felt they had understood the painting. If 3/4th of the visitors who answered the questionnaire both enjoyed the painting and felt they had understood the painting, how many visitors answered the questionnaire?
In a survey of 100 people about their movie-going habits, it was found that 55 people had seen movie X, 45 people had seen movie Y, 50 people had seen movie Z, 10 people had not seen any of the movies and 20 people had seen all the three movies (X, Y and Z). How many people had seen only two of the movies?
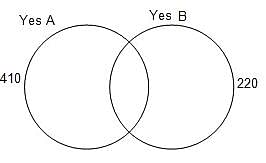
In a survey, 500 people were asked two questions. They were simply to answer 'yes' or 'no'. Of these, 410 people said yes to the first question and 220 people said yes to the second. What is the least number that said yes to both?
Every student of a certain school must take one and only one elective course. Last year, 1/2 of the students took biology as an elective subject, 1/3 of the students took chemistry, and the rest took physics. This year, 1/3 of the students who took biology and 1/4 of the students who took chemistry left school. No other student left and no fresh student was admitted. What fraction of all students took biology and chemistry?
In a survey on political preference, 78% of those asked were in favour of at least one of the proposals I, II and III. 50% of those asked favoured proposal I, 30% of them favoured proposal II, and 20% of them favoured proposal III. If 5% of them favoured all three proposals, then what percentage of those asked favoured more than one of the three proposals?
If X = {8n - 7n - 1 : n ∈ N} and Y = {49(n - 1) : n ∈ N}, then
Directions: Study the given information and answer the following question.
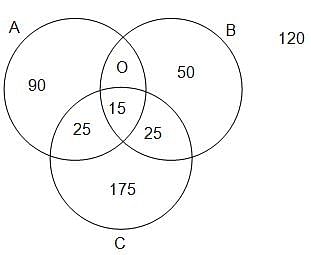
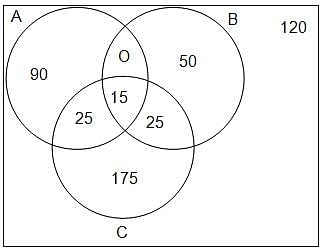
In a survey of 500 customers, the numbers of customers who buy different grades of rice are given below:
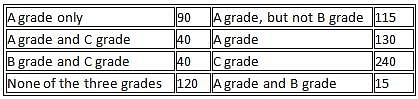
Calculate the approximate percentage of customers who buy all three grades of rice.
Directions: Study the given information and answer the following question.
In a survey of 500 customers, the numbers of customers who buy different grades of rice are given below:
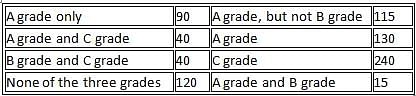
The number of customers who buy A or B, but not C, is
Directions: Read the given information and answer the following question.
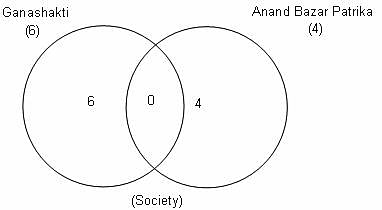
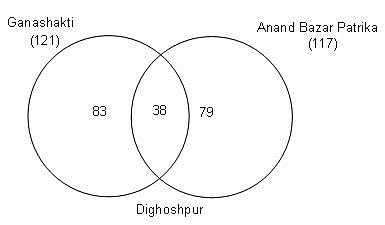
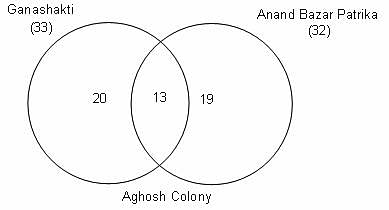
Ghosh Babu is staying at Ghosh Housing Society, Aghosh Colony, Dighoshpur, Kolkata. In Ghosh Housing Society, 6 persons daily read Ganashakti and 4 persons read Anand Bazar Patrika. In this society, there is no person who reads both the newspapers. The total numbers of persons who read these two newspapers in Aghosh Colony and in Dighoshpur are 52 and 200, respectively. The numbers of persons who read Ganashakti in Aghosh Colony and in Dighoshpur are 33 and 121, respectively, while the numbers of persons who read Anand Bazar Patrika in Aghosh Colony and in Dighoshpur are 32 and 117, respectively.
Q. The number of persons in Dighoshpur who read only Ganashakti is
|
5 docs|272 tests
|