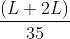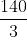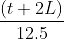Test Level 2: Speed, Time and Distance - 1 - CAT MCQ
10 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Speed, Time and Distance - 1
A monkey starts climbing a 15 feet tree. Each hour, he climbs 3 feet and rests for 30 minutes. During his rest, he slips back 2 feet. How many hours will the monkey take to reach the top?
A man covered a total distance of 1000 km in 16 hours, partly in a taxi at 36 km/hr and partly in a bus at 80 km/hr. The distance covered by the bus is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
x, y and z are the three contestants in a kilometre race. If x can give y a start of 50 metres and x can also give z a start of 69 metres, then how many metres start can y give to z, if the race is supposed to end in a dead heat?
A train passes a 60 metres long platform in 20 seconds and passes a man standing on the platform in 16 seconds. The speed of the train is
In a flat race of L metres, A beats B by x1 metres and C by x3 metres. C will beat B, if
Two trains starting from the same station and travelling in opposite directions are 227.5 km apart in 3 hours 15 min. Had they been travelling in the same direction, they would have been 32.5 km apart in the same time. Find their speeds (in kmph).
Javed wanted to reach the ground floor of the building. He went down at the rate of 1 stair in 1 minute. 16 minutes after he started, Salma started from the same point, from where Javed stared, to reach the ground floor of the building. She went down at the rate of 2 stairs in 1 minute. In how many minutes did Salma overtake Javed?
A man standing inside a tunnel notices that Train A is running inside the tunnel with a speed of 45 kmph. Another Train B, which is half the length of Train A and coming from opposite direction, is running at a speed of 81 kmph. Train A passes Train B in 4 seconds. Which of the following is the closest approximation of the length of the tunnel, if Train A passes completely through it in 4 minutes?
A train of length 500 m crosses a platform in 50 sec and another train of the same length, moving in the opposite direction with double the speed of the first train, crosses a pole in 20 sec. What is the length of the platform?
Varun went for a long ride last Sunday. Every hour, he maintained a constant speed of 60 kmph. But after every 1 hour ride, he took rest for 10 minutes. If he starts from a place A at 8 a.m. and reaches a place B at 2 p.m. on the same day, what is the distance between A and B?
|
5 docs|272 tests
|


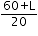 ........ (1)
........ (1)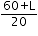
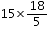 = 54 kmph
= 54 kmph