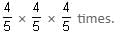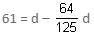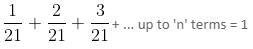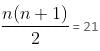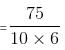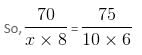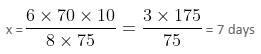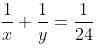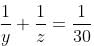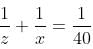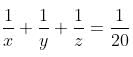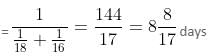Test Level 2: Time & Work - 2 - CAT MCQ
20 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Time & Work - 2
Stuart Little can eat 50 sweets in 4 days and Mickey Mouse can eat 42 sweets in 6 days. If both of them start together, what is the total number of sweets they can eat in 26 days?
54 men can do a piece of work in 90 days, all working at the same rate. If 18 men leave after 62 days, how many days will the remaining men take to complete the work?
A is twice as efficient as B and B is twice as efficient as C. If A, B and C work together, a piece of work will be completed in 12 days. A and B work together for 8 days and thereafter, A is replaced by C.
How many more days are required to complete the work?
Two pipes individually can fill a tank in 10 hours and 12 hours, while a third pipe can empty the full tank in 20 hours. In how much time will the tank be filled, if all three pipes operate together?
A and B can do a piece of work in 20 and 30 days, respectively. A, B and C work together to complete the work. They get Rs. 9000 for completing the work. If they share the money according to the work done by them, then B will get Rs. 1500 more than the amount C gets. How much amount will A get?
P, Q and R can do a piece of work in 11 days, 10 days and 55 days, respectively. On which day will work be completed, if P is assisted by Q and R on alternate days?
There are two identical vessels X and Y. Y is filled with water to the brim and X is empty. There are two pots A and B such that B can hold half as much water as A can hold. One operation is said to be executed when water is transferred from Y to X using A and Y is replenished with water from X using B. If A can hold 1/2 litres of water and it takes 40 operations to equate the water levels in X and Y, then what is the capacity of Y?
20 men could do a piece of work in 30 days working 4 hours a day, 30 men worked for 20 days for 3 hours a day. Then all were laid off, except one. How many more days would it take the last man to complete the remaining work, working 10 hours a day?
A team of 40 employees can complete a project in 60 days, working 6 hours a day. But because of deficiency of resources, only 20 employees can work for the first 20 days at 6 hours per day. Then, the resources are arranged. How many hours per day has the team of 40 employees got to work to complete the project on time?
A can complete a piece of work in 4 days; B takes double the time taken by A; C takes double the time taken by B; and D takes double the time taken by C. They are paired in groups of two each. The first pair takes two-thirds of the time taken by the second pair to complete the work. Who make up the first pair?
Two pipes can fill a cistern in 20 minutes and 10 minutes, respectively. Third pipe can empty it in 25 minutes. Given that 1/5th of the cistern is already full, in how much time will the cistern be full if all three pipes are opened together?
A is 60% more efficient than B. If A takes 9 days less than B to do a certain piece of work, then find the number of days it would take them to complete the same work, if both work together.
'A' can construct a wall in 30 days, whereas 'B' can construct or destroy the same wall in the same time i.e. destroy time is equal to construct time. If 'A' constructs and 'B' destroys, it takes 60 days to construct the wall. How many days will it take to construct the wall, if both 'A' and 'B' work towards constructing the wall?
Five men start a work at 8:00 a.m. so that they can complete the work by 4:00 p.m. If one more man is added at 12:00 noon, then at what time will the work be completed?
A is 20% more efficient than B, but he works for five hours a day, whereas B works for 6 hours a day. If both A and B work together, they complete the work in 6 days. How many days are required to complete the work, if only A works for 3 hours a day?
Some men can do a piece of work in a certain number of days working for a certain number of hours a day. If the number of men, their efficiency and the working hours per day, all are increased by 25%, they complete their work in 61 days less time than estimated. What is the estimated number of days?
Fifteen men could finish a piece of work in 210 days. But at the end of every 10 days, 15 additional men are employed. In how many days will the work be finished?
If 6 men earn 10% of $750 in 10 days, then 8 men will earn 20% of $350 in
A and B can do a piece of work in 24 days. B and C can do a piece of work in 30 days. C and A can do a piece of work in 40 days. How long would they all take to do the same work together?
Mohan can do 1/2 a piece of work in 8 days, while Sohan can do 1/3 of the same work in 6 days. How long would it take for Mohan and Sohan to finish the work?
|
5 docs|250 tests
|



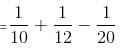

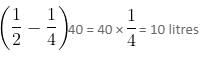
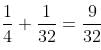 of the work in one day.
of the work in one day.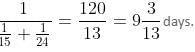
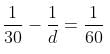
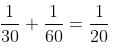
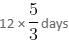 working 3 hours a day, i.e. 20 days.
working 3 hours a day, i.e. 20 days.