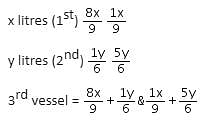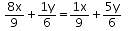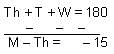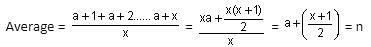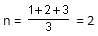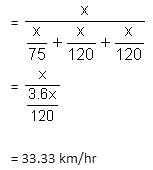Test Level 3: Averages - 1 - CAT MCQ
10 Questions MCQ Test Level-wise Tests for CAT - Test Level 3: Averages - 1
A shipping clerk has five boxes of different but unknown weights, each weighing less than 100 kg. The clerk weighs the boxes in pairs. The weights obtained are 110, 112, 113, 114, 115, 116, 117, 118, 120 and 121 kg. What is the weight of the heaviest box?
Two vessels contain mixtures of milk and water in ratios 8 : 1 and 1 : 5, respectively. The contents of both of these are mixed in a specific ratio into a third vessel. How much mixture should be drawn from the second vessel to fill the third vessel (capacity 26 gallons) completely in order that the resulting mixture may be half milk and half water?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The average temperature on Monday, Tuesday and Wednesday was 55°C and that on Tuesday, Wednesday and Thursday was 60°C. Find the temperature on Monday, if the temperature on Thursday was 56°C.
The average of x consecutive integers is n and the average of the next y consecutive integers is n + 6. Find the relation between x and y.
The average weight of 12 oil drums is 60 kg, which is equal to the difference between the weights of the two heaviest drums. If the average weight of the remaining 10 drums is half the total average weight, then find the weight of the heaviest drum.
One hundred and twenty students take an examination with maximum 100 marks (with no fractional marks). No three students are awarded the same marks. What is the smallest possible number of pairs of students who are awarded the same marks?
A worker earned an average of $1500 per month from January to May. Then, he earned $2000, $2500, $2475 and $2325 during the months of June, July, August and September, respectively. He went on a vacation for the whole month of October. During November, he earned 100% more than what he earned in December. If his average earnings for the entire year was $1800, find his earnings in the month of November (in $).
One-third of a certain journey was covered at a speed of 25 km/hr, one-fourth at a speed of 30 km/hr and the rest at a speed of 50 km/hr. Find the average speed for the whole journey.
A baseball team, which played 60 games, won 30% of its played games. After a phenomenal winning streak, this team raised its winning percentage to 50%. How many more games must the team have won in order to attain this average?
Which of these will definitely not constitute an operation for getting the minimum possible average value for group A?
|
5 docs|272 tests
|