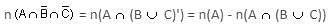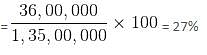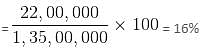Test Level 3: Set Theory - CAT MCQ
10 Questions MCQ Test Level-wise Tests for CAT - Test Level 3: Set Theory
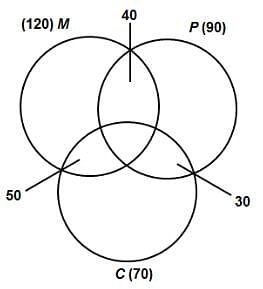
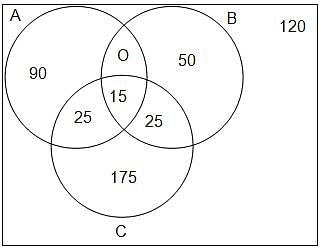
In a survey of 200 students of a higher secondary school, it was found that 120 studied Mathematics, 90 studied Physics, 70 studied Chemistry, 40 studied Mathematics and Physics, 30 studied Physics and Chemistry, 50 studied Chemistry and Mathematics and 20 studied none of these subjects. Find the number of students who studied all the three subjects.
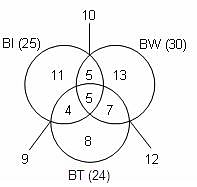
In a survey conducted among 60 managers, it was found that 25 read Business India, 30 read Business World and 24 read Business Today; 10 read both Business India and Business World, 9 read both Business India and Business Today and 12 read both Business World and Business Today. If 5 managers read all three newspapers, then how many managers read only one newspaper?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
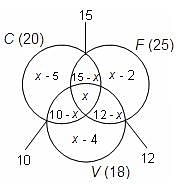
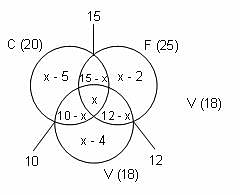
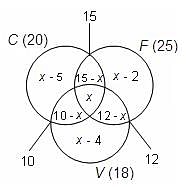
In a class of 33 students, 20 play cricket, 25 play football and 18 play volleyball. 15 play both cricket and football, 12 play both football and volleyball and 10 play both cricket and volleyball. If each student plays at least one game, find the number of students who play only cricket.
In a class of 33 students, 20 play cricket, 25 play football and 18 play volleyball. 15 play both cricket and football, 12 play both football and volleyball and 10 play both cricket and volleyball. If each student plays at least one game, then find the number of students who play any two games.
Directions: Study the given information and answer the following question.
In a survey of 500 customers, the numbers of customers who buy different grades of rice are given below:
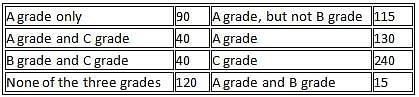
The ratio of frequency of 'B only' to that of 'Both A and C, but not B' is
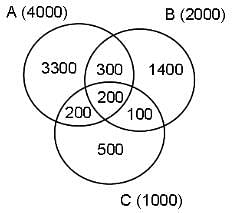
In a town of 10000 families, it was found that 40% families buy newspaper A, 20% families buy newspaper B and 10% families buy newspaper C, 5% families buy A and B, 3% buy B and C and 4% buy A and C. If 2% families buy all the three newspapers, then the number of families that buy A only is
Directions: The following question is based on the Venn diagram given below:
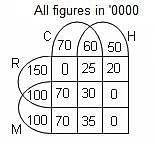
This Venn diagram represents four sets of TV viewers who watch the programmes Mahabharata, Ramayana, Chitrahaar and Hindi films. M, R, C and H respectively represent the number of viewers who watch Mahabharata, Ramayana, Chitrahaar and Hindi films; and M1, R1, C1 and H1 represent respectively the viewers who do not watch them. For example, MR1 and CH1 represent the viewers who watch Mahabharata and Chitrahaar but not Ramayana and Hindi films. (The numbers given are in 10,000).
Q. What is the approximate difference in percentage between people who watch Hindi films and those who watch Chitrahaar? (Assume the TV viewers to be 13.5 million)
In a class of 33 students, 20 play cricket, 25 play football, 18 play volleyball, 15 play both cricket and football, 12 play football and volleyball and 10 play cricket and volleyball. If each student plays at least one game, find the number of students who play all the three games.
In a class of 33 students, 20 play cricket, 25 play football and 18 play volleyball. 15 play both cricket and football, 12 play both football and volleyball and 10 play both cricket and volleyball. If each student plays at least one game, then find the number of students who play only one game.
Three rugs have a combined area of 200 m2. When partially overlapped, the rugs cover a floor area of 140 m2 and the area covered by exactly two layers of rug is 24 m2. What area of the floor is covered by all three layers of rug?
|
5 docs|272 tests
|