All Courses
Get the App Signup / Login
Sign in Open App
Banking Exams  >  Mathematics (Maths) Class 8  >  Test: Mensuration- 2 Download as PDF

Test: Mensuration- 2


Test Description

10 Questions MCQ Test Mathematics (Maths) Class 8 | Test: Mensuration- 2

Test: Mensuration- 2 for Banking Exams 2023 is part of Mathematics (Maths) Class 8 preparation. The Test: Mensuration- 2 questions and answers have been prepared according to the Banking Exams exam syllabus.The Test: Mensuration- 2 MCQs are made for Banking Exams 2023 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Mensuration- 2 below.
Solutions of Test: Mensuration- 2 questions in English are available as part of our Mathematics (Maths) Class 8 for Banking Exams & Test: Mensuration- 2 solutions in Hindi for Mathematics (Maths) Class 8 course. Download more important topics, notes, lectures and mock test series for Banking Exams Exam by signing up for free. Attempt Test: Mensuration- 2 | 10 questions in 10 minutes | Mock test for Banking Exams preparation | Free important questions MCQ to study Mathematics (Maths) Class 8 for Banking Exams Exam | Download free PDF with solutions
1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Mensuration- 2 - Question 1
Save

Find the volume of a cuboid whose length is 8 cm, breadth 6 cm and height 3.5 cm. 

Detailed Solution for Test: Mensuration- 2 - Question 1 (8×6)×3.5=48×3.5= 168.00cm cube.
Test: Mensuration- 2 - Question 2
Save

Find the volume of a cuboid whose length is 8 cm, width is 3 cm and height is 5 cm. 

Detailed Solution for Test: Mensuration- 2 - Question 2 Volume of cubiod =l×b×h
=8×3×5
=120cm3 .
Test: Mensuration- 2 - Question 3
Save

Find the perimeter of the a square with side 4cm.

Detailed Solution for Test: Mensuration- 2 - Question 3 Because the perimeter of square is 4×side
so, 4×4=16
Test: Mensuration- 2 - Question 4
Save

Find the cube root of 140 × 2450.
Detailed Solution for Test: Mensuration- 2 - Question 4

140 x 2450 = 343000
Cube root of 343000 = (343000)1/3 = (703)1/3 = 70

Test: Mensuration- 2 - Question 5
Save

A rectangular paper of width 7 cm is rolled along its width and a cylinder of radius 20 cm is formed. Find the volume of the cylinder. 
Detailed Solution for Test: Mensuration- 2 - Question 5
Given  : A rectangular paper of width 14 cm is rolled along its width to form a cylinder.

Height of cylinder =  h  =  7 cm = width of the rectangular paper

And
Radius of cylinder ( Given ) =  r  = 20 cm

And
we know Volume of cylinder  = π r^2 h , So

Volume of our given cylinder = 22/7 x 20 x 20 x 7  = 8800 cm^3    
Test: Mensuration- 2 - Question 6
Save

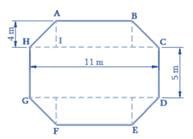
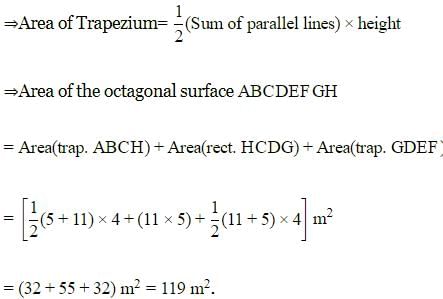
Top surface of a raised platform is in the shape of regular octagon as shown in the figure. Find the area of the octagonal surface.
Detailed Solution for Test: Mensuration- 2 - Question 6

This regular octagon can be into two trapeziums (each having height 4 m and parallel sides as 11 m and 5 m) and a rectangular part with length 11 m and breadth 5 m.​

Test: Mensuration- 2 - Question 7
Save

The diagonal of a quadrilateral shaped field is 24 m and perpendicular dropped on it from the remaining opposite vertices are 6 m and 12 m. Find the area of the field.
Detailed Solution for Test: Mensuration- 2 - Question 7

area=1/2×d×(h1+h2)
=1/2×24×(12+6)
=12(18)
=216 m2

Test: Mensuration- 2 - Question 8
Save

If the parallel sides of a parallelogram are 2 cm apart and their sum is 10 cm then its area is:
Test: Mensuration- 2 - Question 9
Save

If the edge of a cube is 1 cm then which of the following is its total surface area?
Detailed Solution for Test: Mensuration- 2 - Question 9 Total surface area of cube is 6*length^2
=6×1^2
=6×1
=6 cm2
Test: Mensuration- 2 - Question 10
Save

Find the area of a triangle whose base is 4 cm and altitude is 6 cm.
Detailed Solution for Test: Mensuration- 2 - Question 10

We know that area of triangle is equals to 1/2 base × altitude.
Here, base = 4 cm and altitude = 6 cm.
So, area = 1/2 × 4 × 6= 24 /2= 12 cm2.

197 videos|360 docs|48 tests
Join Course
Information about Test: Mensuration- 2 Page
In this test you can find the Exam questions for Test: Mensuration- 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Mensuration- 2, EduRev gives you an ample number of Online tests for practice

Up next

Volume & Capacity
Surface Area and Volume of Cube, Cuboid & Cylinder
Surface Area of Cuboid
Olympiad Test: Mensuration
Volume vs Capacity
197 videos|360 docs|48 tests
Join Course
Download as PDF

Download free EduRev App

Track your progress, build streaks, highlight & save important lessons and more!
(Scan QR code)
© EduRev
Education Revolution
Follow Us