Test: Polynomial Zeros - Grade 10 MCQ
15 Questions MCQ Test - Test: Polynomial Zeros
If the graph of the quadratic polynomial is completely above or below the x-axis, then the nature of roots of the polynomial is _____
A polynomial is said to be linear, quadratic, cubic or biquadratic according to the degree of the polynomial.
A real number is called zeros of the polynomial p(x) if _________
What will be the nature of the zeros of a quadratic polynomial if it cuts the x-axis at two different points?
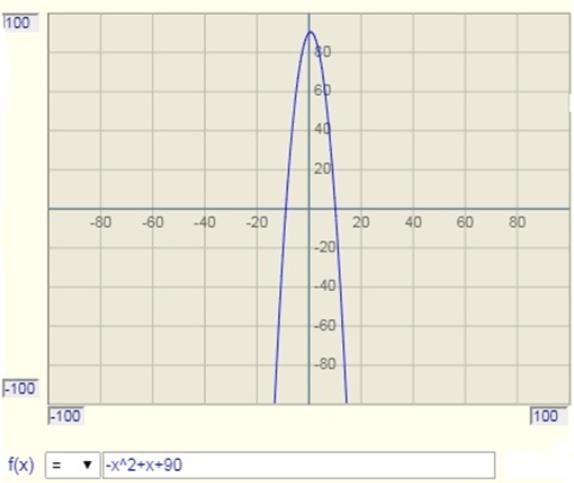
The graph of the quadratic polynomial -x2 + x + 90 will open upwards.
The graph of the polynomial 2x2 - 8x + 5 cuts the y-axis at _________.
If the zeros of a polynomial are 3 and -5, then they cut the x-axis at ____ and _____ points.
If a < 0, then the graph of ax2 + bx + c, has a downward opening.
The graph of a quadratic polynomial cuts the x-axis at only one point. Hence, the zeros of the quadratic polynomial are equal and real.
If the graph of a polynomial cuts the x-axis at 3 points, then the polynomial is ______
How many points will the graph of x2 + 2x + 1 will cut the x-axis?
The graph of the polynomial 4x2 - 8x + 3 cuts the x-axis at ________ and ________ points.