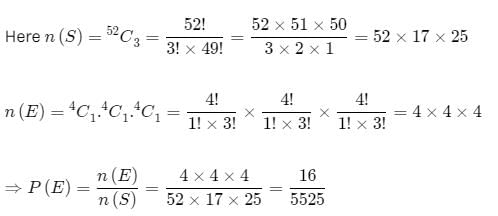All Courses
Get the App Signup / Login
Sign in Open App
Bank Exams Exam  >  Bank Exams Tests  >  IBPS PO Prelims & Mains Preparation  >  Test: Probability- 4 - Bank Exams MCQ

Test: Probability- 4 - Bank Exams MCQ


Test Description

15 Questions MCQ Test IBPS PO Prelims & Mains Preparation - Test: Probability- 4

Test: Probability- 4 for Bank Exams 2024 is part of IBPS PO Prelims & Mains Preparation preparation. The Test: Probability- 4 questions and answers have been prepared according to the Bank Exams exam syllabus.The Test: Probability- 4 MCQs are made for Bank Exams 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Probability- 4 below.
Solutions of Test: Probability- 4 questions in English are available as part of our IBPS PO Prelims & Mains Preparation for Bank Exams & Test: Probability- 4 solutions in Hindi for IBPS PO Prelims & Mains Preparation course. Download more important topics, notes, lectures and mock test series for Bank Exams Exam by signing up for free. Attempt Test: Probability- 4 | 15 questions in 15 minutes | Mock test for Bank Exams preparation | Free important questions MCQ to study IBPS PO Prelims & Mains Preparation for Bank Exams Exam | Download free PDF with solutions
Test: Probability- 4 - Question 1
Save

A bag contains four black and five red balls. If three balls from the bag are chosen at random, what is the chance that they are all black?

Detailed Solution for Test: Probability- 4 - Question 1

Black and Black and Black = 4/9 x 3/8 x 2/7 = 23/504 = 1/21.

Test: Probability- 4 - Question 2
Save

From a pack of 52 playing cards, three cards are drawn at random. Find the probability of drawing a king, a queen and jack.

Detailed Solution for Test: Probability- 4 - Question 2

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Probability- 4 - Question 3
Save

A group of investigators took a fair sample of 1972 children from the general population and found that there are 1000 boys and 972 girls. If the investigators claim that their research is so accurate that the sex of a new bom child can be predicted based on the ratio of the sample of the population, then what is the expectation in terms of the probability that a new child bom will be a girl?

Detailed Solution for Test: Probability- 4 - Question 3

972/1972 = 243/ 493.

Test: Probability- 4 - Question 4
Save

A bag contains 6 red, 4 white and 8 blue balls. If three balls are drawn at random, find the probability that (i) all the three balls are of the same colour.
Detailed Solution for Test: Probability- 4 - Question 4

The required probability would be given by: All are Red OR All are white OR All are Blue = (6/18) x (5/17) x (4/16) + (4/18) x (3/17) x (2/16) + (8/18) x (7/17)x (6/16) = 480/(18 x 17 x 16) 5/51 

Test: Probability- 4 - Question 5
Save

A and B are two candidates seeking admission to the IIMs. The probability that A is selected is 0.5 and the probability that both A and B are selected is at most 0.3. Is it possible that the probability of B getting selected is 0.9.
Detailed Solution for Test: Probability- 4 - Question 5

P (Both are selected) = P(A) x P(B) Since P(A) = 0.5, we get 0.3 = 0.5 x 0.6.
The maximum value of P(B) = 0.6.
Thus P{B) = 0.9 is not possible.

Test: Probability- 4 - Question 6
Save

The odds in favour of standing first of three students Amit, Vikas and Vivek appearing at an examination are 1 : 2. 2 : 5 and 1 : 7 respectively. What is the probability that either of them will stand first (assume that a tie for the first place is not possible).
Detailed Solution for Test: Probability- 4 - Question 6

P (Amit) = 1/3

P (Vikas) = 2/7

P (Vivek) = 1/8.
Required Probability = 1/3 + 2/7 + 1/8 = 125/168.

Test: Probability- 4 - Question 7
Save

A and B are two mutually exclusive events of an experiment. If P(A') = 0.65, P(A u B) = 0.65 and P(B) = p , find the value o f p.
Detailed Solution for Test: Probability- 4 - Question 7

P(A) = 1 - 0.65 = 0.35.
Hence, P(B) = 0.65 - 0.35 = 0.3

Test: Probability- 4 - Question 8
Save

The odds against an event is 5 : 3 and the odds in favour of another independent event is 7 : 5. Find the probability that at least one of the two events will occur.
Detailed Solution for Test: Probability- 4 - Question 8

P (E1) 3/8

P (E2) = 7/12.
Event definition is: E1 occurs and E2 does not occur or E1 occurs and E2 occurs or E2 occurs and E1 does not occur.

(3/8) x (5/12) + (3/8) x (7/12) + (5/8) x (7/12) = 71/96.

Test: Probability- 4 - Question 9
Save

A husband and a wife appear in an interview for two vacancies for the same post. The probability of husband’s selection is (1/7) and that of the wife’s selection is 1/5. What is the probability that (i) both of them will be selected?
Detailed Solution for Test: Probability- 4 - Question 9

(i) 1/5 x 1/7 = 1/35

Test: Probability- 4 - Question 10
Save

There are two bags, one of them contains 5 red and 7 white balls and the other 3 red and 12 white balls, and a ball is to be drawn from one or the other of the two bags. Find the chance of drawing a red ball.
Detailed Solution for Test: Probability- 4 - Question 10

The event can be defined as: First bag is selected and red ball is drawn. 1/2 x 5/12 + y2 x 3/15 = (5/24) + (3/30) = 37/120

Test: Probability- 4 - Question 11
Save

Two fairdices are thrown. Giventh at the sum of the dice is less than or equal to 4, find the probability that only one dice shows two.
Detailed Solution for Test: Probability- 4 - Question 11

The possible outcomes are: (1, 1); (1, 2); (2, 1), (2, 2); (3, 1); (1, 3).
Out of six cases, in two cases there is exactly one ‘2’ Thus, the correct answer is 2/6 =1/3.

Test: Probability- 4 - Question 12
Save

If 8 coins are tossed, what is the chance that one and only one will turn up Head?
Detailed Solution for Test: Probability- 4 - Question 12

One head and seven tails would have eight positions where the head can come.
Thus, 8 x (1/2)8 = (1/32)

Test: Probability- 4 - Question 13
Save

Out of all the 2 - digit integers between 1 to 200, a 2-digit number has to be selected at random. What is the probability that the selected number is not divisible by 7?
Detailed Solution for Test: Probability- 4 - Question 13

The count of the event will be given by: The number of all 2 digit integers - the number of all 2 digit integers divisible by 7

Test: Probability- 4 - Question 14
Save

Tom and Dick are running in the same race; the probability of their winning are 1/5 and 1/2 respectively . Find the probability that (i) either of them will win the race.
Detailed Solution for Test: Probability- 4 - Question 14

(i) 1/5 + 1/2 = 7/10.

Test: Probability- 4 - Question 15
Save

Amamath appears in an exam that has 4 subjects. The chance he passes an individual subject’s test is 0.8. What is the probability that he will (i) pass in all the subjects?
Detailed Solution for Test: Probability- 4 - Question 15

The event definitions are:

(a) Passes the first AND Passes the second AND Passes the third AND Passes the fourth

(b) Fails the first AND Fails the second AND Fails the third AND Fails the fourth

(c) Fails all is the non-event

541 videos|683 docs|263 tests
Join Course
Information about Test: Probability- 4 Page
In this test you can find the Exam questions for Test: Probability- 4 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Probability- 4, EduRev gives you an ample number of Online tests for practice

Top Courses for Bank Exams

541 videos|683 docs|263 tests
Join Course
Download as PDF

Top Courses for Bank Exams

Important Questions for Probability- 4

Find all the important questions for Probability- 4 at EduRev.Get fully prepared for Probability- 4 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Probability- 4 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Probability- 4 resources.

Probability- 4 MCQs with Answers

Prepare for the Probability- 4 within the Bank Exams exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Probability- 4 IBPS PO Prelims & Mains Preparation

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Probability- 4 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup