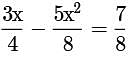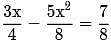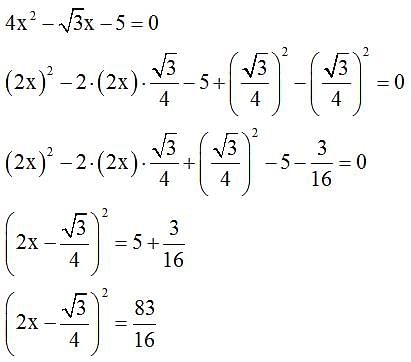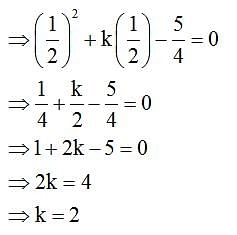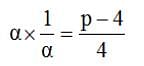Test: Quadratic Equations (Easy) - Class 10 MCQ
15 Questions MCQ Test - Test: Quadratic Equations (Easy)
Think of a positive number, say x From the square of this number, subtract three times of itself. If the result is 10, then x is equal to
Which of the following equations, is not a quadratic equation?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The roots of quadratic equation 5x2 – 4x + 5 = 0 are
Which constant should be added and subtracted to solve the quadratic equation 4x2 − √3x + 5 = 0 by the method of completing the square?
If 1 / 2 is a root of the equation x2 + kx – (5 / 4) = 0 then the value of k is
A natural number, when increased by 12, equals 160 times its reciprocal. Find the number.
The product of two successive integral multiples of 5 is 300. Then the numbers are:
If the one root of the equation 4x2 – 2x + p – 4 = 0 be the reciprocal of other. Then value of p is
Rohini had scored 10 more marks in her mathematics test out of 30 marks, 9 times these marks would have been the square of her actual marks. How many marks did she get in the test?
Equation of (x + 1)2 - x2 = 0 has number of real roots equal to: