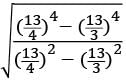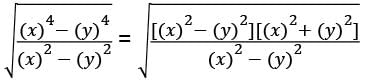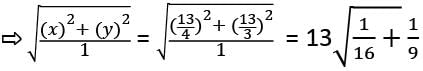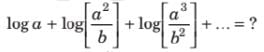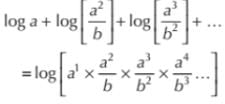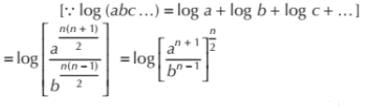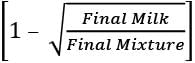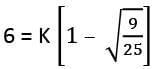Get the App Signup / Login

CAT Exam > CAT Tests > Test: SNAP Quant 2019 - CAT MCQ

Test: SNAP Quant 2019 - CAT MCQ

Test Description

30 Questions MCQ Test - Test: SNAP Quant 2019

Test: SNAP Quant 2019 for CAT 2024 is part of CAT preparation. The Test: SNAP Quant 2019 questions and answers have been prepared according to the CAT exam syllabus.The Test: SNAP Quant 2019 MCQs are made for CAT 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: SNAP Quant 2019 below.

Solutions of Test: SNAP Quant 2019 questions in English are available as part of our course for CAT & Test: SNAP Quant 2019 solutions in Hindi for CAT course. Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free. Attempt Test: SNAP Quant 2019 | 40 questions in 60 minutes | Mock test for CAT preparation | Free important questions MCQ to study for CAT Exam | Download free PDF with solutions

Test: SNAP Quant 2019 - Question 1

Save

Solve this:

Detailed Solution for Test: SNAP Quant 2019 - Question 1

Putting the values:

13 x (5/12) = 65/12

Test: SNAP Quant 2019 - Question 2

Save

What is the area of the shaded portion.

A. √2 - (π/2)
B. √3.5 – (π/2)
C. √3 - (π/2)
D. √7 - (π/2)

Detailed Solution for Test: SNAP Quant 2019 - Question 2

∆ABC is an equilateral triangle of side 2 cm.

Area of an equilateral triangle = √3/4 x a², where a is the side of that triangle.

Then, area of ∆ABC = √3/4x 2 x 2 = √3

Area of 3 sectors of angle 60° each and radius 1 cm each.

= 3 x π (radius)² × (θ/360°)

= 3 x π (1)² × (60°/360°) = π/2

Area of shaded portion = Area of ∆ABC – Area of 3 sectors

= √3 - (π/2)

1 Crore+ students have signed up on EduRev. Have you? Download the App

Test: SNAP Quant 2019 - Question 3

Save

Length of two trains is 150 m each. When they are moving in opposite directions. They cross each other in their 20s and when they are moving in the same direction. Then the faster train passed the slower train in the 40s. Then find the speed of the faster train.

A. 10.50 m/s
B. 11.25 m/s
C. 30.75 m/s
D. 25 m/s

Detailed Solution for Test: SNAP Quant 2019 - Question 3

Let speed of faster train = x m/s

Let speed of slower train = y m/s Distance = Speed × Time

Relative speed (in opposite direction) = (x + y) m/s

Relative speed (in same direction) = (x – y) m/s

In opposite direction

(x + y) = (150 + 150)/20 = 15 m/s

In same direction,

(x - y) = (150 + 150)/40 = 7.5 m/s

Using these two equations, we get x = 11.25 m/s

Test: SNAP Quant 2019 - Question 4

Save

Brigadier Rastogi travels from A to Bat 40 km/h on a bike. He travels from B to C at 10 km/h on cycle. The distance from A to Bisequal to B to C. Then he travels from C to A via B at 24 km/h by auto-rickshaw. Find his average speed.

A. 20.5 km/h
B. 21 km/h
C. 18 km/h
D. 19.2 km/h

Detailed Solution for Test: SNAP Quant 2019 - Question 4

Let distance from A to B LCM of 24, 10, 40 = 120 km

Time from A to B by bike = 120/40 = 3 h

Time from B to C by cycle = 120/10 = 12 h

All of us know that speed = Distance/time

Time from C to A by autorickshaw = 240/24 = 10 h

Average Speed = Total distance travelled/Total time taken

= (4 x 120)/(3 + 12 + 10) = 480/25 = 19.2 km/h

Test: SNAP Quant 2019 - Question 5

Save

Rohan and Rahul are 144 km apart on point A and point B respectively. Rohan travels constantly at 8 km/h. Rahul travels 4 km in the first hour, 5 km in the second hour, 6 km in the third hour and so on. Find the point where they well meet.

A. 70 km from point A
B. 74 km from point A
C. 70 km from point B
D. Exactly at midway between A and B

Detailed Solution for Test: SNAP Quant 2019 - Question 5

Speed of Rohan = 8 km/h

Speed of Rahul in start = 4 km/h and Rahul’s speed increases 1 km/h in every hour.

Relative speed in opposite direction AP series is formed = … 12, 13, 14 = Covered distance per hour

Let them meet after n hour.

According to the question, Distance between Rohan and Rahul = Covered distance in n hours Here, we will use the formula of sum of n terms of an A.P., which is:

S = n/2[2? + (? − 1)]

Where n = number of terms

a = First term of the A.P.

d = Common difference

144 = n/2[2 × 12 + (? − 1)1]

⇨ 288 = n(24 + n – 1)

Solving this equation,

n² + 23n + 288 = 0

Splitting this quadratic equation, (n – 9) (n + 32) = 0

Here, we have two values of n, which are -32 and 9, but a negative value of n is not possible in this question.

So, after 9 h Rahul and Rohan meet, then distance covered by Rohan = 9 x 8 = 72 km.

It means, they meet exactly midway between A and B.

Test: SNAP Quant 2019 - Question 6

Save

Find the unit’s digit of (1!)^1! + (2!)^2! + (3!)^3! + …….. + (100!)^100!.

A. 2
B. 5
C. 7
D. 9

Detailed Solution for Test: SNAP Quant 2019 - Question 6

5! = 5 x 4 x 3 x 2 x 1 = 120

As we know, unit digit for (5!, 6!, 7!, 8! … ∞) is zero.

Also, (1!)^1! + (2!)^2! + (3!)^3! + (4!)^4! = 1¹ + 2² + 6⁶ + 24²⁴ .. . . . .. .(1)

Unit digit for (1): 1 + 4 + 6 + 6 = 17

∴ Required unit digit = 7.

Test: SNAP Quant 2019 - Question 7

Save

In how many ways can 10 books on mechanics and 8 books on quantum physics be placed in a row such that two books on quantum physics may not be together?

A. 180
B. 170
C. 165
D. 175

Detailed Solution for Test: SNAP Quant 2019 - Question 7

Required ways = 11_?₈= (11 x 10 x 9)/(3 x 2 x 1) = 165

Test: SNAP Quant 2019 - Question 8

Save

In an institute, an MBA Exam is conducted and a sectional cut off has been introduced into it. Any candidate appearing for the exam cannot qualify unless he clears the sectional cut off. If there are 4 sections in the paper, then what is the number of ways an applicant may fail in the exam.

Detailed Solution for Test: SNAP Quant 2019 - Question 8

There are two possibilities: pass (P) and Fail (F). Then, possibility of 4 sections

Total possibility = 16

The number of ways on applicant may fail in the exam = (16 – 1) = 15

Test: SNAP Quant 2019 - Question 9

Save

In how many ways can letters of the word SOTICA be arranged such that vowels occupy odd positions?

A. 36
B. 1
C. 42
D. 21

Detailed Solution for Test: SNAP Quant 2019 - Question 9

SOTICA has three vowels. Then, the required ways = 3! x 3! = 6 x 6 = 36

Test: SNAP Quant 2019 - Question 10

Save

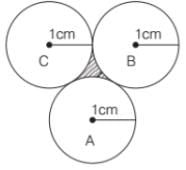
Square, Circle, Hexagon and Octagon have equal perimeter. Which has the maximum area?

A. Square
B. Circle
C. Hexagon
D. Octagon

Detailed Solution for Test: SNAP Quant 2019 - Question 10

If the perimeter of figures is the same then, the area of the figure which has the maximum number of sides is maximum. Then, the circle has a maximum area.

Test: SNAP Quant 2019 - Question 11

Save

In how many ways can one wrap 3 Kitkat, 2 Fivestar and 3 Bar one. If at least one Kitkat has to be there in the gift pack and the gift pack has three chocolates.

A. 56
B. 10
C. 46
D. 35

Detailed Solution for Test: SNAP Quant 2019 - Question 11

Number of ways of the gift pack = 8_?₃ = 56

Number of ways of the gift pack without Kitkat = 8_?₃ = 10

Then, number of ways of the gift pack with Kitkat = 56 – 10 = 46

Test: SNAP Quant 2019 - Question 12

Save

The difference between compound and simple interest for a loan is 114 when invested for 2 yr. The rate of interest is 6% per annum. Find the loan amount.

Detailed Solution for Test: SNAP Quant 2019 - Question 12

Difference between compound and simple interest for 2 years of a principal P with interest rate r% per annum = P(r/100)²

Using this formula:

114 = P(6/100)²

⇨ P = (114 x 100 x 100)/(6 x 6) = 31666.66

Test: SNAP Quant 2019 - Question 13

Save

Detailed Solution for Test: SNAP Quant 2019 - Question 13

Using the properties of Log, and simplifying this

Test: SNAP Quant 2019 - Question 14

Save

What is the coefficient of z³ in -7x y² z³a²b².

Detailed Solution for Test: SNAP Quant 2019 - Question 14

The given polynomial is -7x y² z³a²b²

If we take z³ out of this, it becomes: z³ (-7x y² z³a²b²)

Hence, (-7x y² z³a²b²) is the coefficient of z³ in the given polynomial.

Test: SNAP Quant 2019 - Question 15

Save

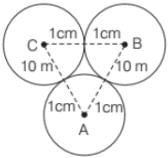
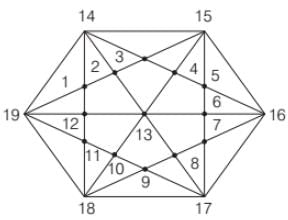
In a regular hexagon field, ropes are tied to connect all vertices (diagonal and side). What is the number of intersection points of ropes?

A. 13
B. 19
C. 15
D. 18

Detailed Solution for Test: SNAP Quant 2019 - Question 15

We can see in the above figure that there are 19 intersection points.

Test: SNAP Quant 2019 - Question 16

Save

In the given diagram, ∠ CAB = 60° and BC = a, AC = b, and AB = c.

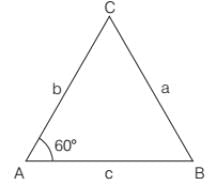
Then which of the following is correct?

A.
a² + b² + c² = abc
B.
a² = b² + c² – bc
C.
c²= b² + a² – ab
D.
b² = a² + c² = 2ac

Detailed Solution for Test: SNAP Quant 2019 - Question 16

Here, we are using “Cosine rule”, which is as follows

Cos 60° = (b² + c²– a²)/2bc

Putting the value of Cos 60°, which is 1/2 and doing the cross multiplication.

a² = b²+ c²– bc

Test: SNAP Quant 2019 - Question 17

Save

In a closed wooden box, length = 20 cm breadth = 14 cm and height = 10 cm and thickness = 5 mm. If the weight of an empty box is 3.462 kg, then what is the weight of 1 cm 3 of wood (in gms)?

Detailed Solution for Test: SNAP Quant 2019 - Question 17

Volume of cuboid = Length × Breadth × Height

Volume of wood = External volume of box with wood – Internal volume of box without wood

= (LBH)_external – (LBH)_internal

= 20 x 14 x 10 = 19 x 13 x 9

= 577 cm³

Total weight of wood = 3.462 kg

Weight of 577 cm³ of wood = 3462 gm

Then, weight of 1 cm³ of wood cm³ = 3462/577 = 6 gms

Test: SNAP Quant 2019 - Question 18

Save

Find the number of zeros at the end of (5!)^5! + (10!)^10! + (50!)^50! + (100!)^100!

A. 120
B. 60
C. 240
D. 480

Detailed Solution for Test: SNAP Quant 2019 - Question 18

(5!)^5! is added to the remaining numbers then only we have to find the number of zero in (5!)^5!

5! = 5 x 4 x 3 x 2 x 1 = 120

Now, 120¹²⁰ will have 120 zeroes.

Test: SNAP Quant 2019 - Question 19

Save

Gitesh is twice as good as Jitesh. Gitesh takes 30 days less than Jitesh to finish a task. How long will Gitesh and Jitesh take to complete the task together.

A.
20 days
B.
30 days
C.
60 days
D.
15 days

Detailed Solution for Test: SNAP Quant 2019 - Question 19

Let efficiency of Gitesh = 2x, and efficiency of Jitesh = x

Jitesh is half efficient as Gitesh, so he will take double time to finish the task.

And, the difference between their time is 30 days.

So, Jitesh’s time = 2 x 30 = 60 days

And, Gitesh’s time = x = 30 days

Let us assume the total work to be 60 units.

Jitesh will do 1 unit per day.

Gitesh will do 2 units per day.

Together they will do 3 units per day.

Time taken by both, when working together = 60/3 = 20 days.

Test: SNAP Quant 2019 - Question 20

Save

Mohan has a 200 L container. K L of milk is kept in the container. Mohan removes 6L milk and adds 6L water. He again replaces 6L of solution with water. Now milk and water are in the ratio 9: 16. What is the value of K?

Detailed Solution for Test: SNAP Quant 2019 - Question 20

Water which was added =

Initial quantity of substance x

Putting the values:

⇨ 6 = 2K/5

⇨ K = 15 litres

Test: SNAP Quant 2019 - Question 21

Save

Indian express travels at 60 km/h and halts. For fixed time every hour. Due to halts, the average speed becomes 50 km/h. Find the time of halt.

A. 10 min
B. 20 min
C. 15 min
D. 12 min

Detailed Solution for Test: SNAP Quant 2019 - Question 21

Halt time = [(Faster train’s speed – Slower train’s speed)/Faster train’s speed] x 60

[(60 – 50)/60] x 60 = 10 mins.

Test: SNAP Quant 2019 - Question 22

Save

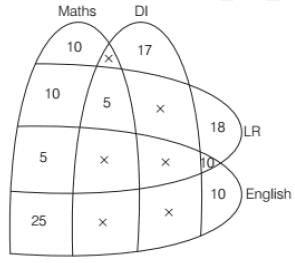
Directions: Read the given information carefully and answer the given question. In 2015, 100 aspirants appeared for an exam.

They had to answer four sections Maths, DI, LR and English.

The number of students who qualified in Maths = 55.

The number of students who qualified in LR= 38.

The number of students who qualified in (Maths + English) = 30.

The number of students who qualified in (LR + English) = 15.

The number of students who qualified in (Maths + LR) = 20.

The number of students who qualified in (Maths + LR + English) = 5.

The number of students who qualified in DI = 22.

The number of students who qualified in (DI + LR) = 5.

The number of students who qualified in (DI + Maths) = 5.

The number of students who qualified in (DI + Maths + LR) = 5.

The number of students who qualified in English = 50.

Those who qualified in English, could not qualify in the DI section.

Q. How many qualified at least two sections?

A. 60
B. 55
C. 50
D. 20

Detailed Solution for Test: SNAP Quant 2019 - Question 22

Using the Venn Diagram concepts, we can draw a diagram like this

Qualified at least two section = 10 + 5 + 5 + 25 + 10 = 55 aspirants.

Test: SNAP Quant 2019 - Question 23

Save

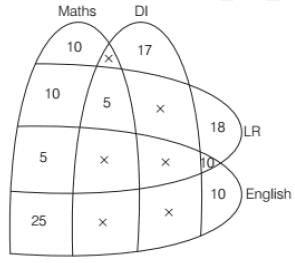
Directions: Read the given information carefully and answer the given question. In 2015, 100 aspirants appeared for an exam.

They had to answer four sections Maths, DI, LR and English.

The number of students who qualified in Maths = 55.

The number of students who qualified in LR= 38.

The number of students who qualified in (Maths + English) = 30.

The number of students who qualified in (LR + English) = 15.

The number of students who qualified in (Maths + LR) = 20.

The number of students who qualified in (Maths + LR + English) = 5.

The number of students who qualified in DI = 22.

The number of students who qualified in (DI + LR) = 5.

The number of students who qualified in (DI + Maths) = 5.

The number of students who qualified in (DI + Maths + LR) = 5.

The number of students who qualified in English = 50.

Those who qualified in English, could not qualify in the DI section.

Q. How many qualified in both Maths and LR, but not in any other section?

A. 10
B. 20
C. 25
D. 5

Detailed Solution for Test: SNAP Quant 2019 - Question 23

Using the Venn Diagram concepts, we can draw a diagram like this

Qualified in both Maths and LR = 10 aspirants.

Test: SNAP Quant 2019 - Question 24

Save

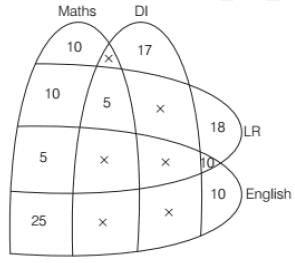
Directions: Read the given information carefully and answer the given question. In 2015, 100 aspirants appeared for an exam.

They had to answer four sections Maths, DI, LR and English.

The number of students who qualified in Maths = 55.

The number of students who qualified in LR= 38.

The number of students who qualified in (Maths + English) = 30.

The number of students who qualified in (LR + English) = 15.

The number of students who qualified in (Maths + LR) = 20.

The number of students who qualified in (Maths + LR + English) = 5.

The number of students who qualified in DI = 22.

The number of students who qualified in (DI + LR) = 5.

The number of students who qualified in (DI + Maths) = 5.

The number of students who qualified in (DI + Maths + LR) = 5.

The number of students who qualified in English = 50.

Those who qualified in English, could not qualify in the DI section.

Q. How many did not qualify any section?

A. 20
B. 10
C. 0
D. 5

Detailed Solution for Test: SNAP Quant 2019 - Question 24

Using the Venn Diagram concepts, we can draw a diagram like this

None of the students failed i.e., 0.

Test: SNAP Quant 2019 - Question 25

Save

Directions: Read the given information carefully and answer the given question. In 2015, 100 aspirants appeared for an exam.

They had to answer four sections Maths, DI, LR and English.

The number of students who qualified in Maths = 55.

The number of students who qualified in LR= 38.

The number of students who qualified in (Maths + English) = 30.

The number of students who qualified in (LR + English) = 15.

The number of students who qualified in (Maths + LR) = 20.

The number of students who qualified in (Maths + LR + English) = 5.

The number of students who qualified in DI = 22.

The number of students who qualified in (DI + LR) = 5.

The number of students who qualified in (DI + Maths) = 5.

The number of students who qualified in (DI + Maths + LR) = 5.

The number of students who qualified in English = 50.

Those who qualified in English, could not qualify in the DI section.

Q. How many qualified only DI sections?

A. 10
B. 25
C. 20
D. 17

Detailed Solution for Test: SNAP Quant 2019 - Question 25

Using the Venn Diagram concepts, we can draw a diagram like this

Qualified only in DI = 17.

Test: SNAP Quant 2019 - Question 26

Save

Directions:

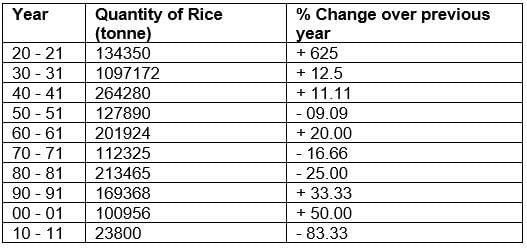
Q. What is the approximate production of rice in the year 1949-50?

A. 132650 tonne
B. 160479 tonne
C. 140679 tonne
D. 120659 tonne

Detailed Solution for Test: SNAP Quant 2019 - Question 26

Production of rice in year 1950 – 51= 127890 tonne

Production of rice in 1950-51 is 09.09% less than that of in the year 1949-50.

As we know that 09.09% is 1/11. Using it below:

Then, production of rice in 1949-50

= (11/10) x Production of rice in year 1950-51

= (11/10) x 127890 = 140679 tonne

Test: SNAP Quant 2019 - Question 27

Save

Directions:

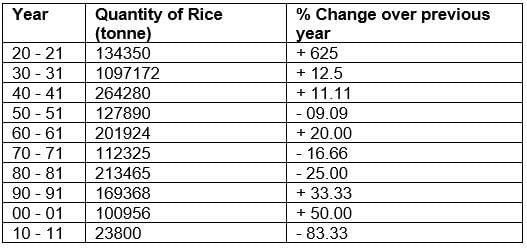
Q. What is the difference in the production of rice in 1969-70 and 1979-80?

A. 149830 tonnes
B. 125600 tonnes
C. 143980 tonnes
D. 162500 tonnes

Detailed Solution for Test: SNAP Quant 2019 - Question 27

Required difference = (Production of rice in 1979-80) − (Production of rice in 1969-70) Using the fraction values of %: = 213465 x (4/3) – 112325 x (6/5) = 284620 – 134790 = 149830 tonne.

Test: SNAP Quant 2019 - Question 28

Save

Directions:

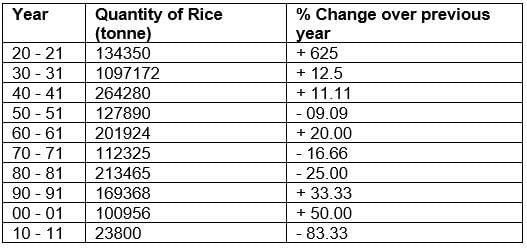
Q. What is the approximate production in 1959-60?

A. 168270 tonnes
B. 148070 tonnes
C. 157270 tonnes
D. 1382890 tonnes

Detailed Solution for Test: SNAP Quant 2019 - Question 28

Production of rice in 1960-61= 201924 tonne

The production in 1959-60:

5/6 of 201924 = 168270 tonne

Test: SNAP Quant 2019 - Question 29

Save

What is the sum of integers from 113 to 113113, which are divisible by 7?

A. 899235637
B. 867265029
C. 913952088
D. 752643214

Detailed Solution for Test: SNAP Quant 2019 - Question 29

119 + 126 + …….. + 113113

This is the series of AP.

Let the total number of terms be n.

Last number of an AP = a + (n – 1)d

Where a = first term

n = number of terms

d = common difference

Putting the values in the formula:

113113 = 119 + (n – 1) x 7

n = 16143

There is another formula, and it is for the sum of n terms of an AP when first and last terms are given.

Sum = n/2(a + l)

= 16143/2 x (119 + 113113)

= 913952088

Test: SNAP Quant 2019 - Question 30

Save

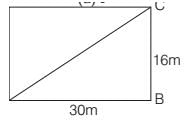
Robot is 4 m in length and placed at a corner of a 16 m × 30 m field. The robot is facing a diagonally opposite corner and reaches the diagonally opposite corner in 15 s. What is the speed (in m/s) of a robot?

A. 5
B. 2
C. 3
D. 1

Detailed Solution for Test: SNAP Quant 2019 - Question 30

ABCD is rectangle. Then, ∠B = 90°

Using the Pythagorean Theorem:

AC = √256 + √900 = √1156 = 34

Length of the Robot = 4 m.

Travelled distance by the Robot = 34 – 4 = 30 m

Speed of the Robot = 30/15 = 2 m/s

View more questions

Information about Test: SNAP Quant 2019 Page

In this test you can find the Exam questions for Test: SNAP Quant 2019 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: SNAP Quant 2019, EduRev gives you an ample number of Online tests for practice

Top Courses for CAT

Download as PDF

Top Courses for CAT

Join with a free account

Get Instant Access to 1000+ FREE Docs, Videos & Tests

10,000,000

Users

100+

Exams

3,25,000

Docs and Videos

75,000

Tests

I have an EduRev Account • Sign Up with Email

Attempt this test on App!

Get detailed analysis along with solutions of each question.

Open in App

Not Now

Schools

Medical
Non- Medical
Commerce
Humanities
CBSE Class 10
CBSE Class 9
CBSE Class 8
CBSE Class 7
CBSE Class 6
CBSE Class 5
CBSE Class 4
CBSE Class 3
CBSE Class 2
CBSE Class 1

Competitive Examinations

UPSC
NEET
JEE
CLAT
CAT
GMAT
GATE
Railways
SSC
IIT JAM
Defence
Insurance
Teaching
CA Foundation

Technical Exams

GATE
SSC JE
RRB JE
Civil Engineering
Computer Science Engineering
Electrical Engineering
Electronics Engineering
Biotechnology Engineering
Mechanical Engineering
Chemical Engineering

Important Questions for SNAP Quant 2019

Find all the important questions for SNAP Quant 2019 at EduRev.Get fully prepared for SNAP Quant 2019 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the SNAP Quant 2019 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of SNAP Quant 2019 resources.

SNAP Quant 2019 MCQs with Answers

Prepare for the SNAP Quant 2019 within the CAT exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for SNAP Quant 2019

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace SNAP Quant 2019 with ease. Join thousands of successful students who have benefited from our trusted online resources.

Education Revolution