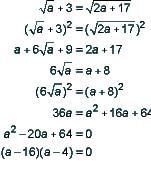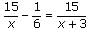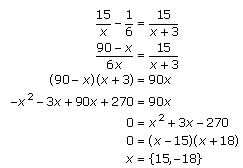Test: Word Problems - 2 - GMAT MCQ
15 Questions MCQ Test - Test: Word Problems - 2
Billy has an unlimited supply of the following coins: pennies (1¢), nickels (5¢), dimes (10¢), quarters (25¢), and half-dollars (50¢). On Monday, Billy bought one candy for less than a dollar and paid for it with exactly four coins (i.e., he received no change). On Tuesday, he bought two of the same candy and again paid with exactly four coins. On Wednesday, he bought three of the candies, on Thursday four of the candies, and on Friday five of the candies; each day he was able to pay with exactly four coins. Which of the following could be the price of one candy in cents?
A certain violet paint contains 30 percent blue pigment and 70 percent red pigment by weight. A certain green paint contains 50 percent blue pigment and 50 percent yellow pigment. When these paints are mixed to produce a brown paint, the brown paint contains 40 percent blue pigment. If the brown paint weighs 10 grams, then the red pigment contributes how many grams of that weight?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The workforce of a certain company comprised exactly 10,500 employees after a four-year period during which it increased every year. During this four-year period, the ratio of the number of workers from one year to the next was always an integer. The ratio of the number of workers after the fourth year to the number of workers after the second year is 6 to 1. The ratio of the number of workers after the third year to the number of workers after the first year is 14 to 1. The ratio of the number of workers after the third year to the number of workers before the four-year period began is 70 to 1. How many employees did the company have after the first year?
A certain farm has a group of sheep, some of which are rams (males) and the rest ewes (females). The ratio of rams to ewes on the farm is 4 to 5. The sheep are divided into three pens, each of which contains the same number of sheep. If the ratio of rams to ewes in the first pen is 4 to 11, and if the ratio of rams to ewes in the second pen is the same as that of rams to ewes in the third, which of the following is the ratio of rams to ewes in the third pen?
The price of a bushel of corn is currently $3.20, and the price of a peck of wheat is $5.80. The price of corn is increasing at a constant rate of 5x cents per day while the price of wheat is decreasing at a constant rate of (x√2 – x) cents per day. What is the approximate price when a bushel of corn costs the same amount as a peck of wheat?
A computer chip manufacturer expects the ratio of the number of defective chips to the total number of chips in all future shipments to equal the corresponding ratio for shipments S1, S2, S3 and S4 combined, as shown in the following table. What is the expected number of defective chips in a shipment of 60,000 chips?
A certain library assesses fines for overdue books as follows. On the first day that a book is overdue, the total fine is $0.10. For each additional day that the book is overdue the total fine is either increased by $0.30 or double, whichever results in the lesser amount. What is the total fine for a book on the fourth day it is
overdue?
When a certain tree was first planted, it was 4 feet tall, and the height of the tree increased by a constant amount each year for the next 6 years. At the end of the 6th year, the tree was 1/5 taller than it was at the end of 4th year. By how many feet did the height of the tree increase each year?
A certain company plans to sell Product X for p dollars per unit, where p is randomly chosen from all possible positive values not greater than 100. The monthly manufacturing cost for Product X (in thousands of dollars) is 12 - p, and the projected monthly revenue from Product X (in thousands of dollars) is p(6 - p). If the projected revenue is realized, what is the probability that the company will NOT see a profit on sales of Product X in the first month of sales?
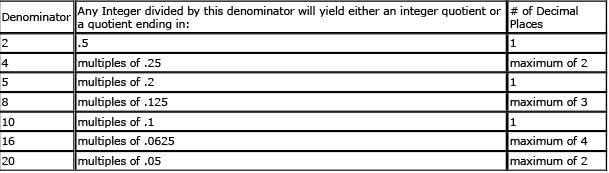
Every day a certain bank calculates its average daily deposit for that calendar month up to and including that day. If on a randomly chosen day in June the sum of all deposits up to and including that day is a prime integer greater than 100, what is the probability that the average daily deposit up to and including that day contains fewer than 5 decimal places?
A certain cube is composed of 1000 smaller cubes, arranged 10 by 10 by 10. The top layer of cubes is removed from a face, then from the adjacent face above it, then from the adjacent face to the right of the first. The process is repeated on the same three faces in reverse order. Finally, a last layer is taken from the first face. How many smaller cubes have been removed from the larger cube?
The vertical position of an object can be approximated at any given time by the function: p(t) = rt – 5t 2 + b, where p(t) is the vertical position in meters, t is the time in seconds, and r and b are constants. After 2 seconds, the position of an object is 41 meters, and after 5 seconds the position is 26 meters. What is the position of the object, in meters, after 4 seconds?
A Trussian's weight, in keils, can be calculated by taking the square root of his age in years. A Trussian teenager now weighs three keils less than he will seventeen years after he is twice as old as he is now. How old is he now?
Jim went to the bakery to buy donuts for his office mates. He chose a quantity of similar donuts, for which he was charged a total of $15. As the donuts were being boxed, Jim noticed that a few of them were slightly ragged-looking so he complained to the clerk. The clerk immediately apologized and then gave Jim 3 extra donuts for free to make up for the damaged goods. As Jim left the shop, he realized that due to the addition of the 3 free donuts, the effective price of the donuts was reduced by $2 per dozen. How many donuts did Jim receive in the end?
Roberto has three children: two girls and a boy. All were born on the same date in different years. The sum of the ages of the two girls today is smaller than the age of the boy today, but a year from now the sum of the ages of the girls will equal the age of the boy. Three years from today, the difference between the age of the boy and the combined ages of the girls will be









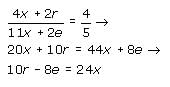
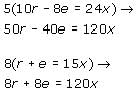
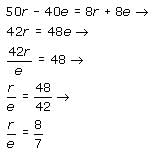
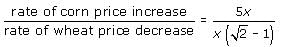
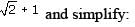
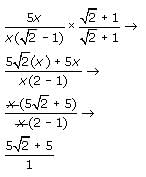
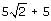 cents. So if the price of wheat decreases by x cents, the price of corn will increase by
cents. So if the price of wheat decreases by x cents, the price of corn will increase by 
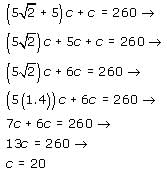
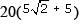 cents when it reached the same level as the decreased price of a peck of wheat. This is equivalent to an increase of approximately 240 cents. Thus the increased price of a bushel of corn = $3.20 + $2.40 = $5.60.)
cents when it reached the same level as the decreased price of a peck of wheat. This is equivalent to an increase of approximately 240 cents. Thus the increased price of a bushel of corn = $3.20 + $2.40 = $5.60.)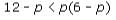
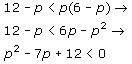
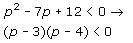
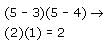
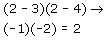
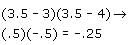
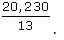

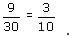
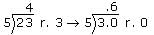
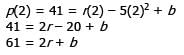
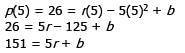
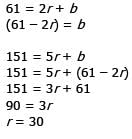
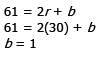
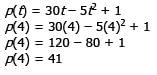
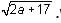 We are told that the Trussian's current weight, √a is three keils less than his future weight,
We are told that the Trussian's current weight, √a is three keils less than his future weight, 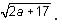 Therefore,
Therefore, 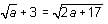 We can solve the equation as follows:
We can solve the equation as follows: