All Courses
Get the App Signup / Login
Sign in Open App
Class 9 Exam  >  Class 9 Tests  >  Mathematics (Maths) Class 9  >  Test: Congruence Criteria- SSS And RHS - Class 9 MCQ

Test: Congruence Criteria- SSS And RHS - Class 9 MCQ


Test Description

10 Questions MCQ Test Mathematics (Maths) Class 9 - Test: Congruence Criteria- SSS And RHS

Test: Congruence Criteria- SSS And RHS for Class 9 2024 is part of Mathematics (Maths) Class 9 preparation. The Test: Congruence Criteria- SSS And RHS questions and answers have been prepared according to the Class 9 exam syllabus.The Test: Congruence Criteria- SSS And RHS MCQs are made for Class 9 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Congruence Criteria- SSS And RHS below.
Solutions of Test: Congruence Criteria- SSS And RHS questions in English are available as part of our Mathematics (Maths) Class 9 for Class 9 & Test: Congruence Criteria- SSS And RHS solutions in Hindi for Mathematics (Maths) Class 9 course. Download more important topics, notes, lectures and mock test series for Class 9 Exam by signing up for free. Attempt Test: Congruence Criteria- SSS And RHS | 10 questions in 10 minutes | Mock test for Class 9 preparation | Free important questions MCQ to study Mathematics (Maths) Class 9 for Class 9 Exam | Download free PDF with solutions
Test: Congruence Criteria- SSS And RHS - Question 1
Save

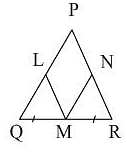
In isosceles ΔPQR, PQ = PR, M is the mid point of QR. LM ⊥ PQ, MN ⊥ PR. By which criterion of congruency is ΔQLM 0 ≅ ΔMNR.

Detailed Solution for Test: Congruence Criteria- SSS And RHS - Question 1

∠LQ = ∠MNR,

∠Q = ∠R

QM = MR,

Hence, ΔQLM ≅ ΔMNR (by AAS)

Test: Congruence Criteria- SSS And RHS - Question 2
Save

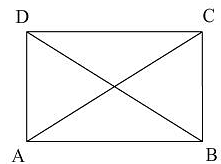
ABCD is a parallelogram, if the two diagonals are equal, then by what criterion are the triangles ABD and ABC congruent

Detailed Solution for Test: Congruence Criteria- SSS And RHS - Question 2

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Congruence Criteria- SSS And RHS - Question 3
Save

In ΔABC and ΔPBC, AB = BP and AC = PC. Can you say whether the triangles are congruent to each other or not:

Test: Congruence Criteria- SSS And RHS - Question 4
Save

In the given figure, AB = PQ, BC = QR and the median AD is equal to the median PM of the other triangle PQR, then ΔABD is congruent  ΔPQM by the criterion
Test: Congruence Criteria- SSS And RHS - Question 5
Save

Two equilateral triangles are congruent when:
Detailed Solution for Test: Congruence Criteria- SSS And RHS - Question 5

Explanation: For two equilateral triangles to be congruent, their corresponding sides must be equal in length. In congruent triangles, all corresponding sides and angles are identical. While equilateral triangles always have equal angles (60°), congruence is specifically determined by the equality of sides.

Test: Congruence Criteria- SSS And RHS - Question 6
Save

In the following figure, if PQR ≅ ABC, then
Test: Congruence Criteria- SSS And RHS - Question 7
Save

Choose the correct statement
Test: Congruence Criteria- SSS And RHS - Question 8
Save

In ΔABC and PQR, AB = QR, BC = RP, AC = QP. So,
Detailed Solution for Test: Congruence Criteria- SSS And RHS - Question 8
Option b is correct because when we write the names of triangle we write according to the corresponding parts of one triangle to otherfor eg. in ∆abc and ∆qrp we have ab = qr which also corresponds to the corresponding parts of congurent triangles property
Test: Congruence Criteria- SSS And RHS - Question 9
Save

In the following figure, PT is the bisector of ___________.
Detailed Solution for Test: Congruence Criteria- SSS And RHS - Question 9

as QT = TR and QP = PR
then  QT/QP = TR/PR
so PT would be internal bisector of ∠QPR

Test: Congruence Criteria- SSS And RHS - Question 10
Save

PQRS is a parallelogram, if the two diagonals are equal, then the measure of PQR is:
44 videos|412 docs|55 tests
Join Course
Information about Test: Congruence Criteria- SSS And RHS Page
In this test you can find the Exam questions for Test: Congruence Criteria- SSS And RHS solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Congruence Criteria- SSS And RHS , EduRev gives you an ample number of Online tests for practice

Top Courses for Class 9

44 videos|412 docs|55 tests
Join Course
Download as PDF

Top Courses for Class 9

Important Questions for Congruence Criteria- SSS And RHS

Find all the important questions for Congruence Criteria- SSS And RHS at EduRev.Get fully prepared for Congruence Criteria- SSS And RHS with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Congruence Criteria- SSS And RHS syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Congruence Criteria- SSS And RHS resources.

Congruence Criteria- SSS And RHS MCQs with Answers

Prepare for the Congruence Criteria- SSS And RHS within the Class 9 exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Congruence Criteria- SSS And RHS Mathematics (Maths) Class 9

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Congruence Criteria- SSS And RHS with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup