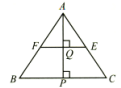Test: Quadrilaterals- Assertion & Reason Type Questions - Class 9 MCQ
10 Questions MCQ Test Class 9: Additional Practice - Test: Quadrilaterals- Assertion & Reason Type Questions
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
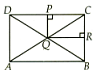
Assertion : ABCD and PQRC are rectangles and Q is a midpoint of AC . Then DP = PC.
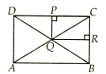
Reason : The line segment joining the midpoint of any two sides of a triangle is parallel to the third side and equal to half of it.

Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : Two opposite angles of a parallelogram are (3x - 2º) and (50 - x)º . The measure of one of the angle is 37º.
Reason : Opposite angles of a parallelogram are equal.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : In ΔABC , E and F are the midpoints of AC and AB respectively. The altitude AP at BC intersects FE at Q. Then, AQ QP = .
Reason : Q is the midpoint of AP.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : The angles of a quadrilateral are xº, (x - 10)º , (x + 30)º and (2x)º , the smallest angle is equal to 58º.
Reason : Sum of the angles of a quadrilateral is 360º.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : A parallelogram consists of two congruent triangles.
Reason : Diagonal of a parallelogram divides it into two congruent triangles.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : If the diagonals of a parallelogram ABCD are equal, then ∠ABC = 90º.
Reason : If the diagonals of a parallelogram are equal, it becomes a rectangle.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : If the angles of a quadrilateral are in the ratio 2 : 3 : 7 : 6, then the measure of angles are 40º, 60º, 140º, 120º, respectively.
Reason : The sum of the angles of a quadrilateral is 360º.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
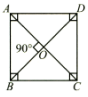
Assertion : ABCD is a square. AC and BD intersect at O. The measure of ∠AOB = 90º.
Reason : Diagonals of a square bisect each other at right angles.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
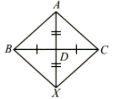
Assertion : In ΔABC , median AD is produced to X such that AD DX = . Then ABXC is a parallelogram.
Reason : Diagonals AX and BC bisect each other at right angles.
Direction: In each of the following questions, a statement of Assertion is given followed by a corresponding statement of Reason just below it. Of the statements, mark the correct.
Assertion : The consecutive sides of a quadrilateral have one common point.
Reason : The opposite sides of a quadrilateral have two common point.
|
4 docs|108 tests
|