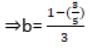Test: Pair of Linear Equations in Two Variables (Hard) - Class 10 MCQ
20 Questions MCQ Test - Test: Pair of Linear Equations in Two Variables (Hard)
Solve the following equations by the substitution method 0.04x + 0.02y = 5, 0.5x - 0.4y = 30
A house has a rectangular yard in front of it for children to play. The length of that rectangle exceeds its width by 6 m and its perimeter is 60 m.find the measurement of the yar(d)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Find The value of x and y using cross multiplication method: 4x + 3y = 8 and 4x + 5y = 12
If the lines 3x + 2ky – 2 = 0 and 2x + 5y + 1 = 0 are parallel, then what is the value of k?
If one equation of a pair of dependent linear equations is -3x + 5y - 2 = 0. The second equation will be:
The solution of the equations x - y = 2 and x + y = 4 is:
A fraction becomes 1/3 when 1 is subtracted from the numerator and it becomes 1/4 when 8 is added to its denominator. The fraction obtained is:
The solution of 4/x+3y=14 and 3/x-4y=23 is:
Ritu can row downstream 20 km in 2 hours, and upstream 4 km in 2 hours. Her speed of rowing in still water and the speed of the current is:
The angles of cyclic quadrilaterals ABCD are: A = (6x + 10), B = (5x)°, C = (x + y)° and D = (3y - 10)°. The value of x and y is:
Half the perimeter of a rectangular room is 46 m, and its length is 6 m more than its breadth. What is the length and breadth of the room?
Solve the following pair of equations: 2x + y = 73x + 2y = 12 Choose the correct answer from the given options.
Choose the pair of equations which satisfy the point (1,-1)
54 is divided into two parts such that sum of 10 times the first part and 22 times the second part is 780. What is the bigger part?
What are the values of a, b and c for the equation y = 0.5x + √7 when written in the standard form: ax + by + c = 0?
Which of these points lie on the line 7x+8y=61
Which of these following equations have x = −3, y = 2 as solutions?
If y = 1 / 2 (3x + 7) is rewritten in the form ax + by + c = 0, what are the values of a, b and c?
Solve the following pair of equations:
(1 / x) + (3 / y) = 1
(6 / x) - 12 / y = 2