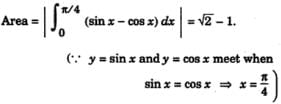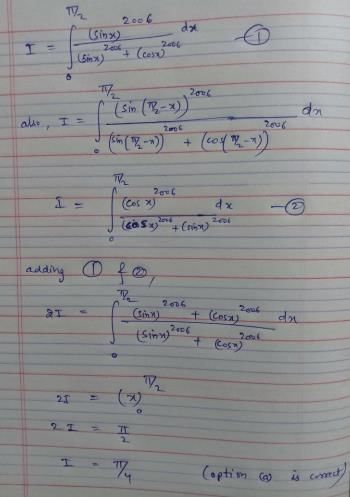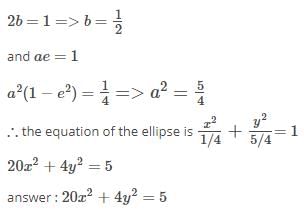JEE Main Practice Test- 16 - JEE MCQ
30 Questions MCQ Test - JEE Main Practice Test- 16
If a, b, c are in H.P., then straight line x/a + y/b + 1/c = 0 always passes thro' a fixed point and that point is
The angle between the line (x - 2)/2 = (y + 1)/-1 = (z - 3)/2 and the plane 3x + 6y - 2z + 5 = 0 is
The area formed by triangular shaped region bounded by the curves y = sinx, y = cos x and x = 0 is
The coordinates of the pole of the line lx+my+n=0 with respect to the circle x2+y2=1 are
The complex numbers z₁,z₂,z₃ satisfying (z₁-z₃)/(z₂-z₃) = 1-(i√3/2) then triangle is
The foci of an ellipse are and minor axis is of unit length. Then the equation of the ellipse is
Which statement is logically equivalent to "If Yoda cannot use a lightsaber, then he cannot help Luke win the battle."
In the following question, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the Statements carefully and mark the correct answer-
Assertion(A): If
Reason(R) : When
In the following question, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the Statements carefully and mark the correct answer-
Assertion(A):
Reason (R): If
In the following questions, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the statements carefully and mark the correct answer -
Assertion (A): The sum of the series
Reason (R): The numbers A1, A2, ....., An are said to be the n arithmetic means between two given numbers a and b if a, A1, A2, ...., An, b are in A.P.
If A and B are two matrices such that A + B and AB are both defined, then
In the following question, a Statement-1 is given followed by a corresponding Statement-2 just below it. Read the statements carefully and mark the correct answer-
Let the vectors represent the sides of a regular hexagon.
Statement-1:
.
Statement-2:
The parabola y2=4x passes thro' the point (2,-6), then the length of its latus rectum is
In a Binomial distribution, the probability of getting a success is 1/4 and standard deviation is 3. Then its mean is
The probability distribution of a random variable X is given below. Then its mean is
X = xi 1 2 3