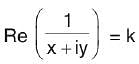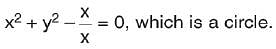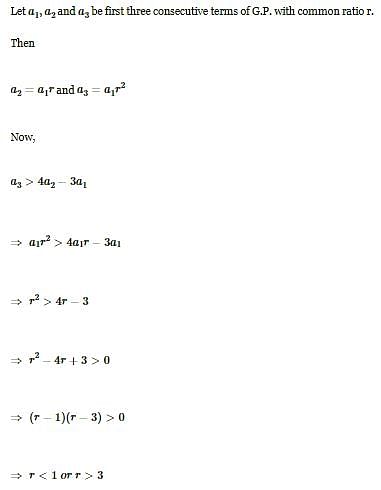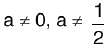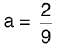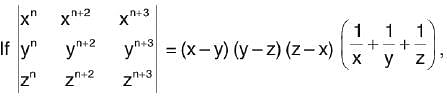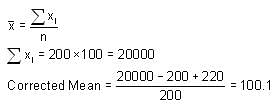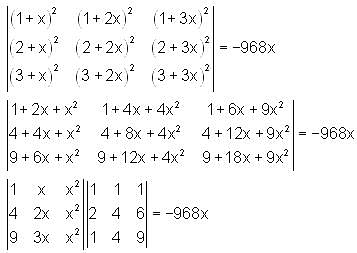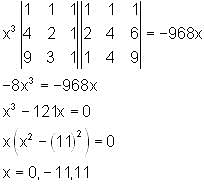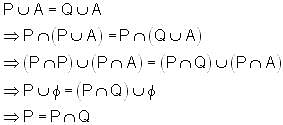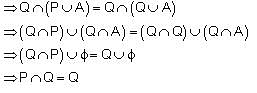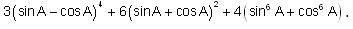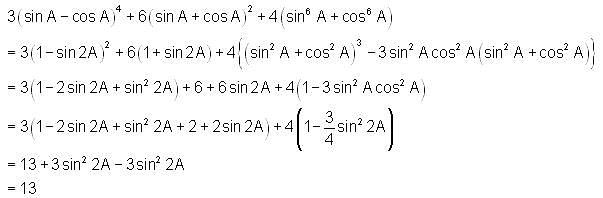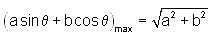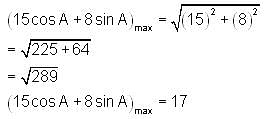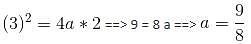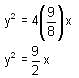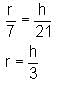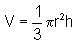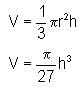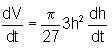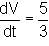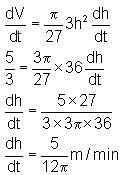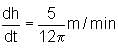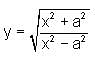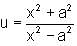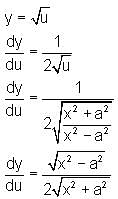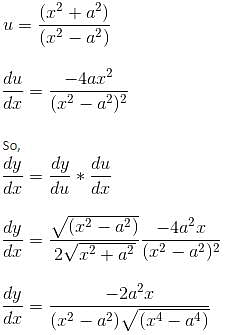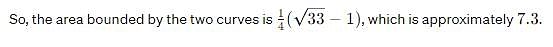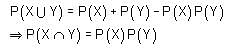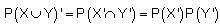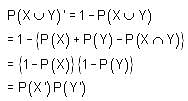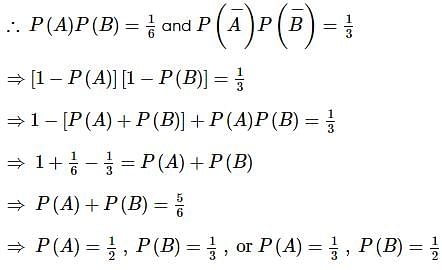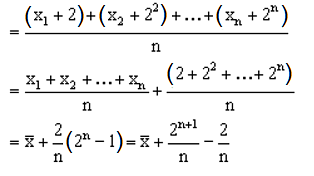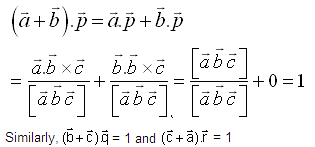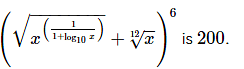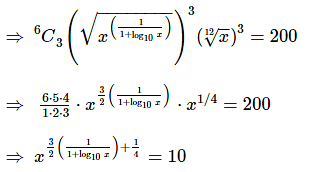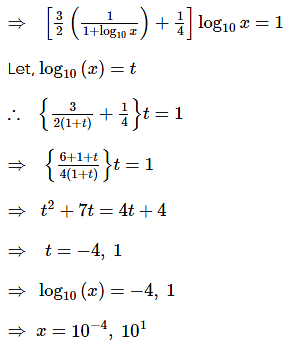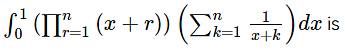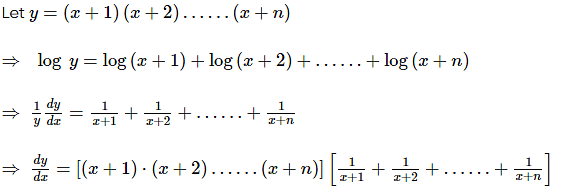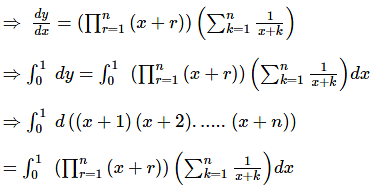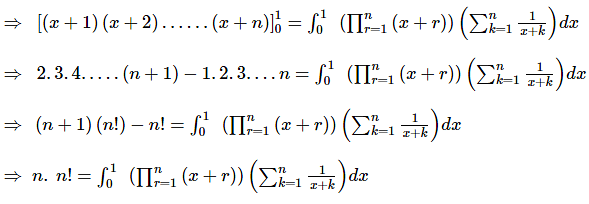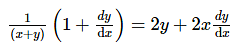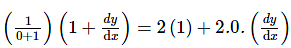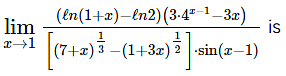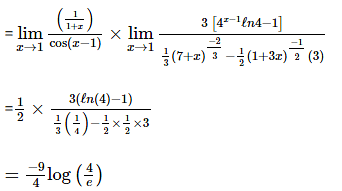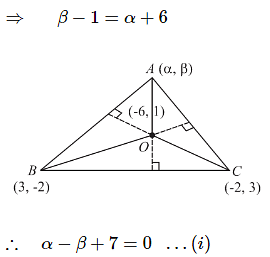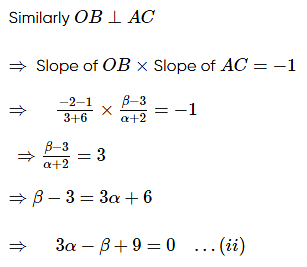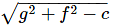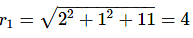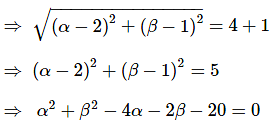BITSAT Mathematics Test - 2 - JEE MCQ
30 Questions MCQ Test BITSAT Mock Tests Series & Past Year Papers 2025 - BITSAT Mathematics Test - 2
The number of surjections from A = {1,2, ...n), n > 2 onto B = (a,b) is
The number of bijective functions from set A to itself when A contains 106 elements is
The locus of point z satisfying Re 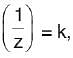 when k is a non-real real number is
when k is a non-real real number is
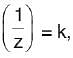 when k is a non-real real number is
when k is a non-real real number isIf a1 a2, a3 are in G.P. with common ratio r, then value of a3 > 4a2 - 3a1 holds if
If a∈ z, ( x - a ) (x - 10) + 1 = 0 has integral roots, then values of a are
The value of a for which (1 - 2a) x2 - 6ax - 1 = 0 and ax2 -x + 1 = 0 have atleast one root, in common are
The number of ways in which one or more balls can be selected out of 10 white, 9 green and 7 blues balls, is
If sum of coefficient of (a + b)n is 4096, then greatest coefficient is
A person mistakenly calculated the mean and the median of a sample data of 200 items as 100 and 104, respectively. The maximum value of the individual data was 200. When the data was rechecked, it was found that the value of the maximum sample data was 220. The values of true mean and true median are
Consider the following expression:
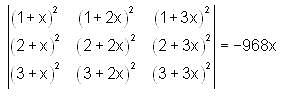
The number of values of x which satisfy the given expression is
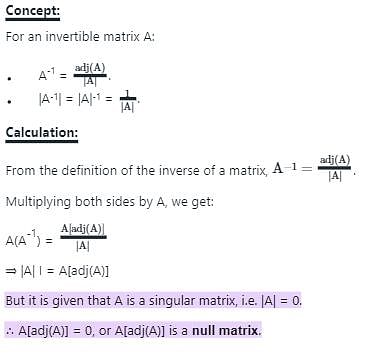
If P and Q are two sets such that 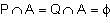 and
and 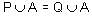 for same set A, then which of the following options is correct?
for same set A, then which of the following options is correct?
The axis of a parabola is along the abscissa. Its vertex is at origin and it passes through a point P(2, 3). The equation of the parabola is
A canonical plastic bottle whose height is 21 m and radius of base is 7 m is being filled with milk at a uniform rate of 5/3 m3/min. When the milk level is 6 m, find the rate at which the level of the milk in the bottle is rising.
Consider the given expression:
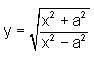
Differentiate y with respect to x.
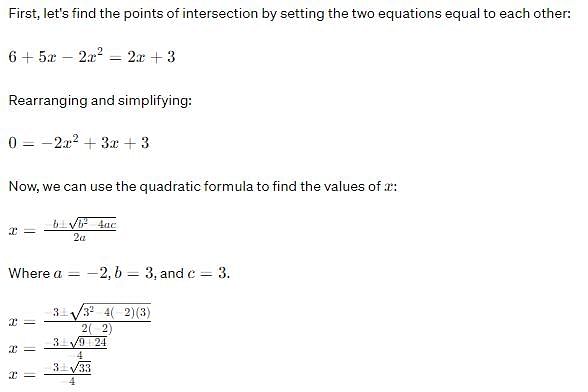
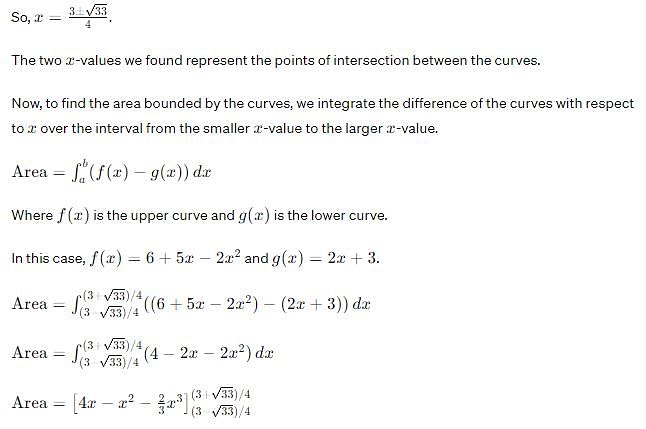
Find the area (unit2) bounded by the two curves y = 6 + 5x - 2x2 y = 2x + 3.
If 0 < P(X) < 1, 0 < P(Y) < 1 and  , then Which of the following is correct?
, then Which of the following is correct?
If A and B are independent events of a random experiments such that
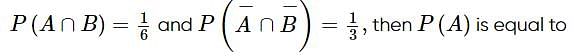
The mean of n items is  If these n items are successively increased by 2, 22, 23, …, 2n, then the new mean is
If these n items are successively increased by 2, 22, 23, …, 2n, then the new mean is
If 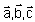 are three non-coplanar vectors and
are three non-coplanar vectors and 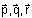 are vectors defined by the relations
are vectors defined by the relations 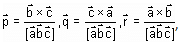 then the value of
then the value of 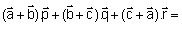
The fourth term of 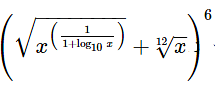 equal to 200, then the value of x satisfying this is
equal to 200, then the value of x satisfying this is
Two vertices of a triangle are (3,−2) and (−2, 3) and its orthocentre is (−6, 1). The coordinates of its third vertex are-
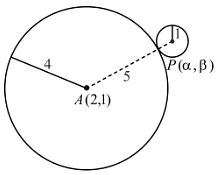
The equation of a circle C1 is x2 + y2 − 4x − 2y − 11 = 0. Another circle C2 of radius 1 unit rolls on the outer surface of the circle C1. Then the equation of the locus of the centre of C2 is
The number of real values of k for which the lines 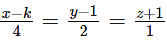 and
and 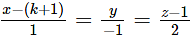 are intersecting is
are intersecting is
|
2 videos|17 docs|85 tests
|
|
2 videos|17 docs|85 tests
|